filmov
tv
Trick for doing trigonometry mentally!

Показать описание
This fast math trick can be used to mentally work out the main basic trigonometric ratios instantly! With this fast mental math technique you can work out the sin, the cos, and even the tan of the most common angles in no time at all! Become the trigonometry master!
Trick for doing trigonometry mentally!
Trick for doing Trigonometry mentally!
Exact Trig Values - Hand Trick | Trigonometry | Maths | FuseSchool
Exact Trigonometric Values using Hand Trick
Trigonometry Hand tricks ||Tricks for doing trigonometry mentally ||Remembering trigonometry values
TRICK | DOING TRIGONOMETRY MENTALLY
Cosine trick for doing trigonometry mentally #shorts
Sine Trick for doing trigonometry mentally
Trick for doing trigonometry mentally
Trick for doing trigonometry mentally | Trigonometric Ratios !
Trick to Remember Trigonometric Values | Hand trick Trigonometry | Do Trigonometry Mentally!
A Simple Trick To Remember Trigonometry Values
Trick For Doing Trigonometry Mentally. Useful for every student above 10 level.
The Hardest Math Test
Math Tricks & Tips: Trick on How to do Trigonometry mentally in seconds!
trigonometry mentally trick
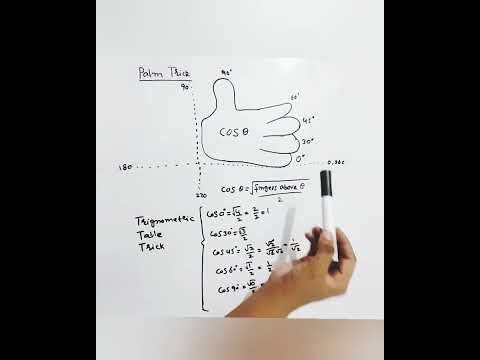
Trick for doing trigonometry mentally!Palm Trick for Cosine #mathconcepts #conceptual #learning
Tricks for doing trigonometry mentally!
Sin trigonometric values by using Hand trick II Mendakuduru #shorts #maths #trigonometry
Trigonometry - Using the left hand trick to find trig values
Trigonometry trick🎡 #sine #cos #tan #mathtrick
Cos Trigonometric values by using Hand trick II Mendakuduru #shorts #maths #trigonometry
trigonometry tricks | trigonometry tricks for competitive exams #shorts #trigonometry #tricks
Trick for doing trigonometry mentally- Easy tricks increase views in YouTube
Комментарии
 0:05:02
0:05:02
 0:02:56
0:02:56
 0:04:08
0:04:08
 0:05:38
0:05:38
 0:00:23
0:00:23
 0:08:26
0:08:26
 0:00:29
0:00:29
 0:01:11
0:01:11
 0:10:02
0:10:02
 0:07:22
0:07:22
 0:11:16
0:11:16
 0:11:10
0:11:10
 0:04:43
0:04:43
 0:00:28
0:00:28
 0:00:41
0:00:41
 0:00:47
0:00:47
 0:00:38
0:00:38
 0:05:02
0:05:02
 0:00:32
0:00:32
 0:12:33
0:12:33
 0:00:25
0:00:25
 0:00:32
0:00:32
 0:00:15
0:00:15
 0:08:06
0:08:06