Siddhartha Mishra: Deep Learning and Computations of high-dimensional PDEs | IACS Seminar

Speaker: Siddhartha Mishra, Professor for Applied Mathematics at ETH-Zurich

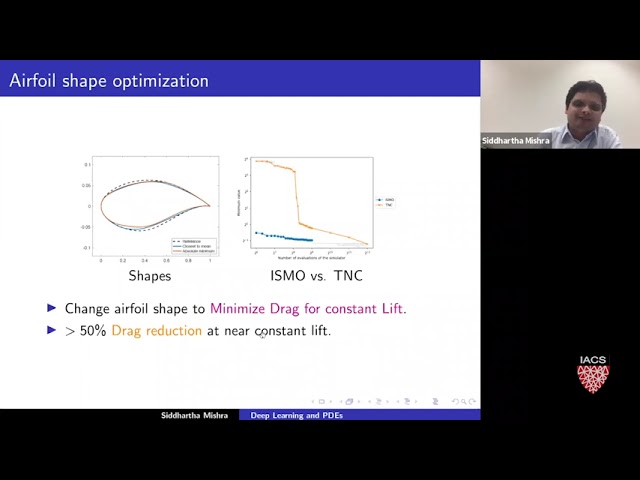
Partial Differential Equations (PDEs) with very high-dimensional state and/or parameter spaces arise in a wide variety of contexts ranging from computational chemistry and finance to many-query problems in various areas of science and engineering. In this talk, we will survey recent results on the use of deep neural networks in computing these high-dimensional PDEs. We will focus on two different aspects i.e., the use of supervised deep learning, in the form of both standard deep neural networks as well as recently proposed DeepOnets, for efficient approximation of many-query PDEs and the use of physics informed neural-networks (PINNs) for the computation of forward and inverse problems for PDEs with very high-dimensional state spaces.