QIP2021 | Entangleability of cones (Ludovico Lami)

Authors: Guillaume Aubrun, Ludovico Lami, Carlos Palazuelos and Martin Plávala
Affiliations: Université Lyon 1 | Ulm University | Departamento de Análisis Matemático y Matemática Aplicada, Universidad Complutense de Madrid | University of Siegen

Abstract
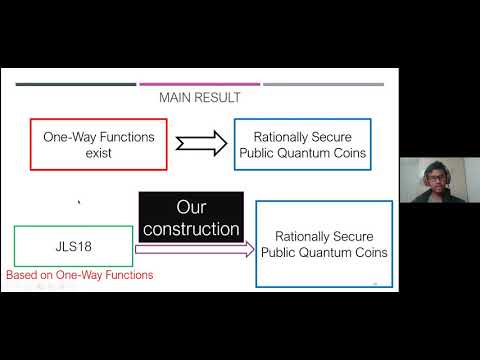
We prove that two non-classical general probabilistic theories must give rise to entanglement, either at the level of states or at the level of measurements, when combined. This reveals a deep connection between a local phenomenon (non-classicality, or the existence of superpositions) and a global one (entanglement), and raises the latter to a generically non-classical rather than merely quantum phenomenon, in a precise mathematical sense. Instrumental in our proof is the solution of a long-standing conjecture by Barker.

Get entangled with us!