Differential Equations, Lecture 6.6: Boundary value problems

Differential Equations, Lecture 6.6: Boundary value problems.

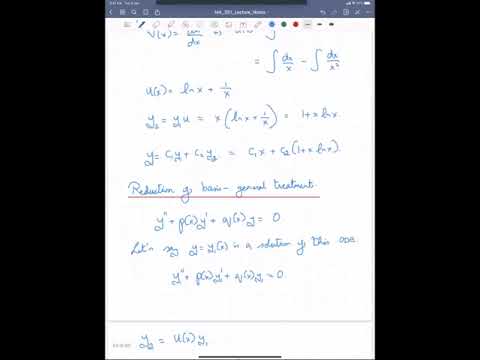
An initial value problem (IVP) is an ODE involving a function y(t) of time, with initial conditions. A boundary value problem (BVP) is an ODE involving a function y(x) of position, with boundary conditions. An example might be where y(x) measures the temperature of a rod, and the temperature at the endpoints is fixed. The theory of IVPs (existence and uniqueness of solutions) is well-understood, whereas BVPs are more mysterious. We give an 3 examples of an ODE with slightly different boundary conditions, yielding infinitely many, one, and no solutions, respectively. We conclude by solving a few variants of a classic BVP that will be very useful in our study of PDEs. In doing so, we revisit the hyperbolic trig functions: cosh and sinh.

[kilobyte]

which textbook can you get this boundary value problems

[salaldin]

Hi, thanks for this amazing video. there is a typo on minute 34:00. y'(pi)=BWcoshWpi in the video you used Cos not Cosh. hope this is clear.

I have questions:
so basically when you are using the solutions:
1- lamba=0.
2- lamda = positive.
3- lamda = negative.
when you use lamda is positive we can always use cosh and sinh for the general solution and when you solve for lamda is negative we can always use sin and cos for the general solution is that correct?

the other question is that we still can use sin and cos or exponential function instead, using the relationship that you mentioned in the beginning of the video and we will get the same answer right?

[dehoog_guitar]

This video assumes you know a lot of things already, leaves you with many questions on conclusions he reaches without explanation

[Fridaydude]

Good day.

Consider a 1st order Differential equation. Is it valid to use Neumann boundary conditions on this one, or should one strictly stick with Dirichlet ones?