Mixing Problem Differential Equation (Application)

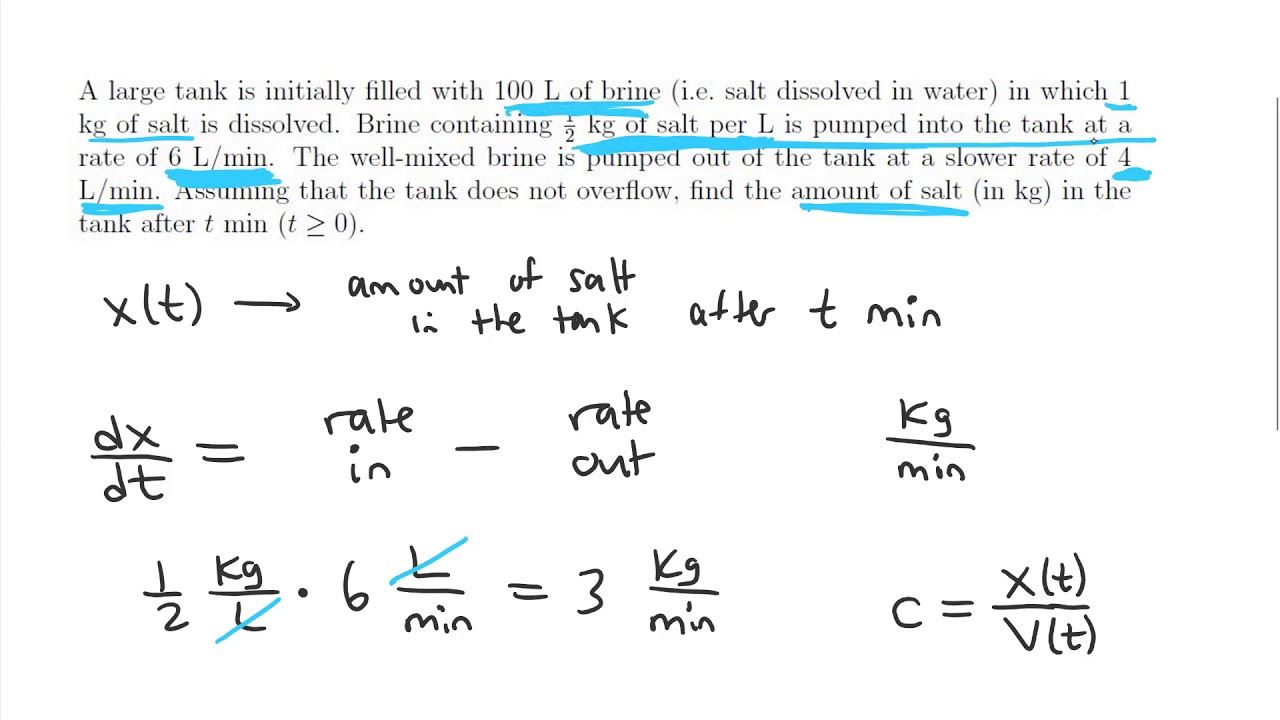
A large tank is initially filled with 100 L of brine (i.e. salt dissolved in water) in which 1 kg of salt is dissolved. Brine containing 1/2 kg of salt per L is pumped into the tank at a rate of 6 L/min. The well-mixed brine is pumped out of the tank at a slower rate of 4 L/min. Assuming that the tank does not overflow, find the amount of salt (in kg) in the
tank after t min (t ＞= 0).

Looking for help with math? I can help you!
~ For more quick examples, check out the other videos on my youtube channel

[maxpercer]

This problem is done accurately, unlike many examples online where they state the concentration of salt water as number of kilograms of salt per liter of pure water - the volume of water will increase if you add kilograms of salt

[theelk]

I worked this one out using arbitrary constants and it’s so complicated

[axianaxmerone]

Why do you. have 2t next to 100, i don't understand where that number come from?

[aimanjamil]

7:17 why the integration of 3(50+t)^2 becomes = (50+t)^3 + C instead of = 7500x+150x^2+x^3+C

[amroalatasi]

As t approaches infinite, does the solution in the tank approach the concentration of the liquid going in? If yes, why does the equation point that as t approaches infinite the mass of salt in the container approaches infinite? How is this possible? Thank you in advance

[hamzaiqbal]

Im sorry but i belive this could have been done easily but u have made it more complex

[JayEulisesAErong]

yung (50 + t)^2x'+2(50 + t)x paano po ba sya naging ((50 + t)^2x)'