filmov
tv
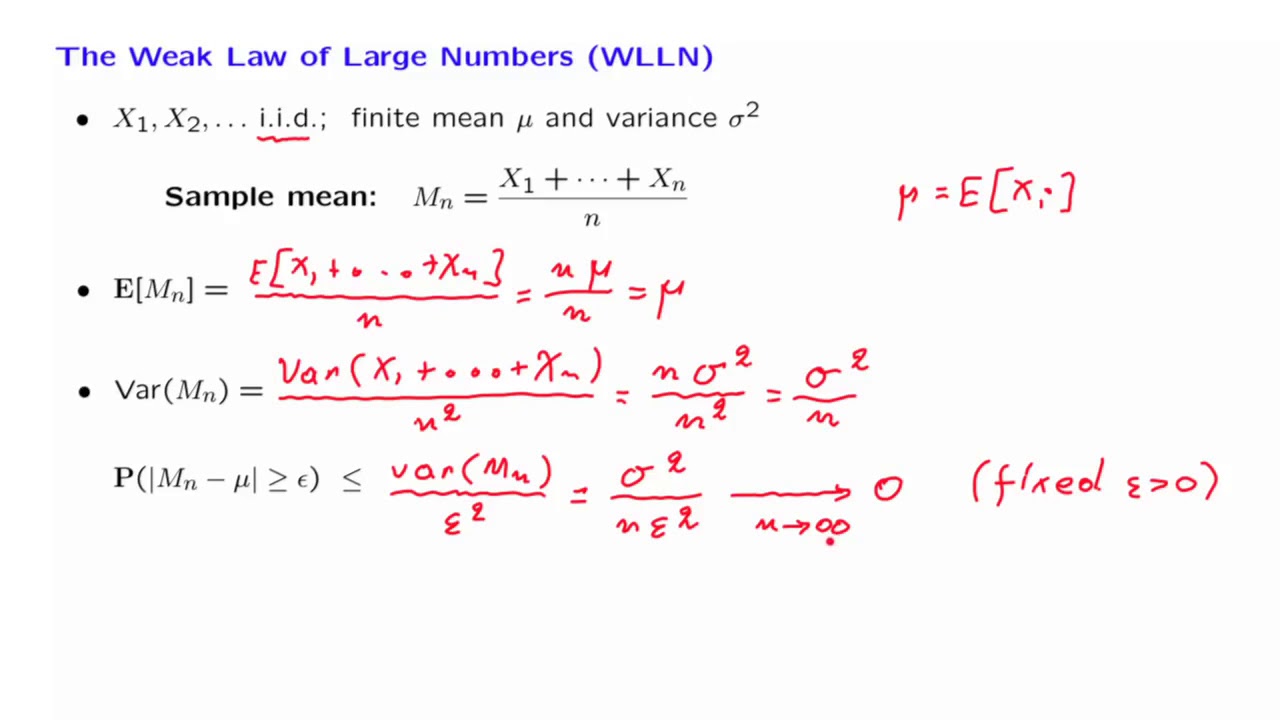
L18.4 The Weak Law of Large Numbers
Показать описание
MIT RES.6-012 Introduction to Probability, Spring 2018
Instructor: John Tsitsiklis
License: Creative Commons BY-NC-SA
Instructor: John Tsitsiklis
License: Creative Commons BY-NC-SA
L18.4 The Weak Law of Large Numbers
A proof of the weak law of large numbers
The weak law of large numbers proof using characteristic functions - part 1
Weak Law of Large Numbers (WLLNs) and Examples
The Law of Large Numbers - Explained
The Weak Law of Large Numbers
Lecture 18 (04/21) Weak law of large numbers
The weak law of large numbers
PB63: Weak Law of Large Numbers vs. Central Limit Theorem
L18.1 Lecture Overview
12.2 The Weak Law of Large Numbers (WLLN)
The Law of Large Numbers
L18.8 Related Topics
L18.6 Convergence in Probability
Probability Theory 110 The Weak Law of Large Numbers
The Weak Law of Large Numbers (Proof)
Law of Large Numbers
L18.5 Polling
The Square Root Law
Most Useless Degree? #shorts
Girls: Born Rich or Intelligent
mod11lec40 - Weak Law of Large Numbers
Strong vs Weak Law of Large Numbers
The Weak Law of Large Numbers (WLLN)
Комментарии
 0:07:31
0:07:31
 0:04:04
0:04:04
 0:09:25
0:09:25
 0:14:30
0:14:30
 0:06:32
0:06:32
 0:05:50
0:05:50
 0:52:12
0:52:12
 0:16:24
0:16:24
 0:05:53
0:05:53
 0:01:57
0:01:57
 0:22:47
0:22:47
 0:03:38
0:03:38
 0:06:44
0:06:44
 0:08:28
0:08:28
 0:12:21
0:12:21
 0:09:49
0:09:49
 0:06:14
0:06:14
 0:08:12
0:08:12
 0:00:25
0:00:25
 0:00:19
0:00:19
 0:00:24
0:00:24
 0:37:32
0:37:32
 0:04:51
0:04:51
 0:13:11
0:13:11