filmov
tv
A proof of the weak law of large numbers
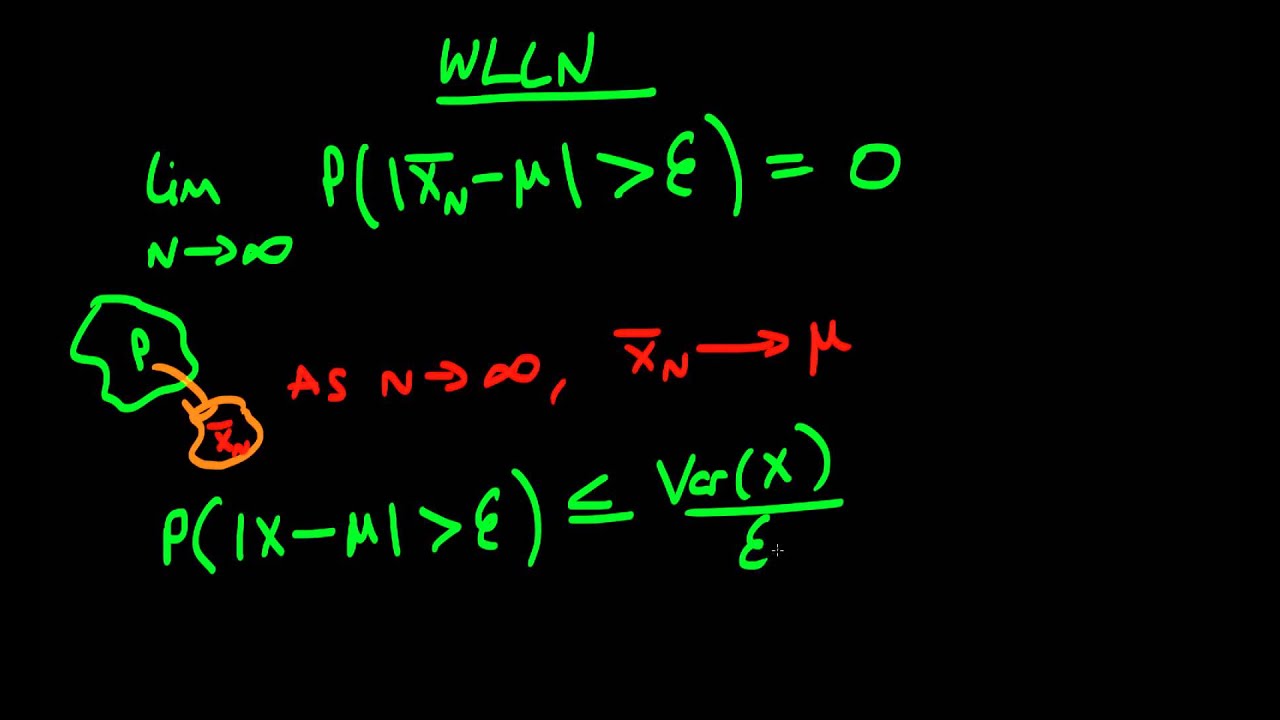
Показать описание
A proof of the weak law of large numbers
L18.4 The Weak Law of Large Numbers
The weak law of large numbers proof using characteristic functions - part 1
The Weak Law of Large Numbers
【 Proof is for the weak! 】
statement and proof of weak law of large numbers
Ben Heidenreich: A Proof of the Weak Gravity Conjecture in Perturbative Bosonic String Theory
Conservativity of Weak Kőnig's Lemma (a proof from the book)
Weak Perfect Graph Theorem
The Weak Law of Large Numbers (Proof)
The weak law of large numbers proof using characteristic functions - part 2
Proof They Want You WEAK
Proof of Strong law of large numbers and weak law of large numbers
Weak Law of Large Numbers (WLLNs) and Examples
Weak Duality Theorem | Proof in 4 lines
Proof by induction | Sequences, series and induction | Precalculus | Khan Academy
Weak law of large numbers
proof not all CAMARA MAN not scared or weak '
Weak Points in Serverless Architectures: A Proof of Concept
proof by weak induction
7. Weak Law of Large Numbers - WLLN - State and Prove - Complete Concept
WEAK LAW OF LARGE NUMBERS: SIMPLE PROOF
Proof Of Weak Īmān! | Islamic Audios
Proof of 'Weak and Strong induction are equivalent' And example statement.
Комментарии
 0:04:04
0:04:04
 0:07:31
0:07:31
 0:09:25
0:09:25
 0:05:50
0:05:50
 0:00:30
0:00:30
 0:05:14
0:05:14
 1:04:14
1:04:14
 0:45:47
0:45:47
 0:10:39
0:10:39
 0:09:49
0:09:49
 0:04:22
0:04:22
 0:00:18
0:00:18
 0:38:46
0:38:46
 0:14:30
0:14:30
 0:09:30
0:09:30
 0:09:23
0:09:23
 0:07:44
0:07:44
 0:00:19
0:00:19
 0:02:43
0:02:43
 0:05:00
0:05:00
 0:06:57
0:06:57
 0:05:59
0:05:59
 0:00:16
0:00:16
 0:12:19
0:12:19