filmov
tv
Linear Algebra 1: Matrix algebra - Oxford Mathematics 1st Year Student Lecture

Показать описание
In this lecture, the second in the first year Linear Algebra 1 course, Andy Wathen address how to add, scale and, crucially, how to multiply matrices.
All first and second year lectures are followed by tutorials where students meet their tutor to go through the lecture and associated problem sheet and to talk and think more about the maths. Third and fourth year lectures are followed by classes.
All first and second year lectures are followed by tutorials where students meet their tutor to go through the lecture and associated problem sheet and to talk and think more about the maths. Third and fourth year lectures are followed by classes.
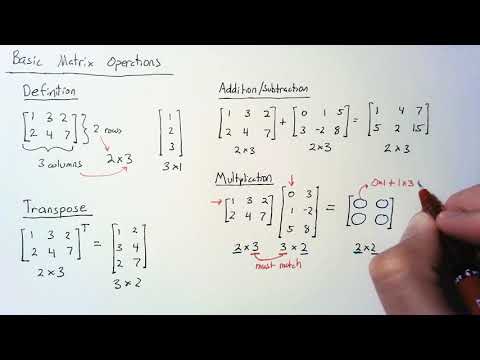
Linear Algebra - Matrix Operations
Intro to Matrices
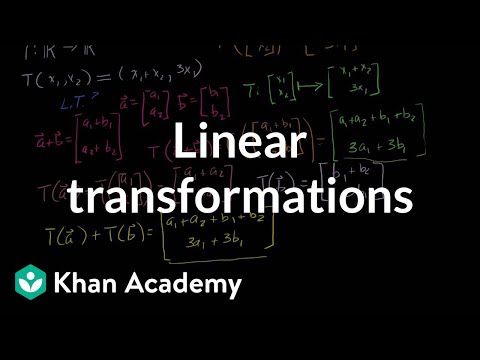
Linear transformations and matrices | Chapter 3, Essence of linear algebra
Linear Algebra - 27 - Algebraic Systems of Equations with Matrices
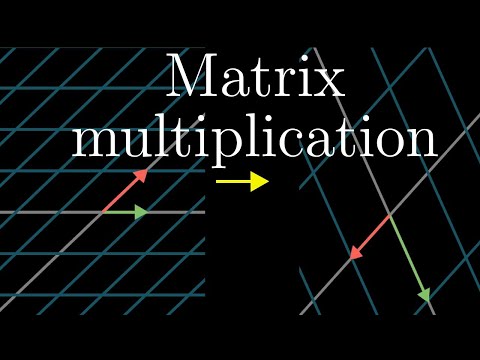
Matrix multiplication as composition | Chapter 4, Essence of linear algebra
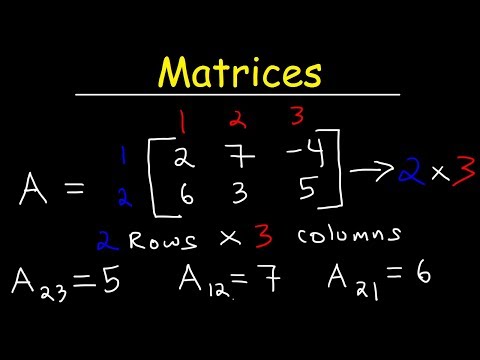
Understanding Matrices and Matrix Notation
Linear Algebra 1.8.1 Matrix Transformations
Vectors | Chapter 1, Essence of linear algebra
#maths #algebra #education #exam #linearalgebra #highschoolmath
Linear Algebra - Lecture 17 - Matrix Transformations
Dear linear algebra students, This is what matrices (and matrix manipulation) really look like
Solving Matrix Equations
Inverse matrices, column space and null space | Chapter 7, Essence of linear algebra
Part 1, Solving Using Matrices and Cramer's Rule
Linear transformations | Matrix transformations | Linear Algebra | Khan Academy
Eigenvectors and eigenvalues | Chapter 14, Essence of linear algebra
How to Find the Matrix of a Linear Transformation
Linear Algebra 1.1.1 Systems of Linear Equations
Linear Algebra 1.4.1 The Matrix Equation Ax=b
Gaussian Elimination & Row Echelon Form
Change of basis | Chapter 13, Essence of linear algebra
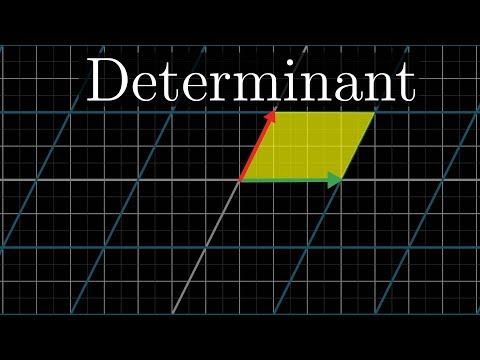
The determinant | Chapter 6, Essence of linear algebra
Essence of linear algebra preview
Row Echelon Form of the Matrix Explained | Linear Algebra
Комментарии
 0:07:08
0:07:08
 0:11:23
0:11:23
 0:10:59
0:10:59
 0:07:18
0:07:18
 0:10:04
0:10:04
 0:05:26
0:05:26
 0:13:49
0:13:49
 0:09:52
0:09:52
 0:01:00
0:01:00
 0:11:32
0:11:32
 0:16:26
0:16:26
 0:06:31
0:06:31
 0:12:09
0:12:09
 0:04:11
0:04:11
 0:13:52
0:13:52
 0:17:16
0:17:16
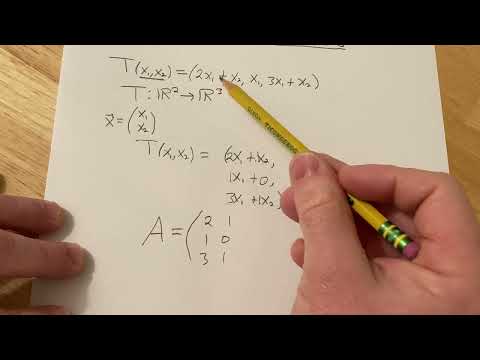 0:05:19
0:05:19
 0:18:18
0:18:18
 0:11:11
0:11:11
 0:18:40
0:18:40
 0:12:51
0:12:51
 0:10:03
0:10:03
 0:05:09
0:05:09
 0:11:11
0:11:11