filmov
tv
Null Spaces And Ranges
Показать описание
Definitions of null space, injectivity, range, and surjectivity. Fundamental theorem of linear maps. Consequences for systems of linear equations.
Null Spaces And Ranges
Inverse matrices, column space and null space | Chapter 7, Essence of linear algebra
The Null Space & Column Space of a Matrix | Algebraically & Geometrically
Null space and column space basis | Vectors and spaces | Linear Algebra | Khan Academy
Linear Algebra - Null Spaces and Ranges
Image and Kernel
Find Null Space and Nullity of a Matrix | Linear Algebra
Order, Dimension, Rank, Nullity, Null Space, Column Space of a matrix
Linear Algebra - Lecture 27: The Range and Null Space of a Matrix
Null Space Linear Algebra
KERNEL and RANGE of a LINEAR TRANSFORMATION - LINEAR ALGEBRA
Lecture 47: Linear Algebra (Range and Null space of a Linear transformation)
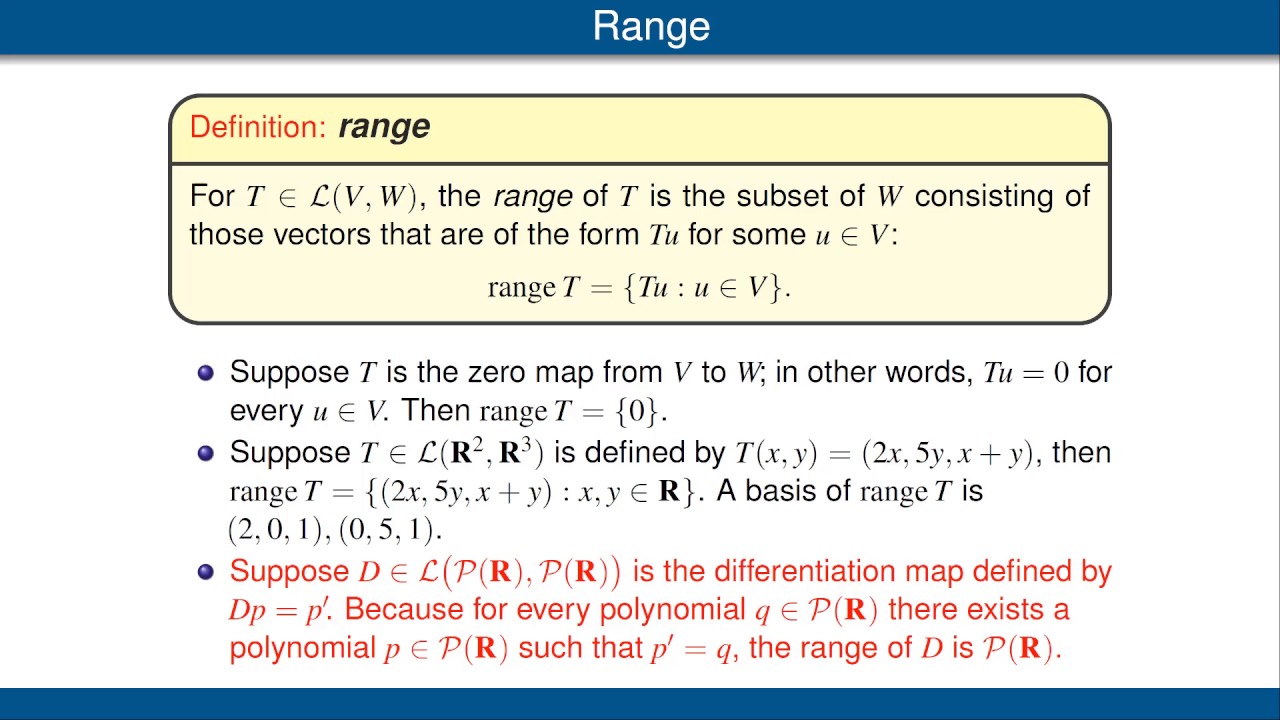
(3.B) Linear Algebra Done Right: Null Spaces and Ranges
2.2 Matrix Inverse & Spaces - #07 Range and Null Space of a Matrix: Intuition
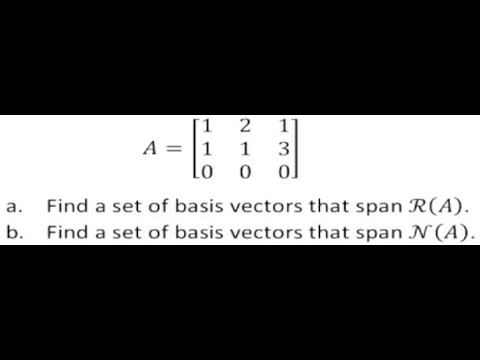
range and null space example
RPLA|MA3355|Unit5|Linear Transformation, Null Space, Nullity, Range, Rank of a Linear Transformation
#2||Range space and Null space||Linear algebra||maths for graduates
Null Space (Kernel) and Range (Image) of a Linear Transformation | Linear Algebra Explained
Finding Basis for Column Space, Row Space, and Null Space - Linear Algebra
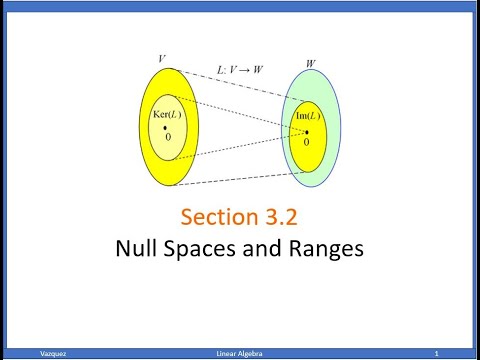
3.2 Null Spaces and Ranges
Lecture 4-2 Linear Transformations, Null Spaces and Range Spaces
Introduction to the null space of a matrix | Vectors and spaces | Linear Algebra | Khan Academy
Null Space of a Vector Space
34. NULL & RANGE SPACE : DEFINITION & EXAMPLES
Комментарии
 0:15:25
0:15:25
 0:12:09
0:12:09
 0:10:41
0:10:41
 0:25:13
0:25:13
 0:42:33
0:42:33
 0:05:35
0:05:35
 0:07:53
0:07:53
 0:14:04
0:14:04
 0:13:49
0:13:49
 0:00:59
0:00:59
 0:08:46
0:08:46
 0:11:00
0:11:00
 0:54:47
0:54:47
 0:10:19
0:10:19
 0:07:02
0:07:02
 0:07:38
0:07:38
 0:05:07
0:05:07
 0:09:57
0:09:57
 0:18:31
0:18:31
 0:29:46
0:29:46
 0:41:16
0:41:16
 0:10:23
0:10:23
 0:08:29
0:08:29
 0:07:07
0:07:07