filmov
tv
The Divergence of a Vector Field: Sources and Sinks

Показать описание
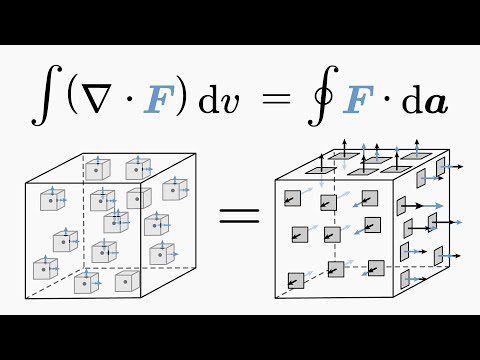
This video introduces the divergence operator from vector calculus, which takes a vector field (like the fluid flow of air in a room) and returns a scalar field quantifying how much the vector field is locally expanding or contracting at every point. The divergence is a fundamental building block in vector calculus.
@eigensteve on Twitter
This video was produced at the University of Washington
%%% CHAPTERS %%%
0:00 Introduction & Overview
3:30 The Divergence is a Linear Operator
4:41 Example of Positive Divergence
8:05 Example of Negative Divergence
10:25 Example of Zero Divergence
13:58 Vector Field is a Differential Equation
16:17 Recap
17:20 Divergence of a Gradient is the Laplacian
@eigensteve on Twitter
This video was produced at the University of Washington
%%% CHAPTERS %%%
0:00 Introduction & Overview
3:30 The Divergence is a Linear Operator
4:41 Example of Positive Divergence
8:05 Example of Negative Divergence
10:25 Example of Zero Divergence
13:58 Vector Field is a Differential Equation
16:17 Recap
17:20 Divergence of a Gradient is the Laplacian
Divergence and curl: The language of Maxwell's equations, fluid flow, and more
The Divergence of a Vector Field: Sources and Sinks
Div, Grad, and Curl: Vector Calculus Building Blocks for PDEs [Divergence, Gradient, and Curl]
Vector Fields, Divergence, and Curl
The Divergence of a Vector Field
Divergence intuition, part 1
The Divergence Theorem // Geometric Intuition & Statement // Vector Calculus
How to calculate the Divergence of a Vector function
Divergence and Curl of Vector Field | Irrotational & Solenoidal Vector With Example By-Pk SIR
Ex 1: Determine the Divergence of a Vector Field
The Divergence Theorem
Divergence of a Vector Function #vectoranalysis #vectorcalculus
1.7 The divergence of a vector field
This Downward Pointing Triangle Means Grad Div and Curl in Vector Calculus (Nabla / Del) by Parth G
Gauss Divergence Theorem. Get the DEEPEST Intuition.
Gradient, Divergence and Curl Concepts | Physics |
Divergence of a vector example | Vector calculus
Divergence of a vector field: Vector Calculus
divergence of a vector || divergence of a vector engineering mathematics
A unified view of Vector Calculus (Stoke's Theorem, Divergence Theorem & Green's Theor...
Divergence Quiz for Vector Calculus
PHYS 102 | The Divergence 1 - The Divergence of a Vector Field
Divergence and Curl
Divergence of a Vector Field (Example 1)
Комментарии
 0:15:42
0:15:42
 0:20:57
0:20:57
 0:13:02
0:13:02
 0:15:36
0:15:36
 0:05:29
0:05:29
 0:06:21
0:06:21
 0:07:35
0:07:35
 0:13:47
0:13:47
 1:05:13
1:05:13
 0:04:51
0:04:51
 0:06:31
0:06:31
 0:08:40
0:08:40
 0:02:14
0:02:14
 0:12:52
0:12:52
 0:07:53
0:07:53
 0:10:25
0:10:25
 0:02:46
0:02:46
 0:06:20
0:06:20
 0:06:28
0:06:28
 0:08:18
0:08:18
 0:08:37
0:08:37
 0:06:50
0:06:50
 0:25:33
0:25:33
 0:02:55
0:02:55