filmov
tv
Evaluating a Surface Integral - Basic Example

Показать описание
In this video, I do one example of evaluating a basic surface integral.
Evaluating Surface Integrals
Evaluating a Surface Integral - Basic Example
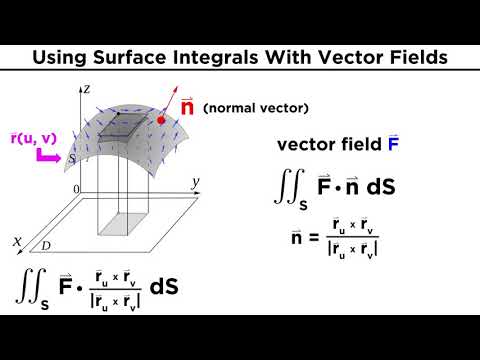
Surface Integrals // Formulas & Applications // Vector Calculus
23: Scalar and Vector Field Surface Integrals - Valuable Vector Calculus
16.7: Surface Integrals (1/2)
How to Evaluate a Surface Integral
surface integral, example 2 (KristaKingMath)
Computing the Flux Across a Surface // Vector Calculus
[CFD | ENG] Introduction to the finite volume method in CFD
surface integral (KristaKingMath)
Evaluation of Surface Integral of a Vector Field | Lecture 14 | Surface Integral @ranjankhatu
Surface Integrals with Parameterized Surface - Part 1
Surface Integrals
Surface And Flux Integrals, Parametric Surf., Divergence/Stoke's Theorem: Calculus 3 Lecture 15...
Evaluation of Surface Integral in a plane 2x+y+2z=6 in the first octant
Example of calculating a surface integral part 1 | Multivariable Calculus | Khan Academy
Surface integral ex2 part 2: Evaluating integral | Multivariable Calculus | Khan Academy
Evaluation of surface integral over the cylinder in first octant
Evaluating the Surface Integral| Surface Integrals| Vector Calculus # Engineering Mathematics
Evaluating Surface Integrals| Engineering Mathematics| VECTOR CALCULUS
Surface integral ex2 part 1: Parameterizing the surface | Multivariable Calculus | Khan Academy
Understanding Surface integrals
EVALUATING SURFACE INTEGRAL ON A HELLICOID
Surface Integrals Of Scalar-Valued Functions
Комментарии
 0:12:24
0:12:24
 0:07:08
0:07:08
 0:08:18
0:08:18
 0:27:51
0:27:51
 0:40:33
0:40:33
 0:10:26
0:10:26
 0:14:09
0:14:09
 0:08:09
0:08:09
![[CFD | ENG]](https://i.ytimg.com/vi/yBP-slVdPoA/hqdefault.jpg) 1:00:58
1:00:58
 0:08:25
0:08:25
 0:20:07
0:20:07
 0:07:08
0:07:08
 0:19:45
0:19:45
 3:31:35
3:31:35
 0:12:02
0:12:02
 0:10:45
0:10:45
 0:09:51
0:09:51
 0:08:11
0:08:11
 0:10:28
0:10:28
 0:13:35
0:13:35
 0:05:10
0:05:10
 0:01:00
0:01:00
 0:13:31
0:13:31
 0:21:14
0:21:14