filmov
tv
Solving Exponential Equation (sol'n includes complex values of X)@KasyannoEZMath
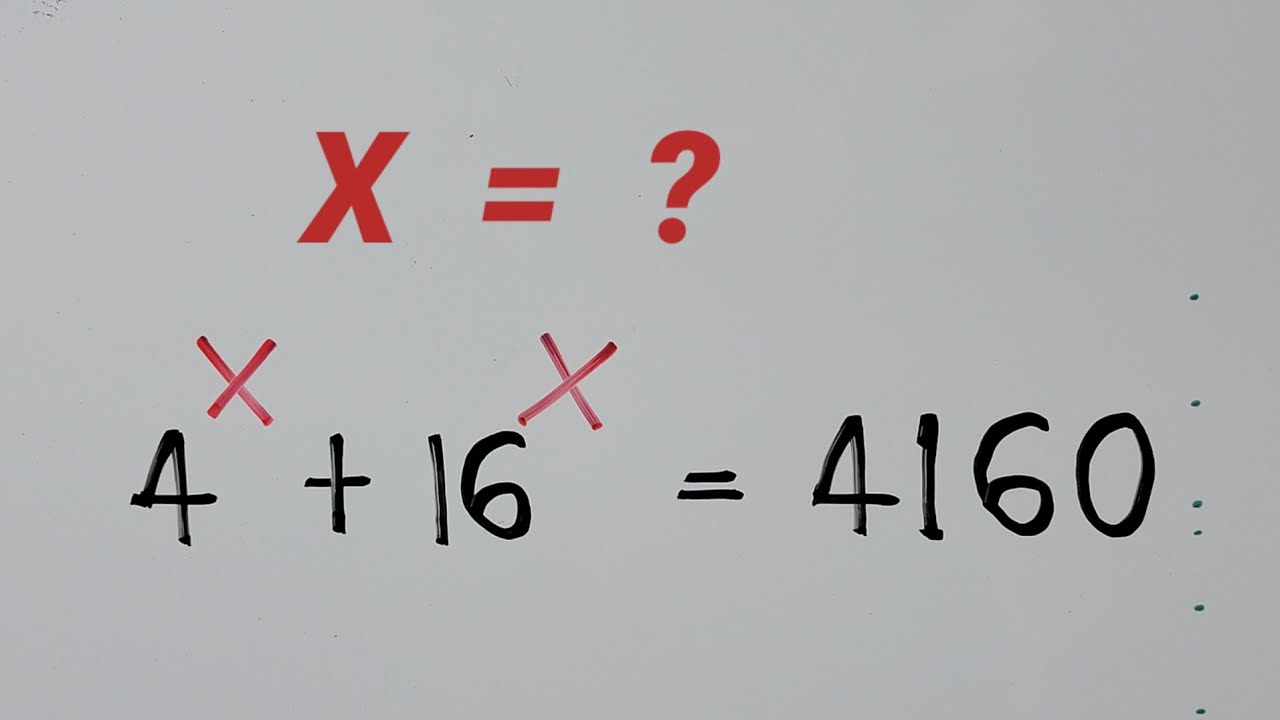
Показать описание
This tutorial video shows how to solve the given exponential equation. The solution included the complex values of X.
For those new to my channel, please subscribe to @kasyannoezmath9158 for more videos. Thank you very much!
Happy learning!
For those new to my channel, please subscribe to @kasyannoezmath9158 for more videos. Thank you very much!
Happy learning!
Solving Exponential Equation (sol'n includes complex values of X)@KasyannoEZMath
Exponential Equations - Algebra and Precalculus
Equations involving Indices
Solving Exponential Equation
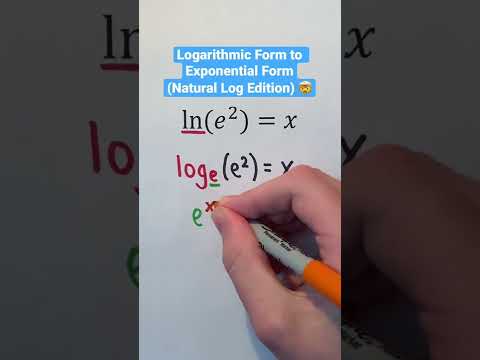
Logarithmic Form to Exponential Form (Natural Log Edition) 🤯 #Shorts #algebra #math #education
Solving Simultaneous With Exponential directly.
Solving Exponential Equation
Solving Log Equation
How To Solve Exponential Equation With Same Base #maths #learnhowtosolvetheexponentialequation
Solving Exponential Equation @KasyannoEZMath
How do you solve an exponential equation with e as the base
Solve an exponential equation by taking log of both sides & approximating the value
5 simple unsolvable equations
A Nice Olympiad Exponential Multiplication Problem #short #olympiad #mathematics #maths #exponents
Solving Exponential Equation
How to solve Challenging Math Olympiad Exponential Equation. #algebra
How to Solve Exponential Equation @KasyannoEZMath
Solving Exponential Equations for the SAT ACT
Can you solve this? | Exponential Equation | Algebra Problem.
Solving Exponential Equation @KasyannoEZMath
How to Solve Exponential Equations using Logarithms - No Common Base Present
Solving Exponential Equation @KasyannoEZMath
How to Solve This Exponential Equation @KasyannoEZMath
Exponential Expression Solution #maths #mathematics #shorts
Комментарии
 0:12:58
0:12:58
 0:05:58
0:05:58
 0:06:10
0:06:10
 0:02:56
0:02:56
 0:00:28
0:00:28
 0:13:50
0:13:50
 0:01:00
0:01:00
 0:00:55
0:00:55
 0:01:00
0:01:00
 0:00:59
0:00:59
 0:02:47
0:02:47
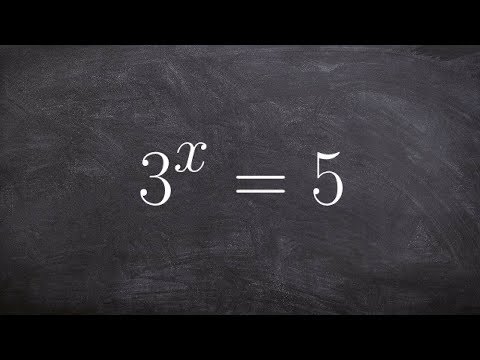 0:03:23
0:03:23
 0:00:50
0:00:50
 0:00:52
0:00:52
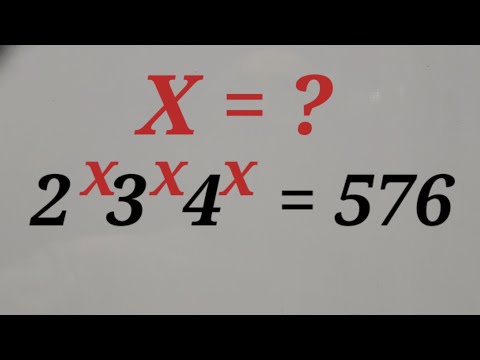 0:01:00
0:01:00
 0:15:11
0:15:11
 0:00:58
0:00:58
 0:00:38
0:00:38
 0:03:33
0:03:33
 0:00:58
0:00:58
 0:05:47
0:05:47
 0:00:57
0:00:57
 0:00:59
0:00:59
 0:01:01
0:01:01