Every Subset of a Linearly Independent Set is also Linearly Independent Proof

preview_player
Показать описание
A proof that every subset of a linearly independent set is also linearly independent.
  1. The Math Sorcerer
  2. Linear Independence
  3. Mathematics (Field Of Study)
  4. Linear Algebra (Field Of Study)
  5. math
  6. Subset
Рекомендации по теме
Every Subset of 0:03:55

Every Subset of a Linearly Independent Set is also Linearly Independent Proof

A Module Where 0:00:53

A Module Where Every Subset is Linearly Independent

VECTOR SPACE/L#23(iv)/Each subset 0:07:22

VECTOR SPACE/L#23(iv)/Each subset of a linearly independent set of vectors is linearly independent.

Chapter 04.02: Lesson:If 0:05:42

Chapter 04.02: Lesson:If Set of Vectors is Linearly Independent, Then Subset is Linearly Independent

Subset of linearly 0:06:24

Subset of linearly independent set and the set containing 0

Lec#36, Every Subset 0:12:22

Lec#36, Every Subset of Linearly Independent Set is Linearly Independent

Lecture 31 | 0:19:26

Lecture 31 | Subset of linearly independent set and superset of linearly dependent set

A subset of 0:09:48

A subset of a vectorspace is linearly dependent iff some vector of it is linear comb.of remaining

Subset of linearly 0:05:12

Subset of linearly independent set is linearly independet-Theorem-Vector Space-Linear Algebra - 29

47. Every linearly 0:05:22

47. Every linearly independent subset of W is part of a basis for W | Vector Space | Linear Algebra

How to find 0:04:41

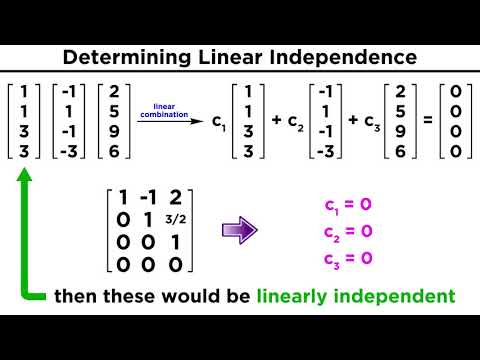
How to find out if a set of vectors are linearly independent? An example.

Any non-zero Singleton 0:04:03

Any non-zero Singleton subset of Vector Space is linearly independent | Linear Algebra

Linear combinations, span, 0:09:59

Linear combinations, span, and basis vectors | Chapter 2, Essence of linear algebra

If dim V 0:13:53

If dim V = n, then any subset of V having more than 'n' vectors is Linearly Dependent.

Linear Independence 0:12:56

Linear Independence

A Set is 0:07:52

A Set is linearly independent iff it's every finite subset is linearly independent - Vector spa...

Theorem: If S 0:11:44

Theorem: If S is linearly independent then its Subset also Linearly Independent | (Lecture 25)

Vector Space, Lec-7(Subspace 0:28:50

Vector Space, Lec-7(Subspace generated or Spanned by a Subset and Linear Span), by Dr.D.N.Garain

Not Every Subset 0:08:06

Not Every Subset is a Subspace

Lecture 34 | 0:09:28

Lecture 34 | Understanding Maximal linearly independent subset of a vector space | Linear algebra

Linear subspaces | 0:23:29

Linear subspaces | Vectors and spaces | Linear Algebra | Khan Academy

Any subset of 0:20:45

Any subset of a linearly independent set is linearly independent thorem in tamil explanation........

50. Extention Theorem 0:03:31

50. Extention Theorem | every Linearly independent subset either form basis or extend to form basis

A linearly Independent 0:16:08

A linearly Independent subset of V can be extended to form a basis of V -Vector space - lesson 40

Комментарии
Автор

Can you make a proof on any set of vectors containing a linearly dependent subset is linearly dependent.

septembabes
Автор

is the converse also true? If a set S has a linearly independent subset, then S itself is also linearly independent?

tabmax
Автор

Hi, Does this proof work? :

I started by assuming that S' is not linearly independent. Then ∃x∈V :
x = k1v1 + k2v2 + k3v3 + ... + knvn
where v1, v2, .., vn ∈ S'

=> k1v1 + k2v2 + k3v3 + ... + knvn + (- 1)x = 0
But x ∈ S. So this means we have gotten the zero vector as a linear combination of the other vectors in S with not all the scalars being zero, which makes S dependent, which is a contradiction. Hence our assumption is wrong and S' is linearly Independent.

legendarynoob