filmov
tv
A Nice and EZ Polynomial Equation
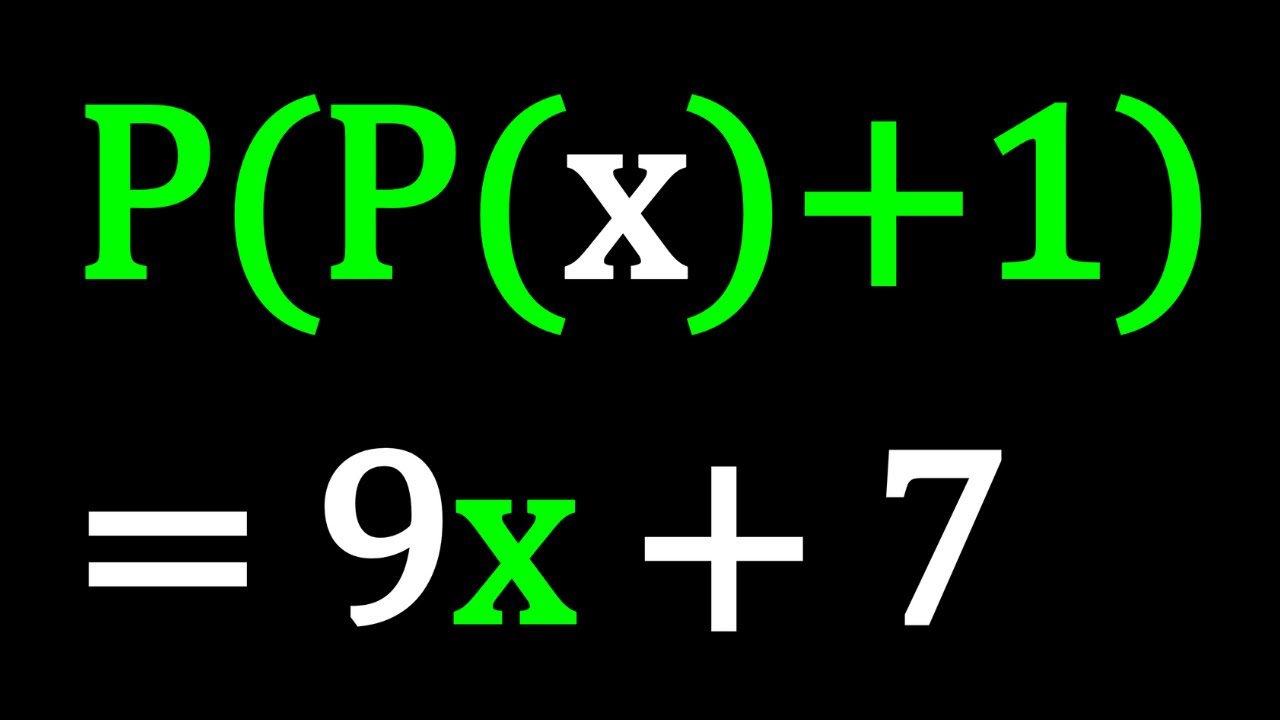
Показать описание
🤩 Hello everyone, I'm very excited to bring you a new channel (aplusbi)
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
When you purchase something from here, I will make a small percentage of commission that helps me continue making videos for you.
If you are preparing for Math Competitions and Math Olympiads, then this is the page for you!
You can find ARML books and many others here. CHECK IT OUT!!! ❤️ ❤️ ❤️
❤️ P(P(x)+1)=9x+7
If you need to post a picture of your solution or idea:
#radicals #radicalequations #algebra #calculus #differentialequations #polynomials #prealgebra #polynomialequations #numbertheory #diophantineequations #comparingnumbers #trigonometry #trigonometricequations #complexnumbers #math #mathcompetition #olympiad #matholympiad #mathematics #sybermath #aplusbi #shortsofsyber #iit #iitjee #iitjeepreparation #iitjeemaths #exponentialequations #exponents #exponential #exponent #systemsofequations #systems
#functionalequations #functions #function #maths #counting #sequencesandseries
#algebra #numbertheory #geometry #calculus #counting #mathcontests #mathcompetitions
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
When you purchase something from here, I will make a small percentage of commission that helps me continue making videos for you.
If you are preparing for Math Competitions and Math Olympiads, then this is the page for you!
You can find ARML books and many others here. CHECK IT OUT!!! ❤️ ❤️ ❤️
❤️ P(P(x)+1)=9x+7
If you need to post a picture of your solution or idea:
#radicals #radicalequations #algebra #calculus #differentialequations #polynomials #prealgebra #polynomialequations #numbertheory #diophantineequations #comparingnumbers #trigonometry #trigonometricequations #complexnumbers #math #mathcompetition #olympiad #matholympiad #mathematics #sybermath #aplusbi #shortsofsyber #iit #iitjee #iitjeepreparation #iitjeemaths #exponentialequations #exponents #exponential #exponent #systemsofequations #systems
#functionalequations #functions #function #maths #counting #sequencesandseries
#algebra #numbertheory #geometry #calculus #counting #mathcontests #mathcompetitions
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
A Nice and EZ Polynomial Equation
A Nice, Quick and Easy Polynomial Equation
A Nice Polynomial Equation | Algebra
Solving a Nice Polynomial Equation
Polynomials - Classifying Monomials, Binomials & Trinomials - Degree & Leading Coefficient
A Nice, Quick, and Easy Polynomial System
How To Factor Polynomials The Easy Way!
Algebra Basics: What Are Polynomials? - Math Antics
SHS 1 Elective Math - How To Solve Polynomial Functions
Long Division With Polynomials - The Easy Way!
A Nice Polynomial Equation
A Quick and Easy Polynomial Differential Equation
A Nice Polynomial System
A Nice Polynomial Equation from Croatia 🇭🇷
Adding Polynomials | Polynomial Review #math #algebra #polynomials #shorts
Solving a Polynomial Equation | jensenmath.ca #maths #math #advancedfunctions
A Nice System of Equations | Polynomials
A Nice Polynomial Equation | Math Olympiads
Introduction to Polynomials
Polynomial long division example in less than 1 minute #shorts
Taylor Polynomial Dance
I love this SAT ACT polynomial Question
Solving a Nice Polynomial System in Two Ways
Polynomials || IIT&JEE Questions NO 20 || X Class
Комментарии
 0:08:08
0:08:08
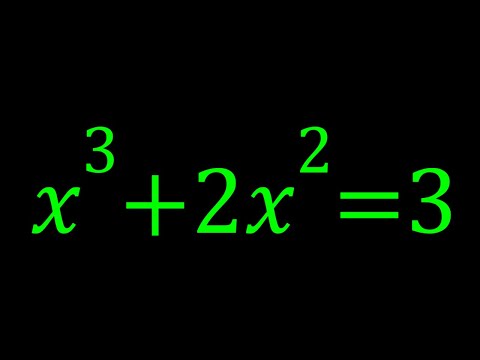 0:09:37
0:09:37
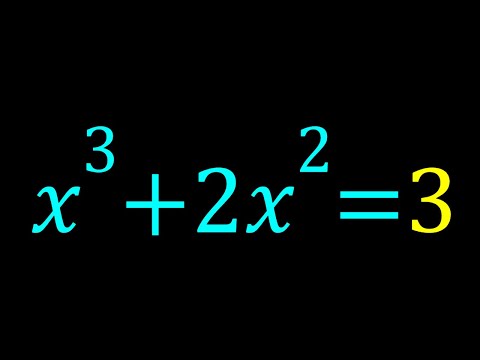 0:10:00
0:10:00
 0:05:53
0:05:53
 0:11:13
0:11:13
 0:08:27
0:08:27
 0:11:54
0:11:54
 0:11:09
0:11:09
 0:14:16
0:14:16
 0:12:12
0:12:12
 0:00:51
0:00:51
 0:08:20
0:08:20
 0:08:13
0:08:13
 0:09:27
0:09:27
 0:00:52
0:00:52
 0:00:59
0:00:59
 0:10:38
0:10:38
 0:09:32
0:09:32
 0:05:13
0:05:13
 0:00:59
0:00:59
 0:00:15
0:00:15
 0:00:59
0:00:59
 0:09:27
0:09:27
 0:00:25
0:00:25