filmov
tv
Understanding Quantum Mechanics #1: It’s not about discreteness

Показать описание
This must be one of the most common misunderstandings about quantum mechanics, that quantum mechanics is about making things discrete. But is an understandable misunderstanding because the word “quantum” suggests that quantum mechanics is about small amounts of something. Indeed, if you ask Google for the meaning of quantum, it offers the definition “a discrete quantity of energy proportional in magnitude to the frequency of the radiation it represents.” Problem is that just because energy is proportional to frequency does not mean it is discrete. In fact, in general it is not.
Understanding Quantum Mechanics #1: It’s not about discreteness
Brian Cox explains quantum mechanics in 60 seconds - BBC News
Quantum Mechanics - Part 1: Crash Course Physics #43
What Is Quantum Mechanics Explained
Quantum Mechanics Explained in Ridiculously Simple Words
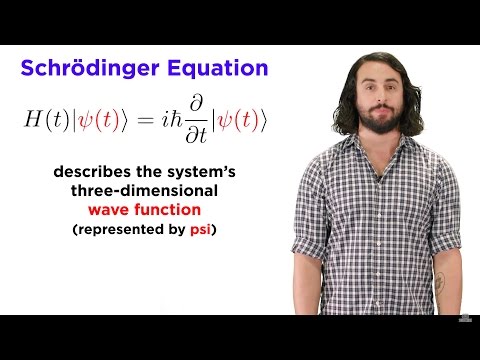
Quantum Mechanics and the Schrödinger Equation
If You Don't Understand Quantum Physics, Try This!
Understanding Quantum Mechanics
Catalysis in Single-Atom Alloys: Quantum Magic
Understanding Quantum Mechanics #2: Superposition and Entanglement
Quantum Wavefunction in 60 Seconds #shorts
Quantum Mechanics for Dummies
Orbitals, Atomic Energy Levels, & Sublevels Explained - Basic Introduction to Quantum Numbers
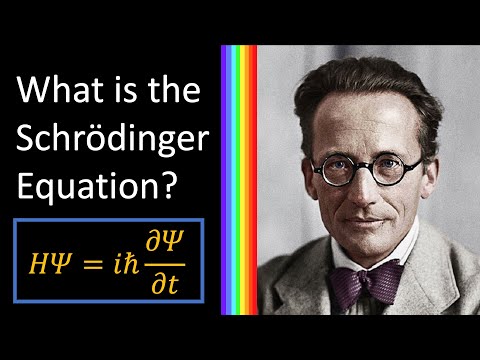
What is the Schrödinger Equation? A basic introduction to Quantum Mechanics
Understanding Quantum Mechanics #4: It's not so difficult!
Physics - Chapt. 66 Quantum Mechanics (1 of 9) What Is Quantum Mechanics?
What is quantum mechanics really all about?
The SIMPLEST Explanation of QUANTUM MECHANICS in the Universe!
What Is Quantum Mechanics & How's It Different From Classical Mechanics? | Quantum Physics ...
Understanding Quantum Mechanics #3: Non-locality
Ch 1: Why linear algebra? | Maths of Quantum Mechanics
Quantum Numbers, Atomic Orbitals, and Electron Configurations
Understanding Quantum Mechanics #6: It's not just a theory for small things
Some light quantum mechanics (with minutephysics)
Комментарии
 0:03:07
0:03:07
 0:01:22
0:01:22
 0:08:45
0:08:45
 0:12:03
0:12:03
 0:07:47
0:07:47
 0:06:28
0:06:28
 0:12:45
0:12:45
 0:11:01
0:11:01
 0:01:00
0:01:00
 0:05:42
0:05:42
 0:00:59
0:00:59
 0:22:00
0:22:00
 0:11:19
0:11:19
 1:27:34
1:27:34
 0:08:05
0:08:05
 0:05:54
0:05:54
 0:10:19
0:10:19
 0:14:00
0:14:00
 0:08:21
0:08:21
 0:07:09
0:07:09
 0:11:18
0:11:18
 0:08:42
0:08:42
 0:08:04
0:08:04
 0:22:22
0:22:22