filmov
tv
Proof: Supremum of {1/n} = 1 | Real Analysis

Показать описание
The supremum of the set containing all reciprocals of natural numbers is 1. That is, 1 is the least upper bound of {1/n | n is natural}. We prove this supremum in today's real analysis lesson using the epsilon definition of supremum!
Thanks to Nasser Alhouti, Robert Rennie, Barbara Sharrock, and Lyndon for their generous support on Patreon!
I hope you find this video helpful, and be sure to ask any questions down in the comments!
+WRATH OF MATH+
Follow Wrath of Math on...
Thanks to Nasser Alhouti, Robert Rennie, Barbara Sharrock, and Lyndon for their generous support on Patreon!
I hope you find this video helpful, and be sure to ask any questions down in the comments!
+WRATH OF MATH+
Follow Wrath of Math on...
Proof: Supremum of {1/n} = 1 | Real Analysis
Proof: Supremum of {n/(n+1)} = 1 | Real Analysis
Sup(1- 1/n), n is natural number | Sup(A) and Inf(A) | Use of Archimedean Property
Proof: Supremum and Infimum are Unique | Real Analysis
How to use the epsilon definition of sup in a proof
Proof: Infimum of {1/n} = 0 | Real Analysis
Definition of Supremum and Infimum of a Set | Real Analysis
2.5 Supremum: proof - sup(0,1)=1
The derivative and and mean value theorem | Advanced calculus bsc 2nd year ex 2.2
Real Analysis 6 | Supremum and Infimum
How to use Definition 1 of Sup in a proof
Show that Inf(1/n)=0
Proof! sup(1-1/n)=1 (Real Analysis)
Fundamental Properties of Supremum and Infimum, Including sup(A + B) = sup(A) + sup(B)
Example 1 of Finding Supremum and Infimum
Epsilon Definition of Supremum and Infimum | Real Analysis
Intro to Real Analysis - Video 6: ex of proof showing sup{1/n}=1 and inf{1/n}=0
Supremum and Infimum (Sup and Inf) Definitions and Examples, Prove sup{cos(n) | n in N} Equals One
Supremum, Infimum: Definition and Explanation
Proof of the Associativity of the Supremum (Sup{ Sup{ a, b }, c } = Sup{ a, Sup{ b, c } })
#Adv_Calculus #upper_bound (u-1/n) is not upper bound but (u+1/n) is upper bound of S.URDU/HINDI
Sup(m/n : m , n are natural numbers where m is less than 2n) | Infimum | Supremum
Proof! sup(aS)=a(sup S) (Real Analysis)
Supremum and Infimum of a Sequence
Комментарии
 0:04:34
0:04:34
 0:10:20
0:10:20
 0:04:28
0:04:28
 0:04:21
0:04:21
 0:07:54
0:07:54
 0:05:21
0:05:21
 0:13:51
0:13:51
 0:03:21
0:03:21
 0:28:42
0:28:42
 0:09:10
0:09:10
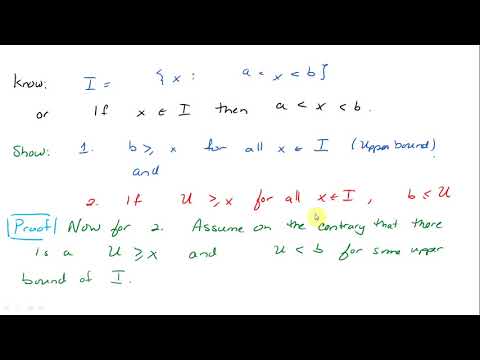 0:07:19
0:07:19
 0:05:52
0:05:52
 0:04:46
0:04:46
 0:15:46
0:15:46
 0:10:12
0:10:12
 0:11:07
0:11:07
 0:17:44
0:17:44
 0:26:04
0:26:04
 0:07:31
0:07:31
 0:06:08
0:06:08
 0:03:53
0:03:53
 0:03:53
0:03:53
 0:05:48
0:05:48
 0:13:20
0:13:20