filmov
tv
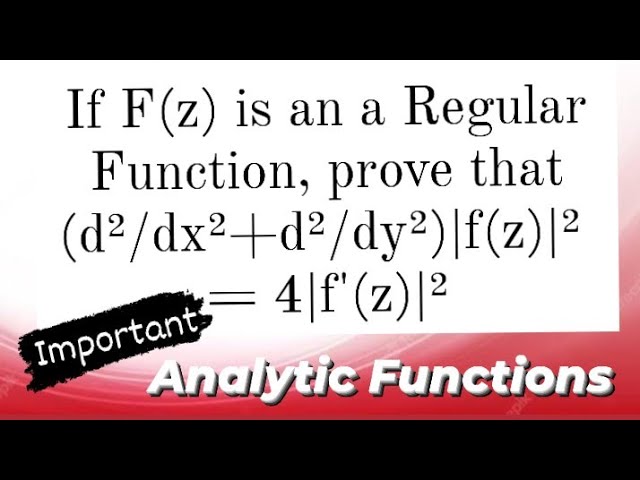
If f(z) is a Regular Function, prove that (d²/dx² + d²/dy²)|f(z)|² = 4|f'(z)|² ANALYTIC FUNCTION
Показать описание
0:11:11
If f(z) is a Regular Function, prove that (d²/dx² + d²/dy²)|f(z)|² = 4|f'(z)|² ANALYTIC FUNCTI...
0:20:27
#25 || Problem# ||If f(z) is analytic, show that [𝝏^𝟐/〖𝝏𝒙〗^𝟐 +𝝏^𝟐/〖𝝏𝒚〗^𝟐 ] |𝒇(𝒛)|^𝟐=𝟒|𝒇^′ (𝒛)|^𝟐 ||...
0:08:49
Prove that if f(z) is analytic at z_0, then f(z) is continuous at z_0.
0:05:36
Cauchy Riemann Show that [(∂^2/∂x^2)+(∂^2/∂y^2)]|f(z)|^2 = 4|f’(z)|^2
0:16:13
#26 || Problem#6 || show that [𝝏/𝝏𝒙 |𝒇(𝒛)|]^𝟐+[𝝏/𝝏𝒚 |𝒇(𝒛)|]^𝟐=|𝒇^′ (𝒛)|^𝟐 || 18MAT41||...
0:13:23
13. Construction of Analytic Function | Problem#5 | Most Important | Complete Concept
0:05:19
Problem of Analytic Function | Analytic Function Problems | Analytic Complex Function
0:04:31
If f(z)=u+iv is an Analytic function such that f'(z)≠0, Prove that : {(∂²/∂x²)+(∂²/∂y²)}log|f&a...
0:00:57
Nagar Baul James Live Concert in ICCB |Amar sonar Bangla|Yamaha Fz-s v4 launching event | NBJtheguru
0:18:13
Examples on Analytic function ( Cauchy-Reimann equations )
0:04:28
If f and it's conjugate are analytic in a domain, then f is constant, Proof
0:06:59
Determine the constants a and b if the function f(z) is analytic
0:07:28
Easily explain how to find analytic function f(z) given real part by easy maths easy tricks
0:06:44
f(z) is not Regular at Origin, although Cauchy Riemann Equations are satisfied at Origin.
0:11:27
if f'(z) =0 then f is constant | complex analysis | differentiability |
0:06:32
Show f(z) = √|xy| is not analytic at origin although the C-R equations are satisfied at that point....
0:26:42
Complex Analysis #11 (Imp.) | Proving an Expression on being Analytic Function
0:13:37
@btechmathshub7050Functions Of A Complex Variable-Problem
0:18:21
Sufficient Condition for a Function to be Analytic with proof
0:04:46
Non Analytic Function | Non-analytic Functions of a Complex Variable | f(z) = sqrt(|xy|)
0:10:24
Theorem: If f(z) is analytic function then u and v are both HarmonicFunctions Complex Analysis
0:07:06
Analytic Function: Show that f(z) = z̄ is continuous at z= zₒ but not analytic there.
0:07:21
if f(z) is an analytic function with constant modulus then it is constant
0:25:48
Cauchy-Riemann equations are satisfied for the function f(z) but f(z) is not analytic at origin
Комментарии