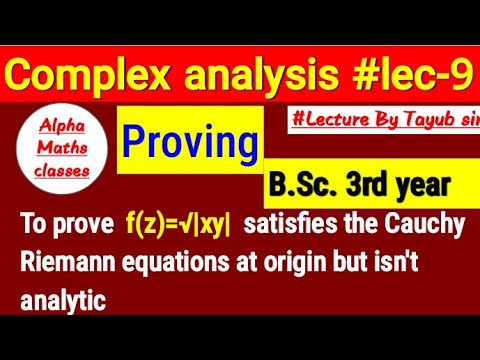
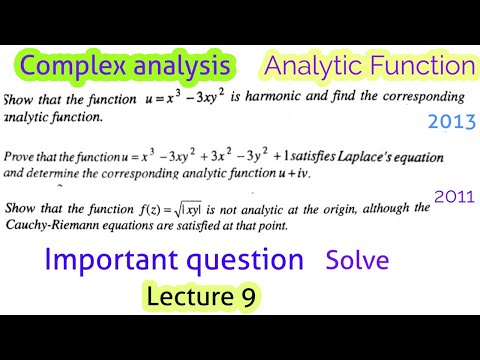
Show f(z) = √|xy| is not analytic at origin although the C-R equations are satisfied at that point.

Показать описание

Complex Analysis Theorem from Analytic function
Statement/Theorem /Prove that :-

Show that the function f(z) = sqrt(|xy|) or √|xy| is not analytic at the origin although the Cauchy-Riemann equations are satisfied at that point.

Solution.
Let f(z) = u(x, y) + iv(x, y) =√|xy|
Here

u(x,y)= √|xy| and v(x,y)=0

At the origin zₒ=(0,0),
(∂u/∂x) = lim ₓ→ₒ {u(x, 0) - u(0, 0)}/x
⇒ (∂u/∂x)= (0 - 0)/x
⇒(∂u/∂x) = 0

and

(∂v/∂y) = lim ᵧ→ₒ {v(0, y) - v(0, 0)}/y
⇒(∂v/∂y)= (0 - 0)/y
⇒(∂v/∂y) = 0

Here ,
∵(∂u/∂x) = 0 and (∂v/∂y) = 0
∴(∂u/∂x) = (∂v/∂y)
Hence Cauchy-Riemann equations are satisfied at the origin zₒ=(0,0).

Now by property of differentiability...

f'(0)=lim z→o {f(z)-f(0)}/z
⇒f'(0)=lim z→o {√|xy|-0}/(x+iy)
⇒f'(0)=lim z→o √|xy|/(x+iy)
Now let a line y=mx, z→o we get

⇒f'(0)=lim z→o √|mx²|/(x+imx)
Simplify
⇒f'(0)=lim z→o √|m|/(1+im)
Here f'(0) is dependent on slop m thus the limit is not unique

∴ differentiability is not exist so that f(z) is not Analytic function at the origin zₒ=(0,0).

Hence Proved...!!!
.
.
.
.
.
.
.
.
Kindly join us for CSIR-UGC National Eligibility Test (NET) for Junior Research Fellowship and Lecturer-ship COMMON SYLLABUS FOR PART 'B' AND 'C' MATHEMATICAL SCIENCE
.
.
.
.
.
All BSc, B.Tech, MSc, MA Mathematics students join with us A great platform to get knowledge in Mathematics and science... Stay tuned....for detailed videos on whole SYLLABUS with topic wise...
.
.
.
.
.
.
.
#complexanalysis #csirnet #analyticfunction
.
Videos links 🔥🔥🔥

Play List ( Complex Analysis)

Algorithm of Basic Concepts

Рекомендации по теме

Комментарии

Sir ji ap khud practice kr rha ya samjah rha hai students ko

lilajyoti

Intro dekhke ko to laga sir horror movie dikhane wale h

Nightmarenarratives

Sir ye formula kab aaye kab lagana hai ye kaise pata chalega

Mridulmishra

Cauchy reimann eqn necessary condition hai lekin sufficient
condition nhi hote hai analytic proof krne k liye isme CR eqn satisfy toh hore hai lekin necessary condition ka ky wo hote hai ki jo partial derivatives hai wo b toh continuous hone chaeye na toh isme wo nhi ho rhe (the single valued fb fz is analytic in domain D if the four partial derivatives ux vx uy vy are continuous and satisfy CR eqn ) in each point D !! Isliye ye analytic nhi hai

pandey

This type of application questions are not given in my college.... 🙂🥲
Thankyou sir for doing this video 🙏🏻

Pranavxyx

Sir book mai bhi yehi h wahan se rat lete kuchh sajhane k liye aaye thr

ishujoshivlogs

Continuous formulae use cheyadaniki avvada

harikaasi

Sir solution toh google se bhi uth jata hai... Aapne ek line bhi ni samjhaya hai

shubhampandey

😂phle khid smjh lo chle ho utube m pdane ko

pandey

Show f(z) = √|xy| is not analytic at origin although the C-R equations are satisfied at that point.

Show f(z) = √|xy| is not analytic at origin although the C-R equations are satisfied at that point....

Analytic Function | C-R Equations | f(z) = sqrt(|xy|) is not regular at origin | LACA

Show that the function f(z) =xy+iy is continuous everywhere but not analytic. Analytic Function

Show that f(z) = xy + iy is everywhere continuous but is not analytic.

Complex analysis| #lec-9 f(z)=√|xy| satisfies the Cauchy Riemann eq at origin but isn't #analy...

Show that the function f(z)=√|xy| is not anyalatic at the origin, Although the Cauchy Remain equatio...

Show that the function f(z)=√|xy| is not analytic at the origin, although.....|| complex analysis

Prove f(x,y) = sqrt(xy) is continuous at (1,4)

Show that the function f(x,y) = e^(x-y) is a solution to the PDE

Lec 12 | Analytic function important question || Complex analysis || `f(z)=xy+iy` | f(z) =`|z|^(2)`

Show that u(x,y) = xy is a harmonic function and find the harmonic conjugate v(x,y) such that f(z) …...

Analytic function based Questions| Analytic function important question | `f(z)=sqrt(|xy|)`

Complex Analysis #2 (V.Imp.) | Checking Analytic Function | Verifying Cauchy Riemann Equations

Cauchy Riemann Show that u-v = (x-y)(x2+4xy+y2) find f(z)

Prove that the function f(x,y)=√(|xy| ) is not differentiable at the point (0,0)but f_x f_y bo......

Difference Between Partial and Total Derivative

How to Find the Matrix of a Linear Transformation

Logarithms: Show that log(x²/yz) + log(y²/xz) + log(z²/xy) = 0.

If z=f(x y) x=rcosӨ y=rsinӨ Find (Әz/Әx)^2 + (Әz/Әy)^2 PARTIAL DIFFERENTIATION

ABC Song - Learn English Alphabet for Children with Diana

Show that R={(x,y): 5 divides x-y} is an equivalence relation on Z

complement of F = xy' + x'y

Partial Derivatives of z =e^(xy)

Second Order Mixed Partials of z = e^(xy)