Numerical-relativistic simulation of a binary neutron star merger

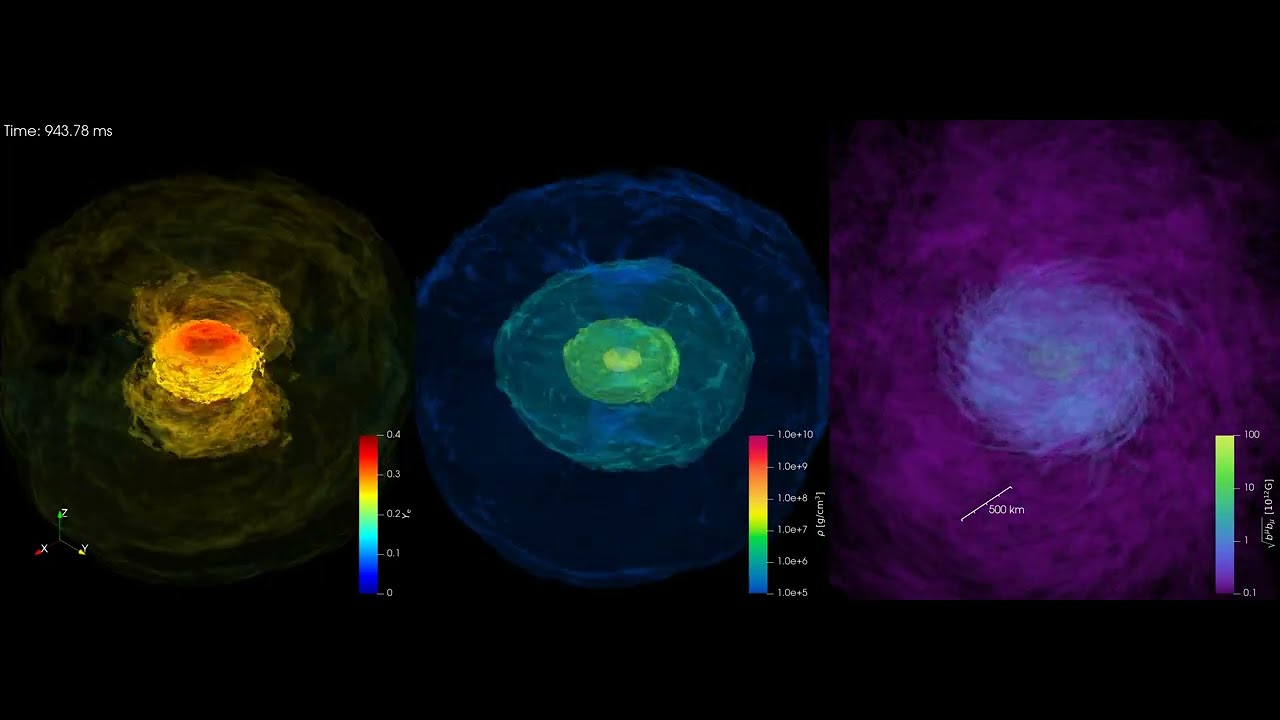
The visualization shows the numerical-relativistic simulation of a binary neutron star merger. The simulation was done on the Japanese supercomputer “Fugaku” and used about 72 million CPU hours (corresponding to 8200 CPU years) with 20,736 CPUs. The neutron stars have masses of 1.2 and 1.5 solar masses, respectively, which is consistent with the parameters of the merger observed in August 2017 (GW170817). The data was generated during a one second-long general-relativistic neutrino-radiation magnetohydrodynamic simulation. The visualization shows the electron fraction on the left, the density in the center, and the magnetic field strength (10^15 Gauss) on the right.
©K. Hayashi, K. Kiuchi (Max Planck Institute for Gravitational Physics & Kyoto University)

[Sanchuniathon]

It's remarkable how they share some of the structural characteristics of atomic orbitals. That's what I love about gravity: there's so many analogues to classical or quantum ideas, they're often buried in the details, and those analogues aren't in any way strongly connected with the other phenomena. In some ways, what we're witnessing is the purest force of nature express itself -- gravity is often so faint, but watching it develop at high gravities is so wonderful. Watching the material properties of gravity arise like this gives me hope for the advancing of our understanding.

[aSpyIntheHaus]

Guys I understand that running these simulations is expensive and the computing time is precious, but I'm going to need more than 46 seconds. 😊 I want to see where all that stuff end up? How much gets throw out and away? How much falls back in? How long until the Kerbals arrive to make their little Dyson Sphere around it?

[-_Nuke_-]

Imagine seeing that in Elite Dangerous :D

[TehNetherlands]

When considering the density visualization, I notice that there's a sudden transition from one density range to the next. Is this a limitation of the simulation or is there expected to be a sudden transition like that?
Secondly, even the lowest density simulated is 1.0e5 g/cm3, which is several orders of magnitude denser than gold. Wow!
The core of the Earth is thought to have a density of roughly 12 g/cm3. What we see in the simulation are densities in excess of 100.000 g/cm3 as far out as ~1000 km.
So essentially we're looking at a turbulent spheroid of relativistic matter roughly the size of Europe, far denser than anything on Earth(!!). What is the actual phase of this matter? Is it an ultra-dense plasma of exotic isotopes? Do elements spontaneously form as the density decreases towards the outer envelope of the object? Mind-blowing stuff.

[pratikt]

After generating the data, which software/language is used for the actual visualisation?