filmov
tv
Abstract Algebra: Exam 3 Review, Overview of Divisibility in Integral Domains, Ring Homomorphisms

Показать описание
We review for abstract algebra exam 3 by mostly focusing on providing an overview of the topics on divisibility in integral domains. This includes: 1) the definitions of irreducible elements and prime elements, 2) the fact that every prime is irreducible, 3) every irreducible is prime in a principal ideal domain (PID), 4) every principal ideal domain (PID) is a unique factorization domain (UFD), 5) every Euclidean domain (ED) is a principal ideal domain (PID) and unique factorization domain, and 6) the fact that Z[sqrt(-5)] is not a unique factorization domain. We also look at old exam questions related to ring homomorphisms and finite Abelian groups.
Links and resources
===============================
⏱️TIMESTAMPS⏱️
AMAZON ASSOCIATE
As an Amazon Associate I earn from qualifying purchases.
Links and resources
===============================
⏱️TIMESTAMPS⏱️
AMAZON ASSOCIATE
As an Amazon Associate I earn from qualifying purchases.
Abstract Algebra Exam 3 Review Problems and Solutions (Basic Ring Theory and Field Theory)
Abstract Algebra Review for Exam 3
Abstract Algebra: Exam 3 Review, Overview of Divisibility in Integral Domains, Ring Homomorphisms
ONLY 3 Students Passed?! This Hard Abstract Algebra Exam made 96% of Math Students FAIL!
Abstract Algebra Final Exam Review Problems and Solutions
Abstract Algebra: review for Test 3, 12-4-17
Review Abstract Algebra in 30 Minutes
Legendary Book for Learning Abstract Algebra
Abstract Algebra Exam 1 Review Problems and Solutions
Linear Algebra Exam 3 Review Problems and Solutions
Gilbert Strang: Linear Algebra vs Calculus
A Brief Introduction to Normal Subgroups of a Group in Abstract Algebra
Math 1210 - Exam 3 Review
When mathematicians get bored (ep1)
Rings Can Be Strange in Abstract Algebra
How To Solve Math Percentage Word Problem?
BEST DEFENCE ACADEMY IN DEHRADUN | NDA FOUNDATION COURSE AFTER 10TH | NDA COACHING #shorts #nda #ssb
Contemporary Abstract Algebra by Joseph Gallian #shorts
Topics to Expect on an Abstract Algebra Final Exam
Memorization Trick for Graphing Functions Part 1 | Algebra Math Hack #shorts #math #school
Math 140 Exam 3 topic review part 1
EGR214 Exam 3: Review Problems 9, 10, 11
Abstract Algebra: questions for Test 3, 12-5-17, part 1
Stop Trying to Understand Math, Do THIS Instead
Комментарии
 1:33:26
1:33:26
 0:52:52
0:52:52
 1:02:42
1:02:42
 0:27:13
0:27:13
 1:30:20
1:30:20
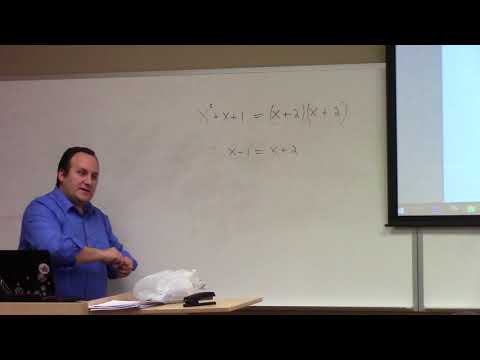 0:47:33
0:47:33
 0:30:24
0:30:24
 0:01:00
0:01:00
 1:22:00
1:22:00
 1:18:42
1:18:42
 0:02:14
0:02:14
 0:04:03
0:04:03
 0:31:28
0:31:28
 0:00:37
0:00:37
 0:44:27
0:44:27
 0:00:29
0:00:29
 0:00:15
0:00:15
 0:00:16
0:00:16
 1:04:52
1:04:52
 0:00:15
0:00:15
 0:33:40
0:33:40
 0:42:13
0:42:13
 0:59:51
0:59:51
 0:05:21
0:05:21