filmov
tv
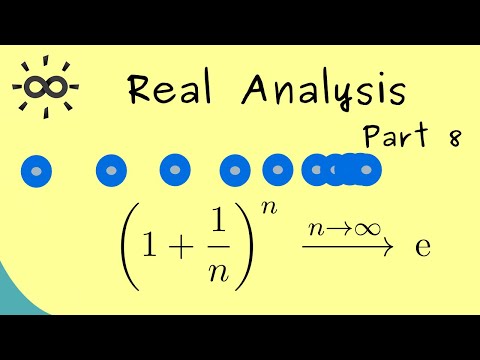
Real Analysis 8 | Example Calculation

Показать описание
Please consider to support me if this video was helpful such that I can continue to produce them :)
🙏 Thanks to all supporters! They are mentioned in the credits of the video :)
This is my video series about Real Analysis. We talk about sequences, series, continuous functions, differentiable functions, and integral. I hope that it will help everyone who wants to learn about it.
x
00:00 Intro
00:21 Recalling the monotone convergence criterion
00:55 Introducing the example
01:21 Proving monotonicity
04:49 Proving bounded from above
08:55 End result of the example
09:46 Credits
#RealAnalysis
#Mathematics
#Calculus
#LearnMath
#Integrals
#Derivatives
I hope that this helps students, pupils and others. Have fun!
(This explanation fits to lectures for students in their first and second year of study: Mathematics for physicists, Mathematics for the natural science, Mathematics for engineers and so on)
Real Analysis 8 | Example Calculation
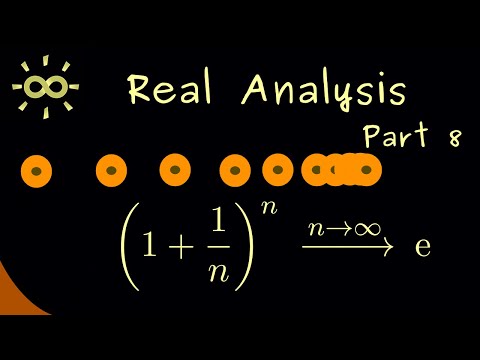
Real Analysis 8 | Example Calculation [dark version]
B.sc 2nd year Real Analysis chapter-1 example-8
Intro Real Analysis, Lec 8: Subsequences, Bolzano-Weierstrass, Cauchy Criterion, Limsup & Liminf
Real Analysis | The Supremum and Completeness of ℝ
Relations and Functions | Domain and Range | EXAMPLES | Real Analysis | Lecture 8
Real Analysis | Series | Malik & Arora | Page-111 | Example-8 | Solution
Real Analysis 13 | Open, Closed and Compact Sets
L-5 Real Analysis || Sequence & Series || Taiyari Jeet Ki
Learn Real Analysis With This Excellent Book
||Real Analysis|| Open Set Thm.5.7 Example-8, 9
Real Analysis-1 Chapter#3 Example#3.2.8 all parts complete and easy proof
The Bolzano–Weierstrass theorem, a proof from real analysis
Real Analysis 9 | Subsequences and Accumulation Values
Isaac Newton's INSANE Sleep Habits 😬
Real Analysis Exam 1 Review Problems and Solutions
11 years later ❤️ @shrads
1st yr. Vs Final yr. MBBS student 🔥🤯#shorts #neet
Intro to Open Sets (with Examples) | Real Analysis
IQ TEST
8. Definition and examples of interior point | point set topology | Real Analysis
Real Analysis | Monotone sequence theorem example.
Intro to Subsequences | Real Analysis
||Real Analysis|| Chapter-4 Example-8 lp space
Комментарии
 0:10:17
0:10:17
 0:10:03
0:10:03
 0:11:59
0:11:59
 0:57:23
0:57:23
 0:16:10
0:16:10
 0:13:05
0:13:05
 0:05:39
0:05:39
 0:09:28
0:09:28
 1:12:50
1:12:50
 0:10:40
0:10:40
 0:11:00
0:11:00
 0:23:29
0:23:29
 0:13:30
0:13:30
 0:08:18
0:08:18
 0:00:24
0:00:24
 1:05:33
1:05:33
 0:00:11
0:00:11
 0:00:20
0:00:20
 0:08:58
0:08:58
 0:00:29
0:00:29
 0:03:53
0:03:53
 0:08:46
0:08:46
 0:15:25
0:15:25
 0:08:16
0:08:16