filmov
tv
Complex analysis: Harmonic functions

Показать описание
This lecture is part of an online undergraduate course on complex analysis.
We study the question: when is a function u the real part of a holomorphic function w=u+iv? An easy necessary condition is that u mist be harmonic. We use the Caucy-Riemann equations to show that this condition is also sufficient if u is defined in a simply connected open set, and given an example to show that it need not be sufficient if u is defined on a non-simply connected set. So on simply connected open sets harmonic functions are the same as the real parts of holomorphic functions.
We study the question: when is a function u the real part of a holomorphic function w=u+iv? An easy necessary condition is that u mist be harmonic. We use the Caucy-Riemann equations to show that this condition is also sufficient if u is defined in a simply connected open set, and given an example to show that it need not be sufficient if u is defined on a non-simply connected set. So on simply connected open sets harmonic functions are the same as the real parts of holomorphic functions.
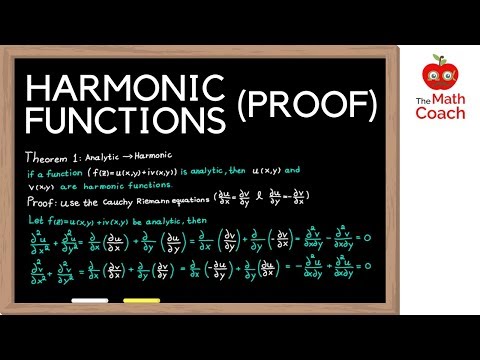
Harmonic functions | Harmonic conjugate | Complex Analysis #3
Complex Analysis 04: Harmonic Functions
Complex analysis: Harmonic functions
Harmonic Functions
Harmonic Functions and Harmonic Function Theorem - Complex Analysis by a Physicist
COMPLEX ANALYSIS- HARMONIC FUNCTIONS: WITH WORKED KNEC PAST PAPER QUESTION
Complex Analysis - Harmonic Functions
Type 2 Harmonic Function - Problem 1 and 2 - Complex Variable - Engineering Mathematics 3
w105 harmonic conjugate
Examples on Harmonic Functions ( Laplace and Cauchy-Reimann equations )
Harmonic Functions- Complex Variables | S3(2019) MAT201 Module 3|S3(2015) MA201 module1,2 KTU|Part 8
LSU Complex Analysis Lecture 25 harmonic functions
Complex Analysis #9 (Imp.) | Harmonic Function | Relation Between Analytic and Harmonic Function
Harmonic Functions & Conjugate Harmonic Functions with 4 Questions
Harmonic Functions -- Complex Analysis 9
6. Harmonic Function | Complex Variables | Complete Concept
Harmonic functions | A Quick Proof | Complex Analysis #4
Complex Analysis L06: Analytic Functions and Cauchy-Riemann Conditions
Harmonic function and Harmonic Conjugate
Harmonic Functions and Determination of Harmonic Conjugate of a function (Complex Analysis)
Complex Analysis - Short Trick To Find Harmonic Conjugate By GP Sir
Complex Analysis- Analytic Functions - Harmonic Functions -(Demo Class)
Concept of Harmonic Function - Complex Variable - Engineering Mathematics 3
Harmonic function | Harmonic conjugate | Complex Analysis | With examples | Limit breaking tamizhaz
Комментарии
 0:06:06
0:06:06
 0:13:15
0:13:15
 0:30:41
0:30:41
 0:10:56
0:10:56
 0:06:30
0:06:30
 0:42:01
0:42:01
 0:16:39
0:16:39
 0:07:41
0:07:41
 0:09:57
0:09:57
 0:25:00
0:25:00
 0:27:29
0:27:29
 0:35:48
0:35:48
 0:34:00
0:34:00
 0:33:30
0:33:30
 0:34:24
0:34:24
 0:02:27
0:02:27
 0:02:52
0:02:52
 0:43:49
0:43:49
 0:14:43
0:14:43
 0:34:02
0:34:02
 0:15:35
0:15:35
 0:39:42
0:39:42
 0:05:55
0:05:55
 0:11:56
0:11:56