filmov
tv
SFU MATH 232 6.2 Geometry of Linear Operators

Показать описание
SFU Math 232 6.2 Geometry of Linear Operators. Orthogonal transformations, orthogonal matrices, length preservation, the properties of orthogonal matrices and what geometrical they look like - think rotation and reflection.
SFU MATH 232 6.2 Geometry of Linear Operators
SFU MATH 232 6.2 Diagonalization
SFU MATH 232 6.1 Eigenvalues and Eigenvectors - PART 2
SFU MATH 232 6.1 Matrices as Transformations
SFU MATH 232 Appendix B Complex Numbers
SFU MATH 232 3.5 The Geometry of Linear Systems
SFU MATH 232 3.6 Matrices with Special Forms
SFU MATH 232 7.6 The Pivot Theorem
SFU MATH 232 7.2 Projections and the Gram Schmidt Process
SFU MATH 232 Sec 4 6 worked examples part 1
SFU MATH 232 6.1 Eigenvalues and Eigenvectors - PART 1
SFU MATH 232 3.4 Subspaces and Linear Independence
Linear Algebra: Linear Transformations in Geometry (full lecture)
SFU MATH 232 1.2 Dot Product and Orthogonality
SFU MATH 232 Sec 1.5 Dot Products and Projections in R^n
SFU MATH 232 7.7 The Projection Theorem
SFU MATH 232 7.1 Basis and Dimension
SFU MATH 232 4.4 A First Look at Eigenvalues and Eigenvectors
SFU MATH 232 3.4 Special Subspaces
SFU MATH 232 2.3 Applications to Spanning and Linear Independence
SFU MATH 232 1.3 Vector Equations of Lines and Planes
SFU MATH 232 3.2 Matrix and Linear Mappings
SFU MATH 232 2.4 Applications to Systems of Linear Equations
SFU MATH 232 7.3 Method of Least Squares (Spring 2022) 7.8 Best Approx and Least squares (Fall 2021)
Комментарии
 0:34:26
0:34:26
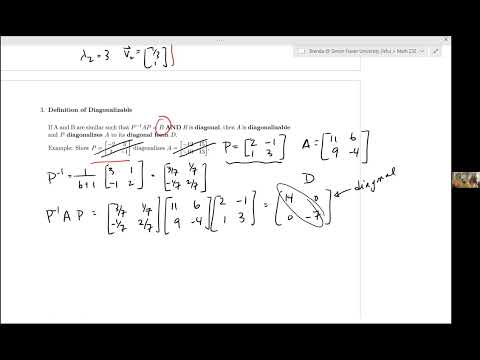 0:35:58
0:35:58
 0:56:45
0:56:45
 0:55:34
0:55:34
 0:42:43
0:42:43
 0:55:06
0:55:06
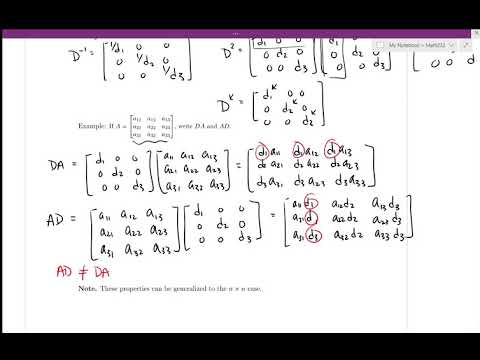 0:44:47
0:44:47
 0:23:02
0:23:02
 0:57:35
0:57:35
 0:44:40
0:44:40
 1:07:56
1:07:56
 1:19:51
1:19:51
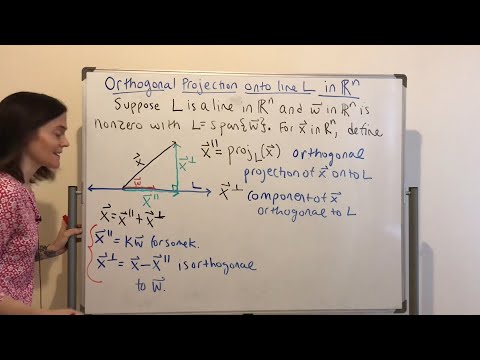 1:10:03
1:10:03
 1:03:12
1:03:12
 0:45:50
0:45:50
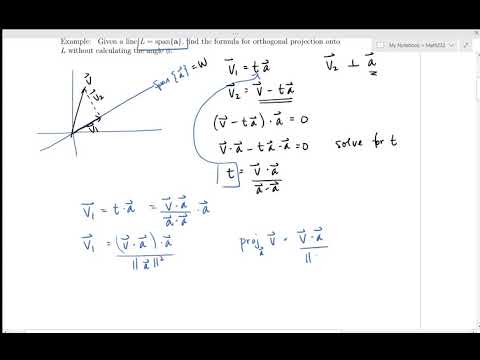 0:39:48
0:39:48
 0:38:15
0:38:15
 0:56:01
0:56:01
 0:47:55
0:47:55
 1:08:17
1:08:17
 0:56:43
0:56:43
 1:31:13
1:31:13
 0:50:25
0:50:25
 0:43:32
0:43:32