filmov
tv
SFU MATH 232 3.4 Special Subspaces

Показать описание
SFU Math 232 Section 3.4. This is the main lecture for section 3.4. This lecture details the four fundamental subspaces of a matrix - the columnspace, the rowspace, the null space and the null space of the transpose. The Rank-Nullity theorem is presented.
SFU MATH 232 3.4 Special Subspaces
SFU MATH 232 3.4 Subspaces and Linear Independence
SFU MATH 232 4.1 Determinants and Cofactor Expansion
SFU MATH 232 4.3 Bases and Dimensions
SFU MATH 232 4.5 General Linear Mappings
SFU MATH 232 Sec 4 6 worked examples part 1
SFU MATH 232 3.6 Matrices with Special Forms
SFU MATH 232 3.5 The Geometry of Linear Systems
SFU MATH 232 3.1 Operations on Matrices
SFU MATH 232 4.4 A First Look at Eigenvalues and Eigenvectors
SFU MATH 232 3.3 Geometrical Transformations
SFU MATH 232 7.3 Fundamental Spaces of a Matrix
SFU MATH 232 1.3 Length and Angles in R^2 and R^3
Sec6.3 and 6.4 Matrix inversion and determinants
SFU MATH 232 3.1 Operations on Matrices
SFU MATH 232 7.11 Coordinates with respect to a Basis
SFU MATH 232 6.4 Composition and Invertibility of Linear Transformations
SFU MATH 232 1.3 Vector Equations of Lines and Planes
SFU MATH 232 7.4 The Dimension Theorem and Its Implications
SFU MATH 232 5.1 Determinants in Terms of Cofactors
SFU MATH 232 8.5 Singular Value Decomposition.
SFU MATH 232 2.3 Applications of Linear Systems
SFU MATH 232 3.2 Inverses and Algebraic Properties of Matrices
SFU MATH 232 6.1 Matrices as Transformations
Комментарии
 0:47:55
0:47:55
 1:19:51
1:19:51
 0:41:42
0:41:42
 1:22:46
1:22:46
 0:55:45
0:55:45
 0:44:40
0:44:40
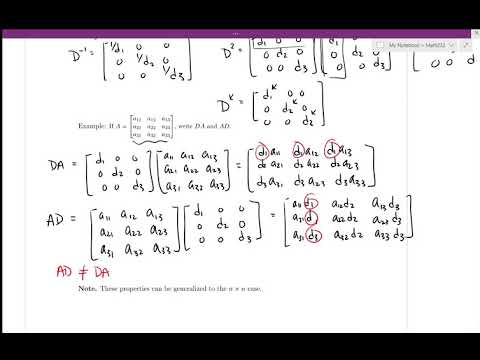 0:44:47
0:44:47
 0:55:06
0:55:06
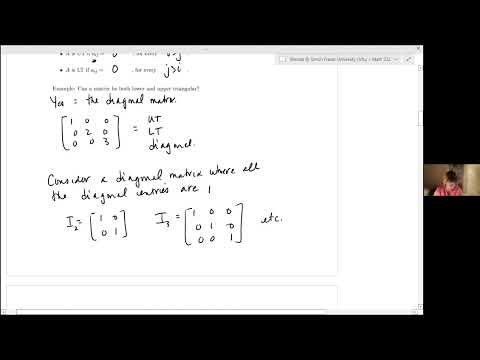 0:57:40
0:57:40
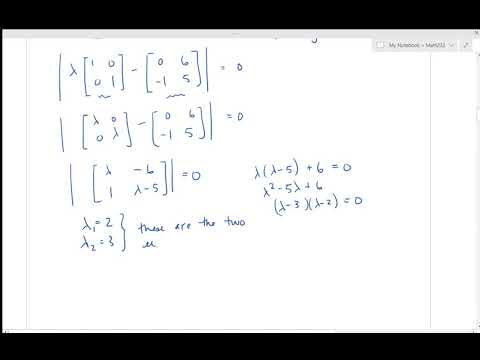 0:56:01
0:56:01
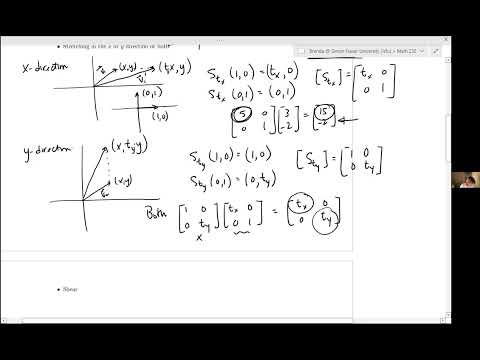 0:45:31
0:45:31
 0:48:59
0:48:59
 0:52:14
0:52:14
 0:37:49
0:37:49
 1:06:44
1:06:44
 0:40:48
0:40:48
 0:35:40
0:35:40
 0:56:43
0:56:43
 0:47:04
0:47:04
 1:08:57
1:08:57
 0:56:45
0:56:45
 0:55:42
0:55:42
 1:09:11
1:09:11
 0:55:34
0:55:34