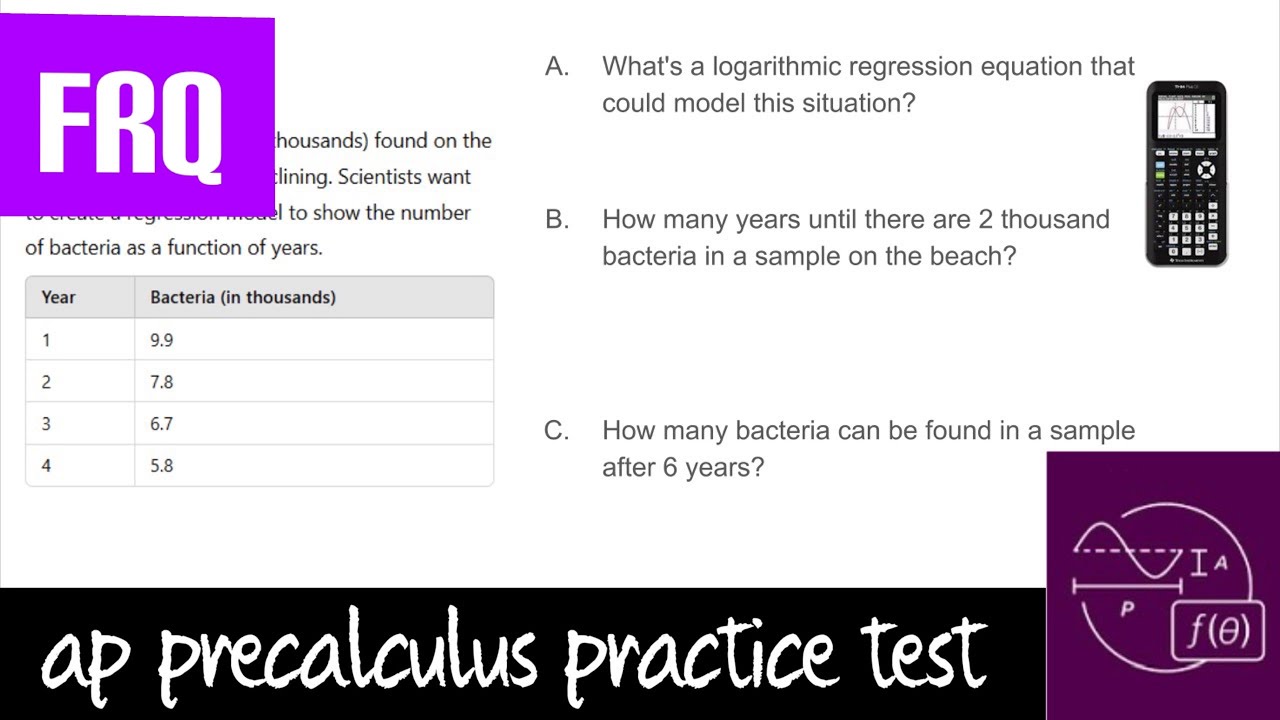
AP Precalculus Practice Test: Unit 2 FRQ #4 TI-84+, Logarithmic Regression, Word Problem

My AP Precalculus Practice Tests are carefully designed to help students build confidence for in-class assessments, support their work on AP Classroom assignments, and thoroughly prepare them for the AP Precalculus exam in May.

Here is a revised version of the response with no inequality symbols:

### AP Precalculus Practice Test: Unit 2 FRQ #4 - TI-84+, Logarithmic Regression, Word Problem

This problem requires you to use a graphing calculator, specifically the TI-84+, to perform logarithmic regression and interpret the results within the context of a word problem.

---

### Problem Overview:

You are typically given a word problem that involves data with a logarithmic trend. Your task is to:

1. **Input the data** into the TI-84+.
2. **Perform logarithmic regression** on the data to find a model that fits the data.
3. **Interpret the regression results**, including the logarithmic function and its meaning in the context of the problem.
4. **Use the model** to make predictions or analyze trends as requested in the word problem.

---

### Steps to Solve:

#### 1. **Input Data into the TI-84+**
- Press `STAT` on the calculator.
- Choose `1: Edit` to enter the data.
- Input the \(x\)-values in `L1` and the corresponding \(y\)-values in `L2`.

#### 2. **Perform Logarithmic Regression**
- After entering the data, press `STAT` again.
- Move over to the `CALC` menu using the arrow keys.
- Select `4: LogReg` (Logarithmic Regression).
- Press `ENTER`. The calculator will perform the regression and provide the regression equation in the form:

\[
y = a \cdot \ln(x) + b
\]

Where:
- \(a\) and \(b\) are the regression constants.
- \(\ln(x)\) represents the natural logarithm of \(x\).

#### 3. **Interpret the Results**
- The calculator will output values for \(a\) and \(b\), which are the coefficients of the logarithmic model. You will need to interpret what these values represent in the context of the problem.
- For example, \(a\) could represent the rate of change, and \(b\) could be the initial value or a baseline measurement.

#### 4. **Use the Model for Predictions**
- Once you have the model, you may be asked to use it to predict a value of \(y\) for a given \(x\), or to interpret what the model implies for large or small values of \(x\).

---

### Example Problem:

**Problem**: A researcher is studying the growth of bacteria over time. The data below shows the number of bacteria \(y\) at various hours \(x\) after the experiment began.

| Hours (x) | Number of Bacteria (y) |
|-----------|------------------------|
| 1 | 100 |
| 2 | 150 |
| 3 | 210 |
| 4 | 270 |
| 5 | 330 |

Find the logarithmic model that best fits the data using logarithmic regression, and then predict the number of bacteria after 6 hours.

---

### Solution Steps:

1. **Enter Data into TI-84+**:
- Press `STAT` `1: Edit`.
- Input the hours into `L1` (1, 2, 3, 4, 5).
- Input the corresponding bacteria counts into `L2` (100, 150, 210, 270, 330).

2. **Perform Logarithmic Regression**:
- Press `STAT` `CALC` `4: LogReg`.
- Press `ENTER` to run the regression.
- The calculator might give the equation:

\[
y = 50 \cdot \ln(x) + 75
\]

This means the number of bacteria increases logarithmically, with a growth rate of 50 per hour and a starting population of 75.

3. **Interpretation of Results**:
- The value \(a = 50\) suggests that the number of bacteria increases by a factor of 50 times the natural logarithm of the time.
- The value \(b = 75\) suggests that the initial number of bacteria at time 0 is 75.

4. **Make Predictions**:
- To predict the number of bacteria after 6 hours, substitute \(x = 6\) into the equation:

\[
y = 50 \cdot \ln(6) + 75
\]

Using a calculator to find \(\ln(6)\), we get:

\[
y \approx 50 \cdot 1.7918 + 75 \approx 89.59 + 75 = 164.59
\]

Thus, after 6 hours, the estimated number of bacteria is approximately 165.

---

### Summary:
- **Logarithmic Regression**: Use the TI-84+ to find the logarithmic regression equation based on the given data.
- **Interpretation**: Understand the meaning of the regression coefficients in the context of the problem.
- **Prediction**: Use the model to make predictions for future values.

I have many informative videos for Pre-Algebra, Algebra 1, Algebra 2, Geometry, Pre-Calculus, and Calculus. Please check it out:

/ nickperich

Nick Perich
Norristown Area High School
Norristown Area School District
Norristown, Pa

#math #algebra #algebra2 #maths #math #shorts #funny #help #onlineclasses #onlinelearning #online #study