Math: Partial Differential Eqn. - Ch.1: Introduction (20 of 42) 1st Order PDE (2 Partial Deriv.)

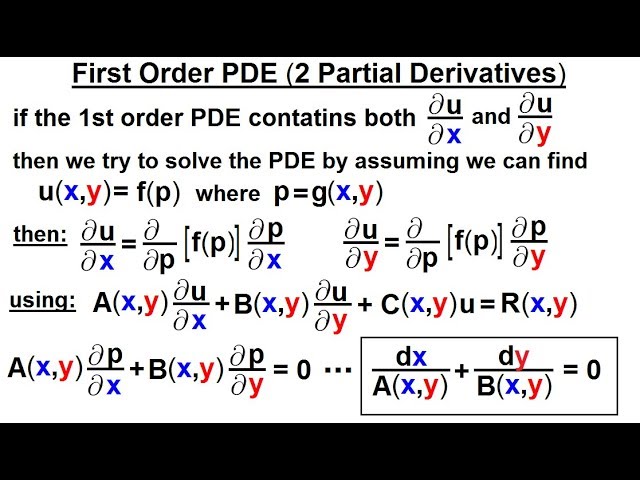
In this video I will explain the steps used to find u=f(x,y)=? if the 1st order partial differential equation, PDE, contain both partial(u)/partial(x)) and partial(u)/partial(y). We have solve the PDE by assuming we can find u(x,y)=f(p) where f(p)=g(x,y) and p is the integrating factor.

Next video in this series can be seen at:

[ronelalday]

This is the one the best playlist I've found in Partial Differential Equations. I highly recommend this to anyone who wants to have a clear introduction (assuming of course that you know ODE already) about the subject.

[sirrenaissance]

Don't get that last step; you're dividing the whole equation or a part of it; you can't just simply cancel if the former; and 0/0 is not a problem; this is the only part of the series which raised a question for me, so pretty amazing explanation.

[vulicuba]

Write two equations in a matrix form. Since the output vector is zero, then the determinant of the matrix must be zero. By calculating the determinant the expression is arrived at with a negative sign between the terms instead of positive sign.

[trickytricks]

Honestly, this doesn't make any sense to me - how was the division executed, and how isn't 0/0 a problem?

[pranavkotteswaran]

It should be dx/A(x, y) - dy/B(x, y) = 0, instead of dx/A(x, y) + dy/B(x, y) = 0

[christopherrosson]

Can someone explain to me how the division of the two different equations works?