filmov
tv
Physics 37 Gauss's Law (15 of 16) Variable Charge Distribution: 'Infinite' Slab
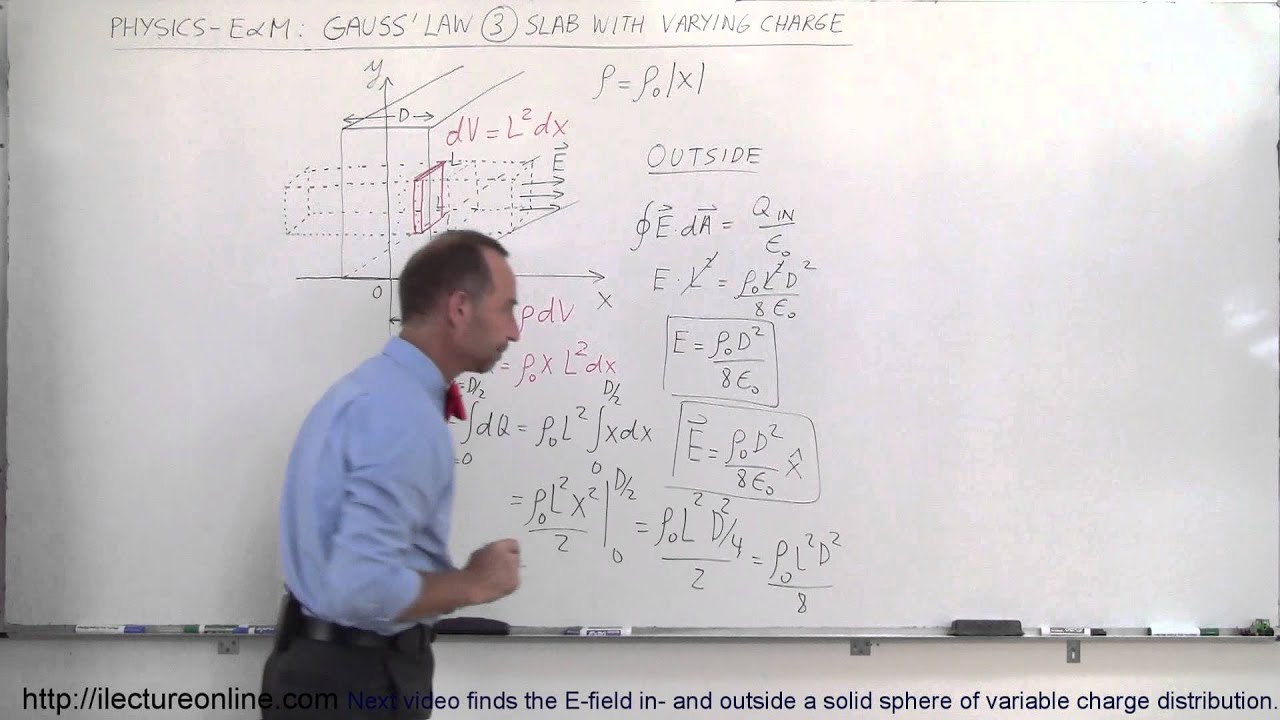
Показать описание
In this video I will find the electric field inside and outside of an infinite slab of variable charge distribution.
Physics 37 Gauss's Law (15 of 16) Variable Charge Distribution: 'Infinite' Slab
Physics 37 Gauss's Law (4 of 16) Electric Field Outside a Conductor
AP Physics C: Electric Force, Field & Gauss’s Law 15: E by Non-Uniform Spherically Symmetric Cha...
Ultimate Gauss' Law review
Physics 37 Gauss's Law (2 of 16) Cylindrical Charge
😁 Playing 🐍Snake🐍 game on calculator 😜 [official video] #shorts #viral #casio
Tough times Never last 😊✌️ #delhipolice #motivation
Most💯 Important Step Before any Procedure 🔥
IIT Bombay CSE 😍 #shorts #iit #iitbombay
UPSC VS IIT JEE 🥵 #iitstatus #motivation #toppers #iitjee #jeemains #upscstatus #neet #nit #jee
Allen🔥 result celebration 🎉🎉 in front of akash institute😡😡 and PW vidhyapeeth😡😡 #allen #pw #akash...
3D: Gauss’ law for infinite slabs
Adda247 Ne PW teachers ko 5 Crore Deya 😱 | Exposed #shorts #physicswallah.
When mathematicians get bored (ep1)
1 15 Application of Gauss Law I Concentric Spheres
Finding Charge on Surfaces of Conductors Using Gauss' Law
#simplification #tricks
NEWYES Calculator VS Casio calculator
Tamasha Dekho 😂 IITian Rocks Relatives Shock 😂😂😂 #JEEShorts #JEE #Shorts
🔥 POV: Integration - Look at me! 👀 💪 | JEE 2024 | Math | Bhoomika Ma'am
Ladies Compartment in Delhi Metro.(1)
Electric flux, derivation of Gauss' Law and using Gauss' Law to find electric field.
Lec 15 Applications of Gauss Law
Gauss's Law
Комментарии
 0:09:53
0:09:53
 0:09:26
0:09:26
 0:11:04
0:11:04
 0:28:05
0:28:05
 0:10:58
0:10:58
 0:00:47
0:00:47
 0:00:22
0:00:22
 0:00:16
0:00:16
 0:00:11
0:00:11
 0:00:14
0:00:14
 0:00:15
0:00:15
 0:14:44
0:14:44
 0:00:36
0:00:36
 0:00:37
0:00:37
 0:08:28
0:08:28
 0:15:16
0:15:16
 0:00:11
0:00:11
 0:00:14
0:00:14
 0:00:13
0:00:13
 0:00:48
0:00:48
 0:00:32
0:00:32
 0:15:25
0:15:25
 0:07:37
0:07:37
 0:06:47
0:06:47