filmov
tv
Algebraic geometry 1 Introduction

Показать описание
This lecture is part of an online algebraic geometry course (Berkeley math 256A fall 2020), based on chapter I of "Algebraic geometry" by Hartshorne. The full set of lectures is in the playlist "Algebraic geometry I: varieties".
(The course continues in the playlist "Algebraic geometry II: schemes" based on chapter II.)
The first lecture describes a birational map from a circle to a line.
(The course continues in the playlist "Algebraic geometry II: schemes" based on chapter II.)
The first lecture describes a birational map from a circle to a line.
Algebraic geometry 1 Introduction
Introduction to Tropical Algebraic Geometry (1 of 5)
What is algebraic geometry?
Introduction to Geometry
Algebraic Geometry (MTH-AG) Lecture 1
Introduction To Algebraic Geometry - Course Introduction
Learn Pre Algebra and Algebra 1
All Of Algebra 1 Explained In 5 Minutes
Lecture 18, History of Math, Princeton University, Prof. Kontorovich, 11/19/2024
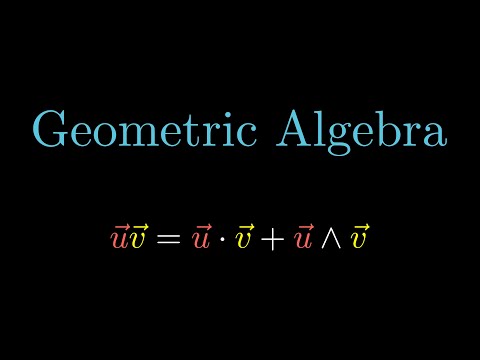
A Swift Introduction to Geometric Algebra
algebraic geometry and Galois theory 1
Algebra For Beginners - Basic Introduction
Studying Algebraic Geometry (A Dream)
Fastest Geometry Summary
Algebra Basics: What Is Algebra? - Math Antics
Algebra 1 Basics for Beginners
What is...algebraic geometry?
Introduction to Algebraic Geometry (Yang Zhang): Lecture 1/3
Elliptic Curves - Lecture 3 - An introduction to Algebraic Geometry (part 1)
What’s the area?
This is Pure Mathematics
Every Type of Math Explained in 9 Minutes.
Algebraic Topology by Allen Hatcher #shorts
Algebra for Beginners | Basics of Algebra
Комментарии
 0:20:58
0:20:58
 0:58:09
0:58:09
 0:11:50
0:11:50
 0:34:03
0:34:03
 1:34:04
1:34:04
 0:12:41
0:12:41
 0:00:33
0:00:33
 0:05:00
0:05:00
 1:14:18
1:14:18
 0:44:23
0:44:23
 1:25:36
1:25:36
 0:59:07
0:59:07
 0:04:35
0:04:35
 0:02:52
0:02:52
 0:12:07
0:12:07
 0:23:05
0:23:05
 0:17:21
0:17:21
 1:30:37
1:30:37
 0:27:11
0:27:11
 0:00:42
0:00:42
 0:00:16
0:00:16
 0:08:50
0:08:50
 0:00:44
0:00:44
 0:37:09
0:37:09