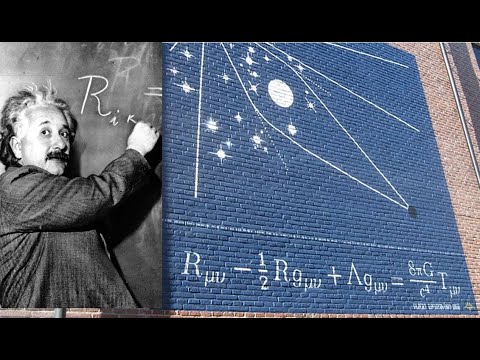
13. The Einstein field equation (variant derivation).

MIT 8.962 General Relativity, Spring 2020
Instructor: Scott Hughes

A second route to the Einstein field equation, using a variational principle.  (Note, this lecture is due for an overhaul; we were unable to do so as planned in Spring 2020 due to the outbreak of the COVID-19 pandemic.)

License: Creative Commons BY-NC-SA

[bishalbanjara]

Simply wow! the most lucid, easy and effective way procedure than a lot of boring textbooks!!

[kunjalvara]

Way of explaining is amazing ☺️. Thank you for these lacture

[MH-mcpp]

The f(R) gravity is literally identical to adding a scalar \phi. So his first two examples are just special cases of the third. If you want to add a scalar, add a scalar. Don't bury it inside of f(R); it adds nothing. This Carrol et al idea is devoid of any substance.

[ekaingarmendia]

I think there is a mistake in the derivation of Klein-Gordon. When considering the Minkowski metric (-1, 1, 1, 1) as defined in lecture 2 around minute 25, the d'Alembert operator should be *minus* the contraction of the derivatives with the metric, so when you write the second part of Euler-Lagrange it should be just d'Alembert operating on the field, *not* minus d'Alembert. Then the signs match with KG.
As it is written now, the solutions are unstable because they blow up at some suffiently distant region, which I guess we do not want. Please correct me if I'm mistaken.

[irinacrouse]

Barry quit cyber squatting out here I beat Einstein you are out here on my feed uninvited guess

[ericsu]

The energy tensor of vacuum in Schwarzschild manifold is not always zero.
Detail in "81 Contracted Bianchi Identities and Energy Tensor" on the website,