filmov
tv
Area bounded between sec(x) and tan(x)+1 (area between functions using symmetry vs. brute force)

Показать описание
00:00 Introduction: we calculate the area between functions using symmetry vs. brute force methods, and this time the use of symmetry saves well over three quarters of the work! We begin with the symmetry trick for integrating the area, then we use the traditional area integral method. Finally, we will show the two results are equivalent, but the symmetry method for integration is definitely the superior answer to the problem.
00:50 Symmetry approach to the area integral: shift the interval and use the even and odd symmetry to simplify the area bounded between even and odd functions. We recognize that sec(x) is an even function (symmetry about the y-axis) and tan(x)+1 is *almost* an odd function. We decide to shift the x axis up to y=1, and this does not change the area between the two curves! However, this changes the two functions to sec(x)-1 and tan(x), and these functions are even and odd functions, respectively. The shift in origin exposes a critical symmetry of the functions, allowing us to reduce the area between functions to a single trivial integral of the tangent function, and we find the area between the two curves immediately.
04:29 Traditional approach part 1: split the integration interval and compute the left and right area between functions using brute force. To find the geometric area between two functions, we split the integration interval at the intersection of the two functions. We do this because this is the location where the function on top reverses, and that's important for defining the height of an area increment so all the area between the functions counts as positive. Next, we set up the left area integral by visualizing a small increment of area that we call dA. On the left side of the integration interval, dA is given by (f(x)-g(x))dx, so we integrate sec(x)-(tan(x)+1) on negative pi/4 to zero. This requires finding antiderivatives of sec(x) and tan(x), applying the limits of integration, and simplifying as much as possible using properties of the natural log function.
07:28 Traditional approach part 2: integrate the right side of the area bounded between the functions. Next we integrate the right side of the desired area, and now dA=(g(x)-f(x))dx. So we integrate tan(x)+1-sec(x) and simplify as far as possible. Now we can add the left and right areas to obtain the area bounded between sec(x) and tan(x)+1.
09:45 Show the two answers are equivalent: now we show the two results for the area bounded between sec(x) and tan(x)+1 are equivalent by using log properties to simplify the result obtained by the brute force method. In particular, we find ln(sqrt(2)+1)+ln(sqrt(2)-1) and we recognize two conjugate binomials in the logarithms. We use log properties to write as a single logarithm and we multiply to obtain the difference of two squares: one. Using the fact that the natural log of 1 is zero, this term vanishes and leaves us with the same geometric area bounded between functions: ln(2).
00:50 Symmetry approach to the area integral: shift the interval and use the even and odd symmetry to simplify the area bounded between even and odd functions. We recognize that sec(x) is an even function (symmetry about the y-axis) and tan(x)+1 is *almost* an odd function. We decide to shift the x axis up to y=1, and this does not change the area between the two curves! However, this changes the two functions to sec(x)-1 and tan(x), and these functions are even and odd functions, respectively. The shift in origin exposes a critical symmetry of the functions, allowing us to reduce the area between functions to a single trivial integral of the tangent function, and we find the area between the two curves immediately.
04:29 Traditional approach part 1: split the integration interval and compute the left and right area between functions using brute force. To find the geometric area between two functions, we split the integration interval at the intersection of the two functions. We do this because this is the location where the function on top reverses, and that's important for defining the height of an area increment so all the area between the functions counts as positive. Next, we set up the left area integral by visualizing a small increment of area that we call dA. On the left side of the integration interval, dA is given by (f(x)-g(x))dx, so we integrate sec(x)-(tan(x)+1) on negative pi/4 to zero. This requires finding antiderivatives of sec(x) and tan(x), applying the limits of integration, and simplifying as much as possible using properties of the natural log function.
07:28 Traditional approach part 2: integrate the right side of the area bounded between the functions. Next we integrate the right side of the desired area, and now dA=(g(x)-f(x))dx. So we integrate tan(x)+1-sec(x) and simplify as far as possible. Now we can add the left and right areas to obtain the area bounded between sec(x) and tan(x)+1.
09:45 Show the two answers are equivalent: now we show the two results for the area bounded between sec(x) and tan(x)+1 are equivalent by using log properties to simplify the result obtained by the brute force method. In particular, we find ln(sqrt(2)+1)+ln(sqrt(2)-1) and we recognize two conjugate binomials in the logarithms. We use log properties to write as a single logarithm and we multiply to obtain the difference of two squares: one. Using the fact that the natural log of 1 is zero, this term vanishes and leaves us with the same geometric area bounded between functions: ln(2).
 0:12:00
0:12:00
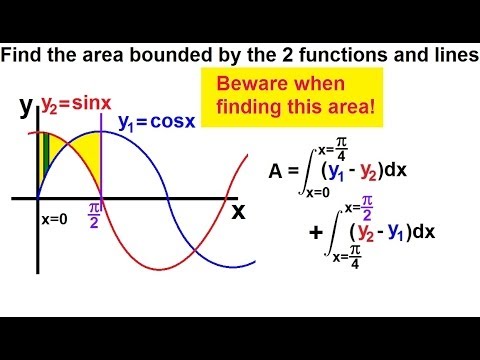 0:08:43
0:08:43
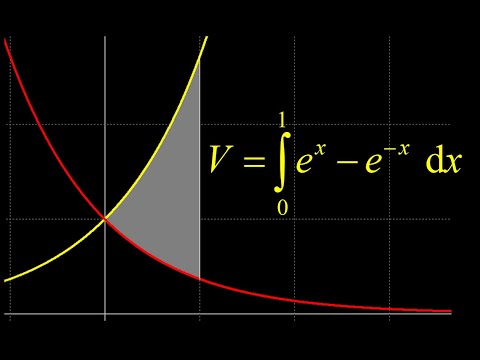 0:03:03
0:03:03
 0:04:15
0:04:15
 0:04:42
0:04:42
 0:03:39
0:03:39
 0:03:56
0:03:56
 0:07:57
0:07:57
 0:02:47
0:02:47
 0:00:35
0:00:35
 0:05:03
0:05:03
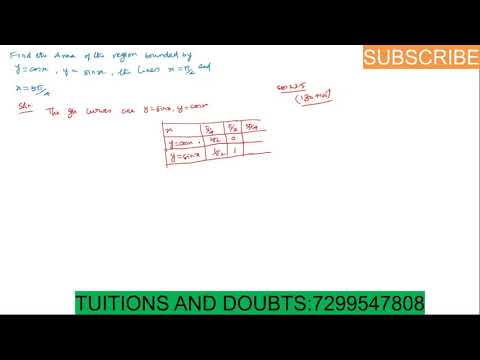 0:08:31
0:08:31
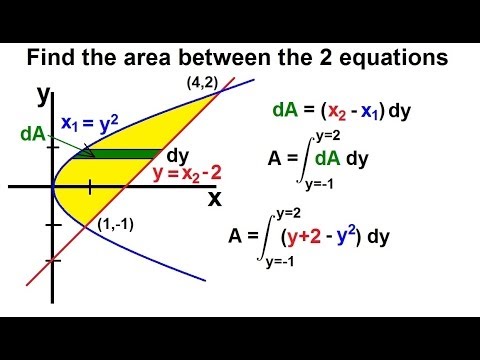 0:08:14
0:08:14
 0:08:10
0:08:10
 0:00:16
0:00:16
 0:05:59
0:05:59
 0:00:15
0:00:15
 0:04:44
0:04:44
 0:14:39
0:14:39
 0:03:58
0:03:58
 0:11:16
0:11:16
 0:00:11
0:00:11
 0:04:54
0:04:54
 0:01:15
0:01:15