filmov
tv
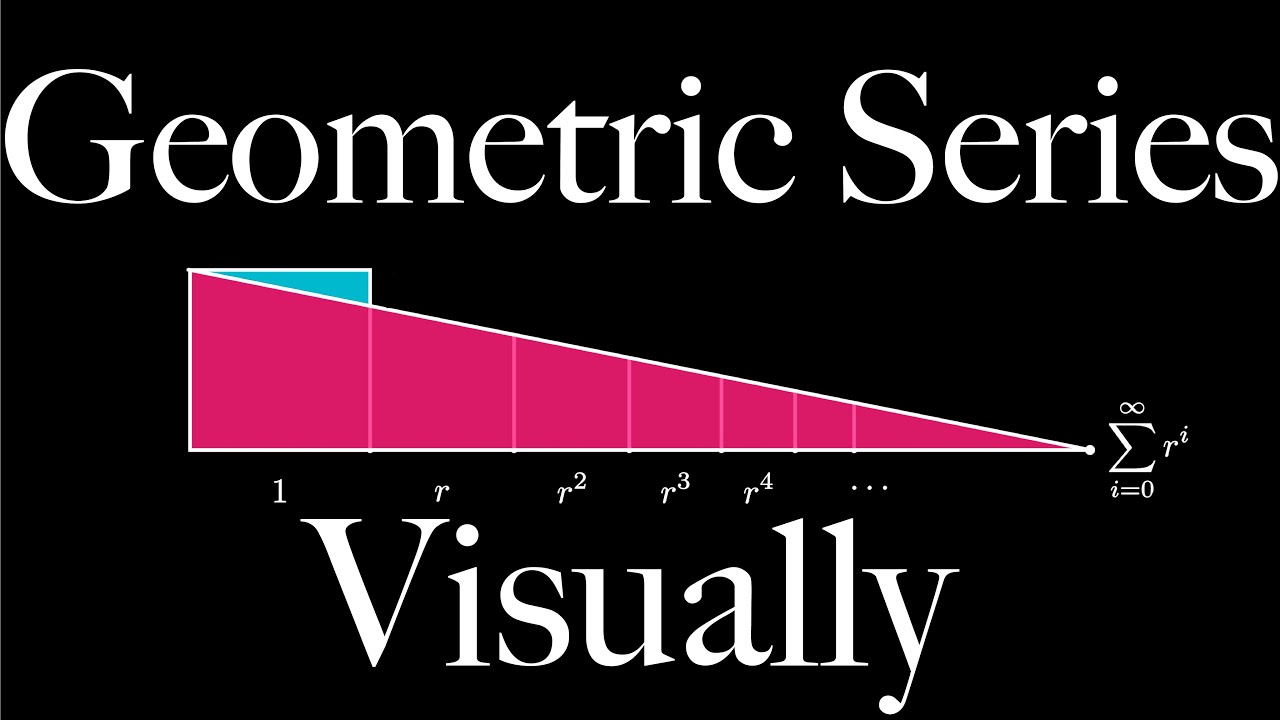
Visual proof of infinite geometric series sum
Показать описание
This is a short, animated visual proof demonstrating the infinite geometric series formula for any positive ratio r with r less than 1. This series is important for many results in calculus, discrete mathematics, and combinatorics. #mathshorts #mathvideo #math #calculus #mtbos #manim #animation #theorem #pww #proofwithoutwords #visualproof #proof #iteachmath #geometricsums #series #infinitesums #infiniteseries #geometric #geometricseries
If you like this content, please consider subscribing.
To learn more about animating with manim, check out:
______________________________
Music in this video:
Addicted by VYEN
If you like this content, please consider subscribing.
To learn more about animating with manim, check out:
______________________________
Music in this video:
Addicted by VYEN
Visual proof of infinite geometric series sum
Visual proof of infinite geometric series sum II
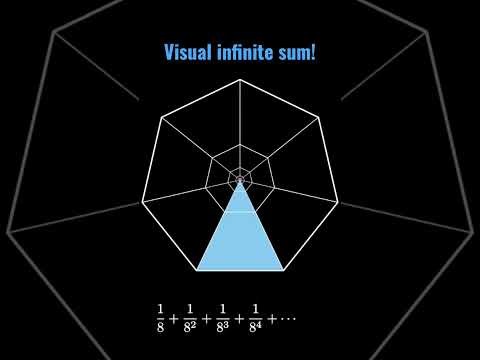
A visual infinite sum like you’ve never seen!
Beautiful Geometry behind Geometric Series (8 dissection visual proofs without words) #math #series
Infinite Geometric Series in a Square (visual proof without words) III
What's this infinite sum?
Infinite Geometric Series Sum
Geometric series: sums of powers of 8 (visual proof)
Two Nonstandard Infinite Geometric Series (visual proof)
A Golden Ratio Infinite Series Dissection (visual proof)
The Geometry of Finite Geometric Sums (visual proof; series)
Summing powers of 1/8 visually!
Geometry behind the geometric sum of powers of 1/3!
Infinite Sums | Geometric Series | Explained Visually
Infinite Sum of Powers of -1/7 in a Square (visual proof without words)
Five Classic Proofs without Words
Visual Harmonic Series Divergence from Bernoulli!
Infinite Geometric Series Sum Formula III (visual proof)
Infinitely Many Infinite Sums Geometrically (visual proof)
Infinite sum of powers of 4/9
Geometric series: sums of powers of 9 are Triangular (visual proof)
Alternating Geometric Series: Powers of -1/3 (visual proof)
Geometric Series: sum of powers of 1/8 (visual proof)
Two Infinite Series Sums from Regular Polygons (visual proof)
Комментарии
 0:01:41
0:01:41
 0:01:41
0:01:41
 0:00:57
0:00:57
 0:06:46
0:06:46
 0:01:58
0:01:58
 0:00:31
0:00:31
 0:01:00
0:01:00
 0:01:48
0:01:48
 0:03:21
0:03:21
 0:03:13
0:03:13
 0:01:53
0:01:53
 0:00:53
0:00:53
 0:01:00
0:01:00
 0:02:47
0:02:47
 0:01:48
0:01:48
 0:03:25
0:03:25
 0:01:00
0:01:00
 0:01:49
0:01:49
 0:04:10
0:04:10
 0:00:52
0:00:52
 0:01:48
0:01:48
 0:01:43
0:01:43
 0:01:13
0:01:13
 0:03:35
0:03:35