A Criterion for Positive Definiteness of a Symmetric Matrix

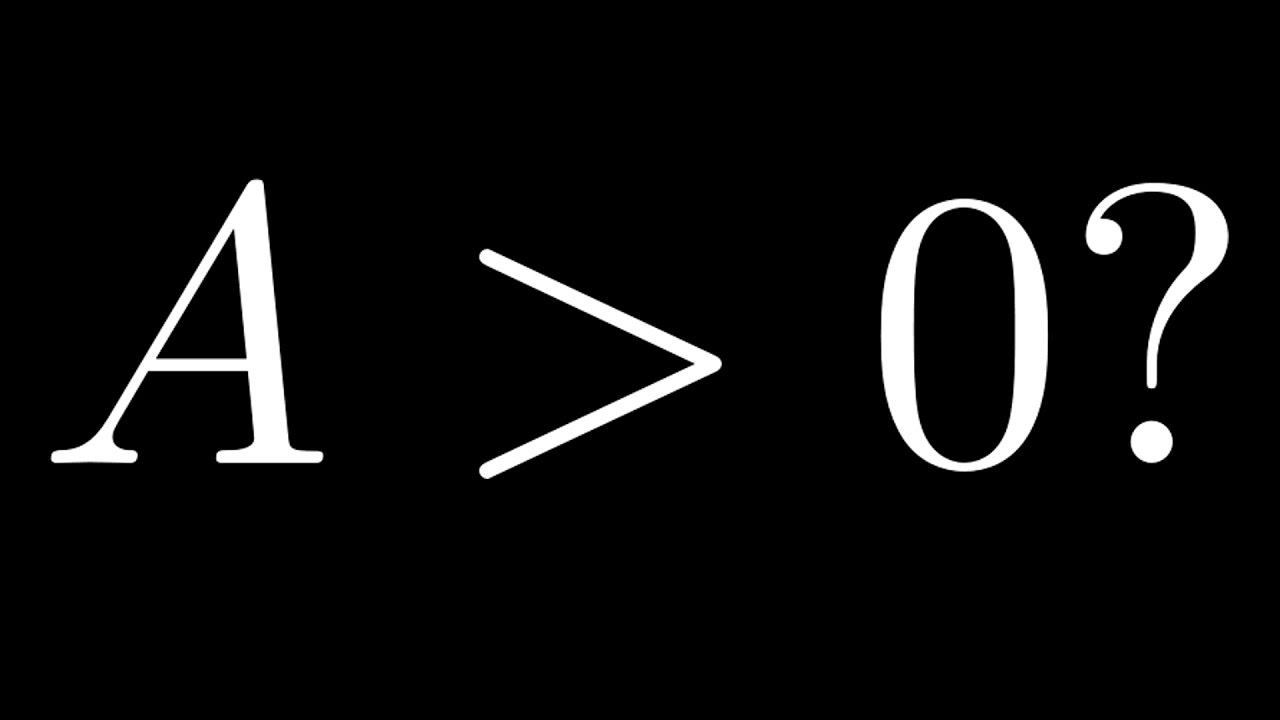
Показать описание

Рекомендации по теме

Комментарии

The necessity for having positive definiteness comes the need decompose A into a product A=M^T * M so se could do using Dirac’ notation <x | A | x > = <x*M | M*x> = <u, v> and have valid inner products in the new vectors u and v. In other words, matrix A must be Gramian. Thanks for teaching us this!

jaimelima

you again?? got to subscribe. Short and sweet explanations

ahming

Is it true positive definiteness should be a term used only for symmetric (quadratic) matrices? And this does not apply to non-symmetric matrices?

jeremykua

For the matrix at 10:15 wouldn't picking one of the roots of the polynomial suffice to disprove posirive definiteness? for example if instead of (-3, 1) we chose (-4, 1) the result would be zero but (-4, 1) is not the zero vector therefore the matrix isn't positive definite.

ennevudoppioo

But here you have assumed y=1. Isn't there a possibility for inner product to be negative for other y value (say y = -16)?

studentcommenter

For the 2x2 matrix A = [a, b;b, c], is it also enough to say det([c]) > 0 and det(A) > 0 if and only if A is positive definite?

Does this slightly different check for positive definiteness generalize in a nice way for larger matrices so we don't have to choose sub-matrices growing from the top left?

menturinai

at 7:40, how does he go straight to the answer without first multiplying the matrix with [a, 1] then the result with [a, 1]?

wolftribe

0:25 "When you're down to one vector -AYYYY"

emilnordstedsivertsen

And we have an information slice, the point at which information begins to multiply.
Well this is so, not especially really thinking, just what would be added to the trend of volotility.

ares

omg, please replace my teacher, i beg u

fakemail

BRO, IT TOOK YOU 16 MINUTES TO DO GRADE 1 MATH?

LithiumReaper

A Criterion for Positive Definiteness of a Symmetric Matrix

A Criterion for Positive Definiteness of a Symmetric Matrix

Sylvester's Criterion For Positive Definite Matrices Explained with Worked Example

How to Prove that a Matrix is Positive Definite

Checking if a Matrix is Positive Definite

Advanced Linear Algebra - Lecture 31: Sylvester's Criterion for Positive Definiteness

5. Positive Definite and Semidefinite Matrices

MA9714: 34 - Criteria for Definiteness

Positive Definite Matrices and Minima

Symmetric Matrices and Positive Definiteness

Prove sylvester criterion for positive definite matrix

Positive Definite Matrices

If A is positive Definite, the A is singular.

Orthogonal, Orthonormal, Positive, and Positive Semi Definite Matrices, Sylvester's Criterion

For a Positive Definite M, Is BᵀMB Always Positive Definite, Too?

EM 23b - Definiteness of a quadratic form and a matrix - Sylvester's criterion

Positive-Definite Matrix

Theoretical Test on Positive Definite Matrices. Energy-based definition of Positive Definite Matrix.

Problem on Positive definiteness||Sylvester's Condition

Positive Definite Matrices. Its testing using the determinants of all top left corner matrices.

Is BᵀB Always Positive Definite? (Also, Messi makes a comeback!)

Vector Calculus 14: The Dot Product Matrix Is Positive Definite

05.4.1 Hermitian Positive Definite matrices

Positive Definite Matrices Video Lecture

Advanced Linear Algebra 20: Positive Definite & Positive Semidefinite Matrices