Real Analysis | A convergent sequence is bounded.

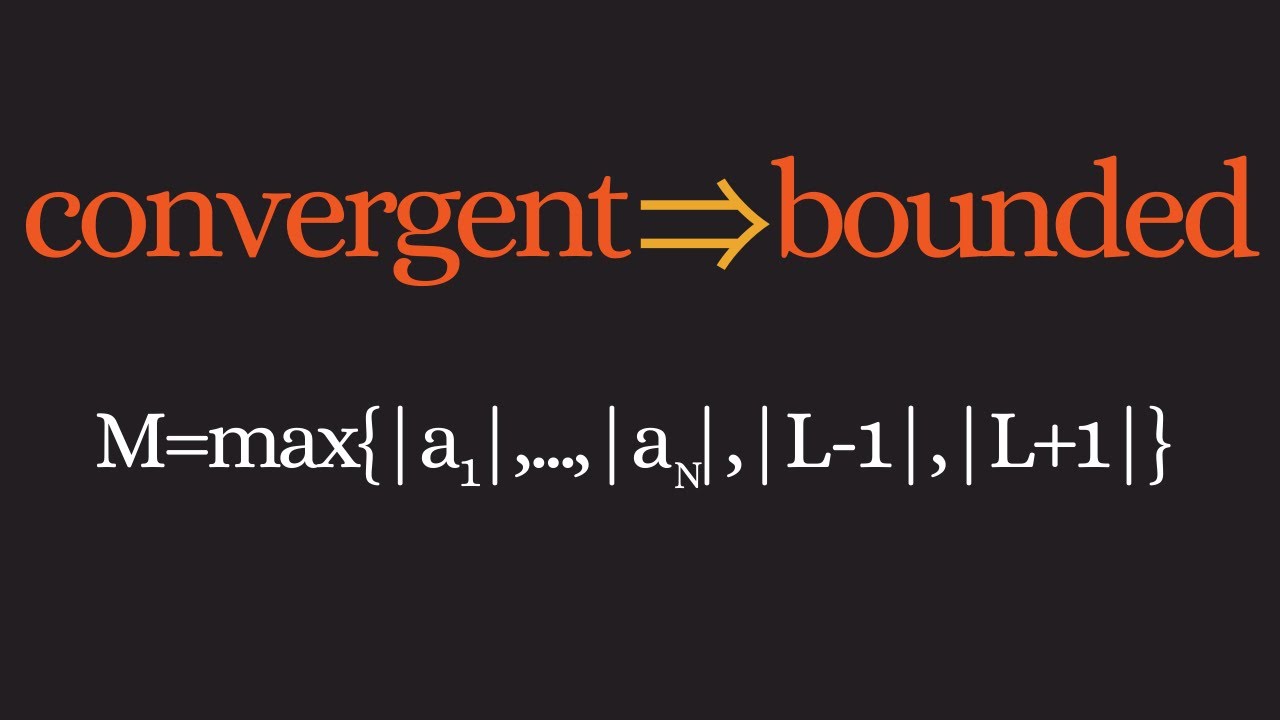
Показать описание

We define the notion of a bounded sequence and prove that every convergent sequence is bounded.

If you are going to use an ad-blocker, considering using brave and tipping me BAT!

Books I like:

Abstract Algebra:

Differential Forms:

Number Theory:

Analysis:

Calculus:

Рекомендации по теме

Комментарии

I really love how topics on this channel are in range from competitive mathematics and puzzles to academic mathematics, rigorous stuff, proofs and such. I was searching for something like that on YT ^^

zaratustra

Hey, I’d love to see a video on Fubini’s theorem. In many videos we just blindly use it to interchange the order of integration, but I think it would be cool to look at the exact statement and maybe some details

YaStasDavydov

"And that's a good place"

AKhoja

i'd love a video on the jacobi theta functions. You're one of the best teachers on youtube, at least for me, that i've come across with.

panagiotisapostolidis

Please do more real analysis videos, your demonstrations provide clarity for self-learners like me. From now on when I see a video that you post for real analysis I will like and comment. I'm currently self-studying Tao's Analysis I. In CH 5, section 6, he defines exponentiation with supremum. I think it'd be helpful if you could explain your perspective on this section, because it isn't clear to me how to think about the section such that I'm able to do proofs. Thanks for your content!

brandonread

Let a_n be a convergent sequence and let lim n →∞ a_n = L. Take ε = 1, then there is N ∈ ℕ such that |a_n - L| < 1 for all n > N. From the reverse triangle inequality ( | |A| - |B| | ≤ |A - B| ), we can show that | |a_n| - |L| | ≤ |a_n - L| < 1 which implies |a_n| < |L| + 1. Now define M = max{|L| + 1, |a_1|, |a_2|, ..., |a_N|}. Therefore we have 0 ≤ |a_n| ≤ M for all n ∈ ℕ.

heewahhin

The sequence (a_n) converges to a limit L if we can find an index N such that for n > N, or n >= N (depending on your book), the sequence terms permanently stay within epsilon distance of L.
Said slightly different, a sequence (a_n) converges to L if for any epsilon no matter how small, the sequence terms eventually stay within epsilon distance of L.

maxpercer

I think you are missing a ‘there exists’ before the n>N part of your negative example at the end. The negation of ‘if P then Q’ is ‘there exists a P such that not Q’ (i.e. a counterexample). Your statement as written sounds like you mean that none of the a_n get close to a proposed L when you really mean that there is always at least one more a_n that is separated from L no matter how far along the sequence you get.

stephenbeck

OMG. That negation statement is quite a Mindfull

debendragurung

I love your number theory lectures it is better than any other channel I don't know why your subscribers are low people are not smart enough to understand these things

adarshyadav

Thank you so much! This is a great video, really really helped me a lot

barendbadenhorst

Damn this guy makes it look easy, thanks sir for this video

thehym

Could you make a video based on Galois's theory, or his cohomology?

wafizariar

Why is |an| < |L-1|. Surely after N sufficiently large, it is above |l-1|

dadrunkgamer_

@Michael Penn
3:12 There might be an error here - as a counter-example take *a_n = -1 - 1/n* with the limit *L = -1* . We get

    *| a_n | = 1 + 1/n > | L + 1 | = 0 (Contradiction to last line of **3:12** !)*

Similarly, *a_n = 1 + 1/n* with the limit *L = 1* contradicts the second inequality

   *| a_n | = 1 + 1/n > | L - 1| = 0 (Contradiction to last line of **3:12** !)*

*Rem.:*
I'd say there are two possible changes to correct the proof: Either change "and" to "or" in that line starting at 2:53 (that is where I felt the proof was going, and using the maximum on the next screen seems to confirm that guess) or use the inverted form of the triangle inequality to get a different upper bound

    *| x | - | y | <= | | x | - | y | | <= | x - y | for all x, y in R ==> 0 <= | a_n | <= | L | + 1 for n >= N*

P.S.: I really appreciate you take the time and include the corner case *a_n = (-1) ^ n* at the end - such examples always helped to remind me why we need certain assumptions in proofs in the first place.

carstenmeyer