Quantum Chemistry 7.3 - Hydrogen Atom Radial Wavefunctions

Short lecture on hydrogen atom radial wavefunctions.

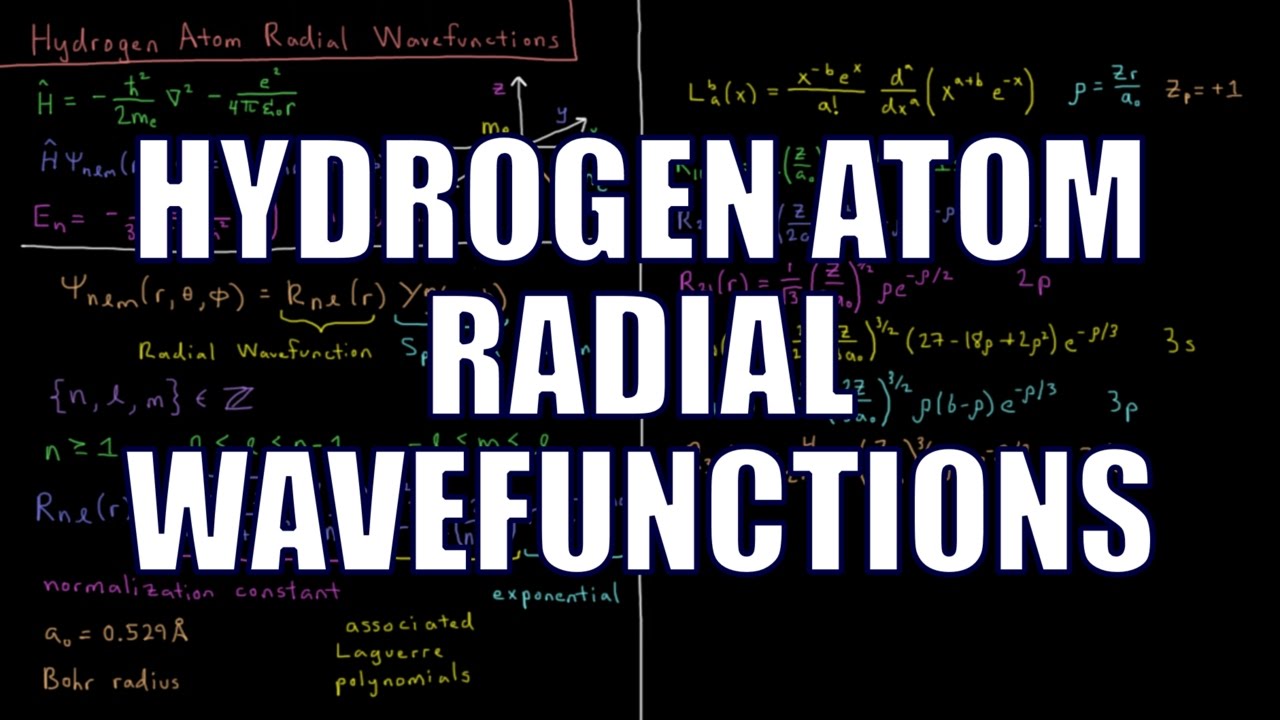
The wavefunctions of the hydrogen atom quantum mechanical model system can be separated into functions of the spherical polar coordinates r, theta, and phi. The functions of r are called the radial wavefunctions. The hydrogen radial wavefunctions are composed of a normalization constant, the associated Laguerre polynomials, and a decaying exponential of r. The inputs to each function depend on the quantum number n, l and m, as well as the Bohr radius a_o. The Laguerre polynomials can be produced from a generating formula or looked up in a table.

--- About TMP Chem ---

All TMP Chem content is free for everyone, everywhere, and created independently by Trent Parker.

--- Video Links ---

--- Social Links ---

--- Equipment ---

Microphone: Blue Yeti USB Microphone

Drawing Tablet: Wacom Intuos Pen and Touch Small

Drawing Program: Autodesk Sketchbook Express

Screen Capture: Corel Visual Studio Pro X8

[TMPChem]

Notes and Corrections:

0:01 The range of allowed values of quantum number l (left side, middle, green) should go from 0 to n-1, not 0 to n as written. Expression should read [ 0 <= l <= n - 1 ].

[gagepreston]

I must say, your notes are a life saver. Thank you so much for this resource!

[arsalaraichand]

Your videos are so helpful! God bless you! You have made my life so much easier especially since physical chemistry has been giving me such a hard time!

[xccsys_err]

Using these to review some of the key concepts before Finals. I hope you're having a fantastic day!

[gregoryb]

Great video! Btw, I think the normalization constant is [(n+l)! ]^3 not [(n+1)!]^3

[dutonic]

Frustrated. This is one of my first quantum classes and I can see that angular momentum and whatever m represents are quantized but I don't know why or where it came from...

[pasikein]

Thanks for the well structured videos. One note, should l be 0 ≤ l ≤ n-1, not 0 ≤ l ≤ n as it is written in your slide?

[omarfarukh]

Sir, please explain to me why the quantum spin number is expressed as 1/2 and -1/2

[laurendanielle]

I’m confused on how z and rho go into the the radial wavefunction. Can you please explain this to me?

[zahirmuhammad]

i saw 100 vadeo no body touch Laguerre polynomial

[neilsucks]

Hi,

Can you explain what you meant (t= 8:00) when you said the n=2 functions decay faster? Shouldn't e^(-rho) decay faster than e^(-rho/2)?

Thank you for your fantastic work.

[SampleroftheMultiverse]

This video shows under the right conditions, the quantization of a field is naturally produced.

The ground state energy is induced via Euler’s contain column analysis. The containment of the column must come in to play before over buckling occur or the effect will not work.

The system response in a quantized manor when force is applied in the perpendicular direction. Bonding at the points of highest probabilities and maximum duration( peeks and troughs) of the fields/sheet produced a stable structure out of three fields

People say I am just plucked guitar strings. I said you can not make structures with vibrating guitar strings or harmonic oscillators.


In the model, “U” shape waves are produced as the loading increases and just before the wave-like function shifts to the next higher energy level.

Over-lapping all the waves frequencies together using Fournier Transforms, I understand makes a “U” shape or square wave form.

If this model has merit, seeing the sawtooth load verse deflection graph produced could give some real insight in what happened during the quantum jumps.

You can reproduce my results using a sheet of Mylar* ( the clear plastic found in school folders.

[urty]

Can you show the way to obtain the normalization constant