filmov
tv
FIT4.3.1. Galois Group of Order 8

Показать описание
Field Theory: Let K be Q[sqrt(2), sqrt(3), sqrt(5)], the splitting field of f(x) = (x^2-2)(x^2-3)(x^2-5) over Q. Find the Galois group of K over Q, find all subgroups of the Galois group, and find all subfields of K over Q.
FIT4.3.1. Galois Group of Order 8
FIT4.3. Galois Correspondence 1 - Examples
FIT4.1. Galois Group of a Polynomial
FIT4.3.2. Example of Galois Group over Finite Field
Galois Group of Cyclotomic Polynomial
Galois Theory by Emil Artin #shorts
Example Galois Group Primitive Root
Galois Extensions: Finding the Subgroup Lattice of the Galois Group
Galois Group of Quartic Polynomial which is Dihedral
The Galois Group of the Composite of Galois Extensions (Algebra 3: Lecture 24 Video 1)
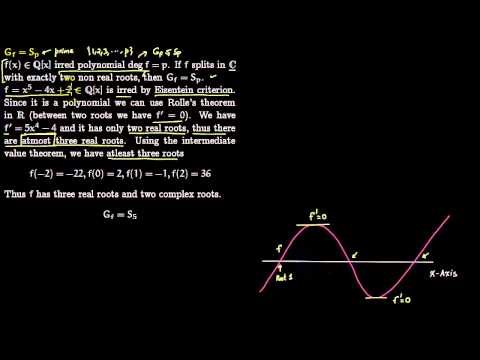
When is Galois Group S_p?
Fields: Galois Group of a Quadratic Extension
Galois Group of Cubic Polynomial which is Symmetric
Abstract Algebra II: Galois groups of polynomials order 2 and 3, cubic formula, 2-18-22 part 1
When is Galois group A n
Galois group of x^3-3x+3
Abstract Algebra, Lec 38: Galois Group Example, Grad School Topics, Review for Final Exam
Galois Extensions: Finding the Galois Group
GaloisTheoryIV
Galois Extensions: An Example of Finding a Galois Group
Galois Group of Quintic Polynomial which is Symmetric
Fields: Galois Group of a Double Quadratic Extension
Galois Theory Lecture 18: Galois Group of Irreducible Cubic Polynomials
Abstract Algebra II: Galois group of polynomial, 3-2-18
Комментарии
 0:10:50
0:10:50
 0:19:35
0:19:35
 0:22:51
0:22:51
 0:06:10
0:06:10
 0:08:52
0:08:52
 0:00:23
0:00:23
 0:08:52
0:08:52
 0:02:42
0:02:42
 0:24:22
0:24:22
 0:11:26
0:11:26
 0:08:47
0:08:47
 0:05:30
0:05:30
 0:20:47
0:20:47
 0:59:51
0:59:51
 0:15:21
0:15:21
 0:05:35
0:05:35
 1:05:24
1:05:24
 0:05:14
0:05:14
 0:13:59
0:13:59
 0:08:41
0:08:41
 0:09:11
0:09:11
 0:04:06
0:04:06
 0:19:03
0:19:03
 0:52:56
0:52:56