filmov
tv
Vectors and the Geometry of Space: The Dot Product
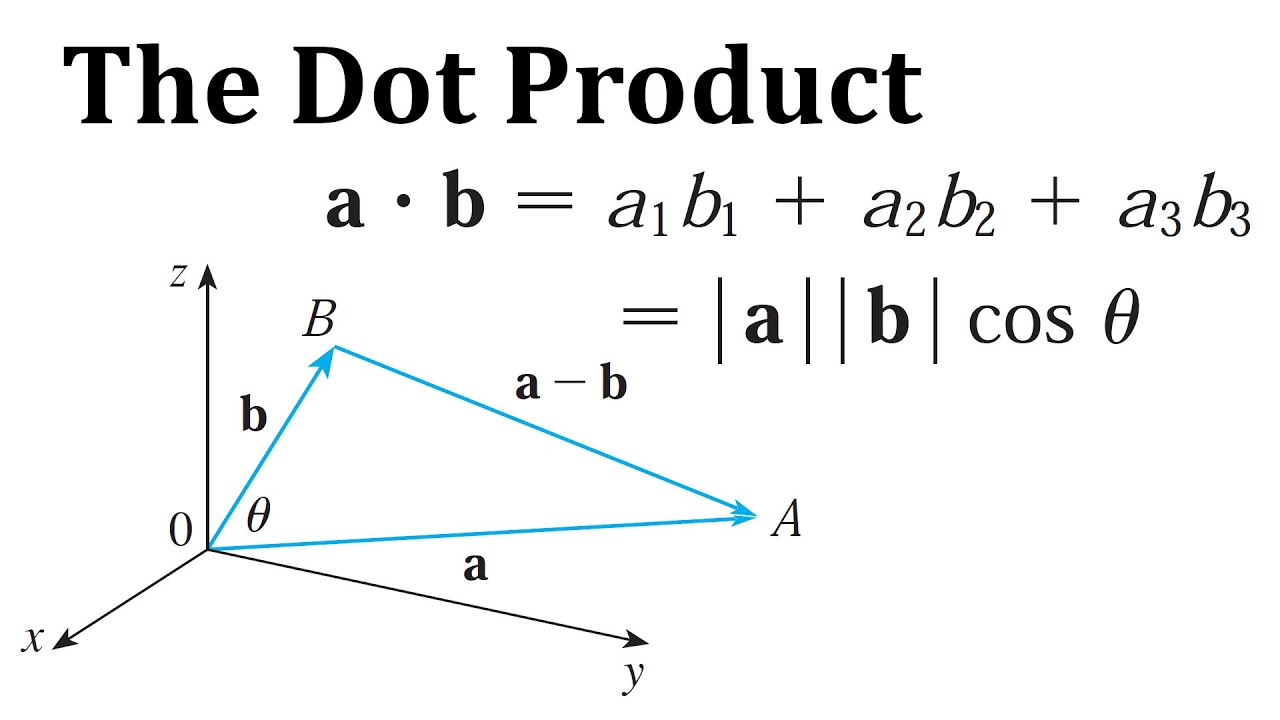
Показать описание
In this video I go over further into vectors and this time look at multiplying vectors with the dot product. In my previous videos I discussed adding vectors and multiplying vectors with a scalar. This time I discuss multiplication of two vectors via the dot product, which is defined as multiplying all corresponding vector components and adding them up, thus giving a scalar or real number value; aka not a vector. Further in the video I show that this way of defining vector multiplication has useful applications since it can be reinterpreted in terms of the angle between 2 vectors. I also discuss vector projections, direction cosines, and applications to physics of the dot product.
The topics covered as well as their timestamps are listed below.
- Introduction: 0:00
- Sections in Calculus Book Chapter: 1:03
- Topics to Cover: 1:46
1. The Dot Product: 2:52
- Definition 1: Dot Product: 3:38
- Example 1: 6:15
- Properties of the Dot Product: 10:49
- Proof of Property 1: 12:42
- Proof of Property 2: 14:00
- Proof of Property 3: 15:59
- Proof of Property 4: 19:15
- Proof of Property 5: 24:00
- Geometric Interpretation of the Dot Product: 24:45
- Definition 2: Dot Product: 28:02
- Example 2: 43:14
- Corollary: 45:46
- Example 3: 46:49
- Perpendicular Vectors: 52:42
- Example 4: 54:47
2. Vector Angles: 56:49
- Direction Angles and Direction Cosines: 1:02:55
- Example 5: 1:17:28
3. Projections: Vector and Scalar Projections: 1:21:27
- Summary of Projections: 1:30:22
- Example 6: 1:33:19
4. Vectors in Physics: 1:39:04
- Example 7: 1:42:33
- Example 8: 1:45:25
Related Videos:
------------------------------------------------------
The topics covered as well as their timestamps are listed below.
- Introduction: 0:00
- Sections in Calculus Book Chapter: 1:03
- Topics to Cover: 1:46
1. The Dot Product: 2:52
- Definition 1: Dot Product: 3:38
- Example 1: 6:15
- Properties of the Dot Product: 10:49
- Proof of Property 1: 12:42
- Proof of Property 2: 14:00
- Proof of Property 3: 15:59
- Proof of Property 4: 19:15
- Proof of Property 5: 24:00
- Geometric Interpretation of the Dot Product: 24:45
- Definition 2: Dot Product: 28:02
- Example 2: 43:14
- Corollary: 45:46
- Example 3: 46:49
- Perpendicular Vectors: 52:42
- Example 4: 54:47
2. Vector Angles: 56:49
- Direction Angles and Direction Cosines: 1:02:55
- Example 5: 1:17:28
3. Projections: Vector and Scalar Projections: 1:21:27
- Summary of Projections: 1:30:22
- Example 6: 1:33:19
4. Vectors in Physics: 1:39:04
- Example 7: 1:42:33
- Example 8: 1:45:25
Related Videos:
------------------------------------------------------
Комментарии
 0:04:41
0:04:41
 0:17:42
0:17:42
 0:09:52
0:09:52
 0:18:47
0:18:47
 0:10:17
0:10:17
 0:57:54
0:57:54
 0:05:59
0:05:59
 0:11:37
0:11:37
 0:00:15
0:00:15
 0:32:25
0:32:25
 4:12:18
4:12:18
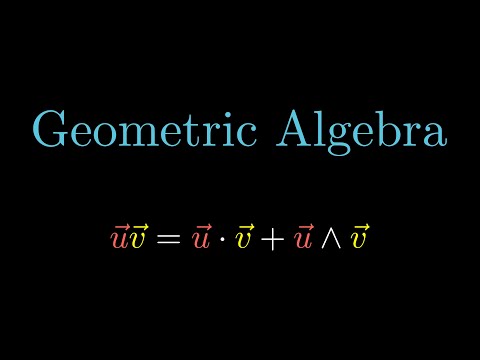 0:44:23
0:44:23
 0:12:29
0:12:29
 0:13:54
0:13:54
 0:27:08
0:27:08
 0:21:25
0:21:25
 0:10:36
0:10:36
 0:20:33
0:20:33
 0:04:28
0:04:28
 0:34:03
0:34:03
 0:38:35
0:38:35
 0:31:10
0:31:10
 0:10:27
0:10:27
 0:07:27
0:07:27