Diff Eqs #22, Zero as an Eigenvalue, Bifucations of Linear Systems, Trace-Determinant Plane

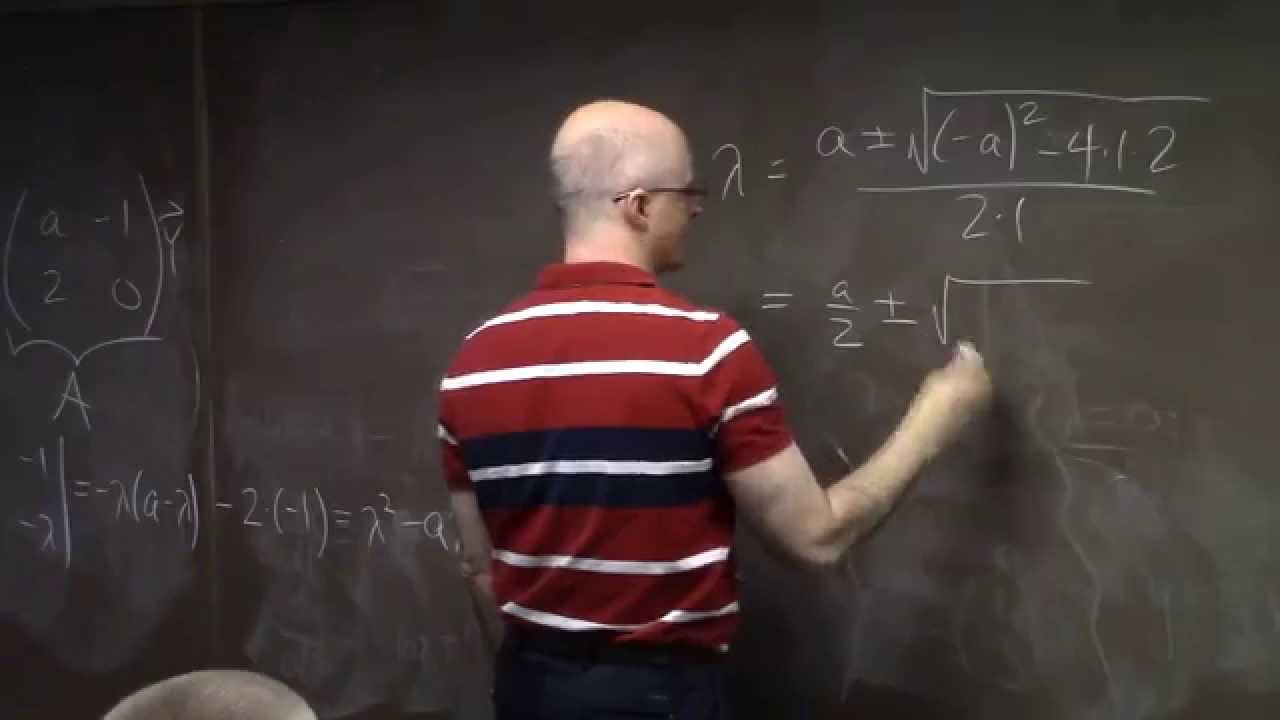
Показать описание

Differential Equations, Lecture 22. (0:00) Comments about Exam 2. (0:45) Summary of the class. (1:37) An example where zero is a (non-repeated) eigenvalue. (3:06) There are infinitely many equilibrium points (along a line through the origin) since det(A)=0. (5:49) Find the eigenvalues, eigenvectors, and the general solution. (14:50) Bifurcations in a one-parameter family of linear systems of differential equations. (16:14) Find the eigenvalues and classify the behavior of the system based on their values (real or complex? both positive? both negative? opposite signs?) (27:34) Visualize the bifurcations with Manipulate and StreamPlot. (31:05) The trace-determinant plane and its critical loci. (38:00) Draw the TD-plane and its critical loci. (46:10) Draw the parametric curve (T(a),D(a)) in the TD-plane and note the bifurcation values as those values of "a" where the critical loci are crossed.

AMAZON ASSOCIATE
As an Amazon Associate I earn from qualifying purchases.

Рекомендации по теме

Комментарии

Actually if t is time and hence t>=0, then k2 is involved in placing a bound on x and y:

If k2>=0:

x>=3*k1 + k2
y<=2*k1 - 3*k2

swastiktiwari

About content at 14:18 (y, x) = k1*(3, 2)+k2*exp(11t)*(1, -3) = (3*k1+k2*exp(11t), 2*k1-3*k2*exp(11t))
So,
x = 3*k1+k2*exp(11t)
y = 2*k1-3*k2*exp(11t)

That means 3x + y = 11*k1

So, is my understanding correct that:

1) On x-y plane the solution depends only on k1 and not k2 ?
2) Solutions are just parallel lines with slope -3 and intercept dependent only on k1 ?

swastiktiwari

Diff Eqs #22, Zero as an Eigenvalue, Bifucations of Linear Systems, Trace-Determinant Plane

Diff Eqs #22, Zero as an Eigenvalue, Bifucations of Linear Systems, Trace-Determinant Plane

The Big Theorem of Differential Equations: Existence & Uniqueness

How to Solve Constant Coefficient Homogeneous Differential Equations

Differential Equations: The Language of Change

Physics Students Need to Know These 5 Methods for Differential Equations

First Order Linear Differential Equations

5 simple unsolvable equations

Separable First Order Differential Equations - Basic Introduction

non-diagonalizable systems -- differential equations 22

Differential Equations: Lecture 1.1-1.2 Definitions and Terminology and Initial Value Problems

Slope Fields | Calculus

Differential Equations - 22 - Why superimpose? (y=c1y1+c2y2)

Order And Degree Of Differential Equations | Differential Equation Class 12

Differential Eqns. F22-03 -- Differential equations from calculus

Using Laplace Transforms to solve Differential Equations ***full example***

Differential Eqns. F22-16 -- Elimination method for systems of equations

Differential Eqns. F22-06 -- Exact ODEs + Substitution

First order, Ordinary Differential Equations.

High-Order Ordinary Differential Equations with More Derivatives (from Physics)

Differential Equations Exam 1 Review Problems and Solutions

Differential Equations: Method of Undetermined Coefficients - Annihilator Approach

Differential Equations: Lecture 2.5 Solutions by Substitutions

Autonomous Systems and Phase Line Diagrams - Ordinary Differential Equations | Lecture 7

Cauchy - Euler Equations and Variation of Parameters Problem 4 (Differential Equations)

Using Laplace Transforms to solve Differential Equations full example