filmov
tv
Differential Equations - 22 - Why superimpose? (y=c1y1+c2y2)

Показать описание
Answering a question that was submitted to me: why do we take our solution to be linear combinations of the two solutions associated with our two roots?!
Solving Differential Equations with a Composition (Obvious) Substitution (Differential Equations 22)
Differential Equations: The Language of Change
Differential Equations - 22 - Why superimpose? (y=c1y1+c2y2)
22. Partial Differential Equations 1
🔵22 - Method of Variation of Parameters 1 - Non-Homogeneous Differential Equations
Solve the given differential equation by separation of variables
First order, Ordinary Differential Equations.
First Order Linear Differential Equation, 2 3#22
DIFFERENTIAL EQUATIONS WITH VARIABLE SEPARABLE||EXERCISE-9.4||Q.No.- (20 to 23)||CHAPTER-9||NCERT
Solution Exercise 2.5 Q.21,22.differential equation by D G zill.Berniulli problem
Differential Equations - Full Review Course | Online Crash Course
22 | DU | Compartmental Model | Differential Equation
Ex 2.2: Qs 11 - 22 - First-Order Differential Equations | Dennis G. Zill | Solutions | The Study Pod
Differential Equation - 2nd Order (22 of 54) Constant Coeff. w/ Complex Roots: Example 1
What are differential equations?
Ordinary Differential Equations -22 Particular Integral : Rule 4
Order And Degree Of Differential Equations | Differential Equation Class 12
Differential Equations || Lec 66 || Ex: 5.1: Q 17 - 22 || Free Damped Motion, Spring Mass System
Exercise 2.4 || Question 22 || Differential Equations
DIFFERENTIAL EQUATION BY D.G.ZILL.CHAP#2 EXE#2.2 Q(21 TO 28)
Variation of Parameters Revisited - Ordinary Differential Equations | Lecture 22
Eigenvalues Problems in PDEs - Partial Differential Equations | Lecture 22
Formation of PARTIAL DIFFERENTIAL EQUATIONS | Problem 22 | By Eliminating Arbitrary Constants
Differential Equations || Lec 37 || Ex: 4.3 Q15 - 22 || Higher Order ODEs with Constant Coefficients
Комментарии
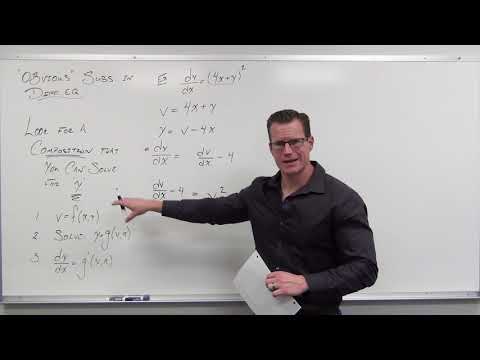 0:40:03
0:40:03
 0:23:24
0:23:24
 0:09:21
0:09:21
 0:49:34
0:49:34
 0:14:07
0:14:07
 0:07:47
0:07:47
 0:48:35
0:48:35
 0:05:54
0:05:54
 0:39:36
0:39:36
 0:14:53
0:14:53
 9:59:49
9:59:49
 0:05:08
0:05:08
 0:13:12
0:13:12
 0:02:42
0:02:42
 0:05:35
0:05:35
 0:04:06
0:04:06
 0:01:55
0:01:55
 0:35:38
0:35:38
 0:05:30
0:05:30
 0:24:16
0:24:16
 0:25:32
0:25:32
 0:15:40
0:15:40
 0:05:40
0:05:40
 0:14:10
0:14:10