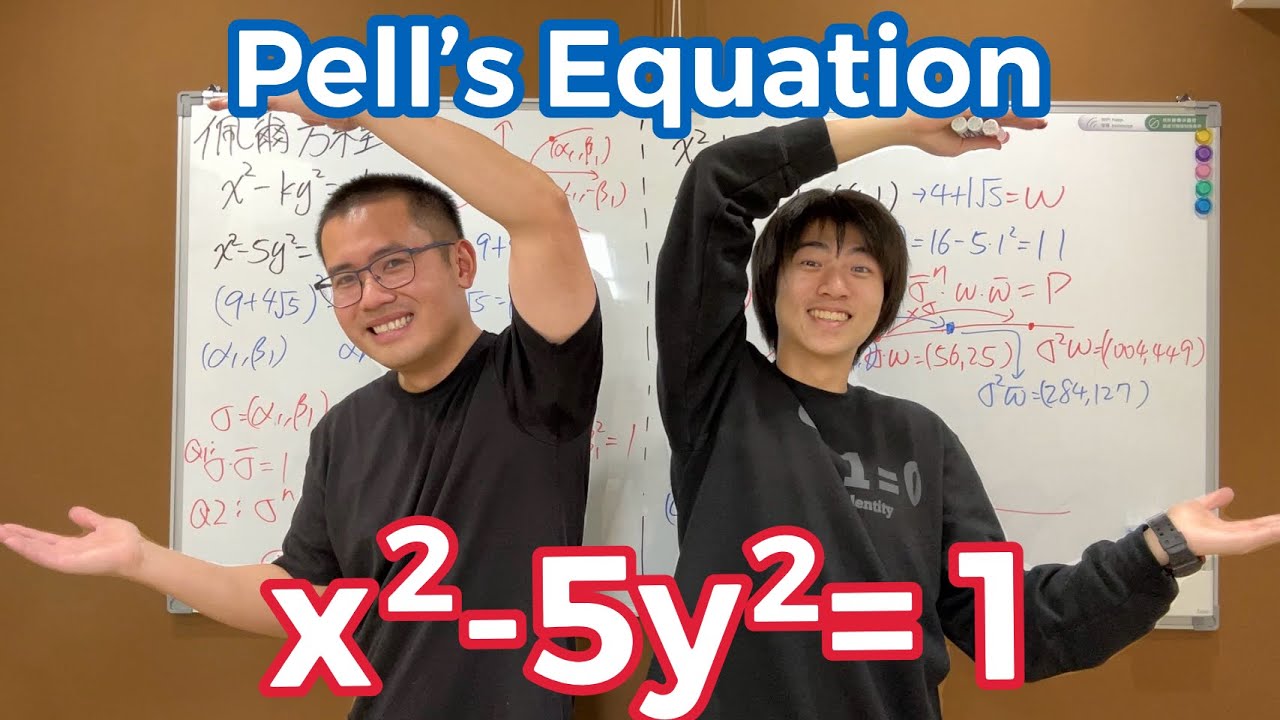
A general strategy for solving Pell's equations (ft. @octopuskeng )

How can we solve Pell's equations, i.e. equations in the form of x^2-ky^2=p? Today we will learn a general strategy of finding all integer solutions to x^2-5y^2=1 and x^2-5y^2=11. Big thanks to @octopuskeng for being a guest on my channel.

By the way, he did this math research and gave a talk with his middle school teacher in 9th grade. However there were no live audience when he gave this talk due to the pandamic. So we recorded a video to share with everyone!

----------------------------------------
Big thanks to my Patrons for the full-marathon support!
Ben D, Grant S, Mark M, Phillippe S. Michael Z, Jan P. Devun C. Stefan C. Ethan BW Didion S. NN Minkyu Y Brandon F Levon M shortsleeve Jack P Gabriel G Yeeted C David H Mateo F Emma M Eden E Minicat Jose R Nicholas Fey Tyler B Lucas W Ahmet Ö An R Camila L Luupo Farrah Jordan T Batman 1127 Mathguru Дмитрий П

----------------------------------------
#blackpenredpen #math #calculus #apcalculus

[blackpenredpen]

What are the tens and the units digits of 7^7^7?

[amansparekh]

What this links to is units in the ring Z[rootk]. Every unit has norm 1 and thus is a solution to the equation. Clearly units form a multiplicative group.

[randomjin]

But now the most important part: how do you find that "first solution" - and more importantly, enure that it is the first (i.e. smallest)? Guess and check sounds very involved for equations with non-trivial coefficients, e.g. x²-73y² = 1217

[HarunaTukur-zbkq]

Nice to meet you ❤ Tomorrow morning I have mathematics exam wish me success

[ssgamer]

That notebook was the least asian thing in the video.I can confirm that every competitive exam aspirant prefers longbooks(400pgs+) for exam prep because it is easier to maintain and search old things.

[paultijink]

Great! Please do make that video showing there are no other solutions!

[asifulalamc]

Amazing video! Happy new year to everyone. 🎉🎆

[Budgeman]

I may not understand the math completely but the video is amazing to watch

[devrimturker]

Log Ladders, Lemmermeyer's Product

[Happy_Abe]

Wouldn’t negating x values also give solutions so we also have all the reflected values

[Nikkikkikkiz]

In my classes, we solve this by having the solution be a continued fraction and just write answer in continued fraction format

[GreenMeansGOF]

I want to see the proof! Also, can this be extended to when the right hand side is composite?

[Blaqjaqshellaq]

Squaring 9+4*5^(1/2) gives the pair {161, 72}. (I was waiting for you to give that pair, but I had to figure it out myself.)
You can also use this method to give rational approximations of the square root of 5: 9/4, then 160/72=20/9...

[khoozu]

The pell equation x^2-2y^2=+-1 gives approximation of square root 2

[johns.]

OK, now how about doing this for x^2 - 61y^2 = 1 ?

[trelligan]

Sorry, I really need comments for this gentleman.

[johns.]

And for that matter, is there a simple way of finding all integer solutions for elliptic curves of the form y^2 = x^3 - x + r^2 ?

[SDCORL]

Secant, tangent/k are all of the solutions.

[OubleJum]

I dont understand anything, I just watch these to fall asleep

[Maths]

If "f" is a differentiable function on the interval [0, 1], with the following boundary conditions:

f(0) = 0

f(1) = 1


Then, find the minimum value of the integral:
∫ from 0 to 1 of (f'(x))² dx.