Potential Functions, Fundamental Theorem of Calculus Applied to Line Integrals, & Path Independence

Показать описание

In this video we continue our discussion on line integrals by introducing the concept of potential functions which can be used to derive vector fields/functions. This allows us to apply the fundamental theorem of calculus to line integrals. We show that in this case, the line integral is independent of path (AKA the vector field is conservative).

Topics and timestamps:
0:00 – Introduction
0:35 – Potential functions
3:41 – Fundamental theorem of calculus applied to line integrals
13:15 – Example
29:49 – Conservative vector fields, relation to curl

#Calculus

Рекомендации по теме

Комментарии

AE 501: Thanks for a very clear and helpful review video of path independence!

ahmedashmaig

AE501-Johnny Riggi: Very insightful video Professor. Have not heard of this concept as it relates to vector fields in mathematics. Very powerful tool!

giovanniriggi

AE501: Great video! I watched it back and forth with the curl video and the end of the curl video makes more sense to me now. Thanks!

Julia_Westfall

AE501: This video is packed with information. Following along with the provided lecture notes really helped me get the concepts down.

KarlaPkva

AE 501: I do not remember doing much with curl in undergrad, but this was a great explanation of the topic!

carlydunford

AE501: Thanks again for another great review! On a very minor side note, this video was only playing audio in my right headphone until the end of the section on potential functions (3:41).

Evan.M.Phillips

AE 501: Great video thank you Professor!

Tamanaaaa

AE501: I had originally only learned about divergence and curl through E&M physics. Seeing them through a plain mathematical perspective helped my understanding. Thanks!

KennethWright-kh

AE 501: Potential Functions are extremely interesting. I will definitely look up applicable examples of potential functions in my desired field.

keyshawnb

AE 501: Thanks for this helpful video professor

AurashFilsoof

AE501: Very helpful video on potential functions

BennettBoyd-hf

AE501: Great video, was very helpful on the homework

paxtonschipper

[AE501] I hadn't heard of potential functions before so it was new to me

TriMartz-fx

AE501: I prefer using the brute force method but I can see where the potential function method can be beneficial/easier method. But this was very helpful with the homework!

EfremNickel

ae501 following from previous video Caleb T

calebtuw

AE501: I find the Brute Force method easier to use, but the Potential Function method is fundamentally more interesting!

ethanngo

AE501: The brute force method seems to be more intuitive to me. But I bet the potential function probably saves on computational power when it comes to control systems.

jacobgivens

AE501: Interesting lecture. It seems like the Example of F(x, y) = <3 + 2 x y, x^2 - 3 y^2> is an Exact, Conservative, and irrotational vector since the curl(F) = 0

Chuan-YuTsai

AE501: I feel like is an application of optimization here. Finding the path with the least/most work could be useful for optimizing some system moving through space.

HJ

Nice, video sir ، but if y relate the math with real life use it will be very nice to understand

eng-vfgh

Potential Functions, Fundamental Theorem of Calculus Applied to Line Integrals, & Path Independence

Finding the scalar potential function for a conservative vector field // Vector Calculus

Conservative Vector Fields // Vector Calculus

Potential Functions, Fundamental Theorem of Calculus Applied to Line Integrals, & Path Independe...

The Fundamental Theorem of Line Integrals // Big Idea & Proof // Vector Calculus

Determining the Potential Function of a Conservative Vector Field

Finding a Potential for a Conservative Vector Field

How to Test if a Vector Field is Conservative // Vector Calculus

Multivariable Calculus: Fundamental Theorem of Line Integrals - Potential Functions (16.3)

Project Presentation | Tate Allen | Minimizing the standard Ring Axioms

How to use the fundamental theorem of line integrals when you have a conservative vector field.

Find Potential Function for Conservative Vector Field

potential function of the conservative vector field to evaluate a line integral (KristaKingMath)

Path Independence, Conservative VFs, Potential Functions, & Fundamental Theorem of Line Integral...

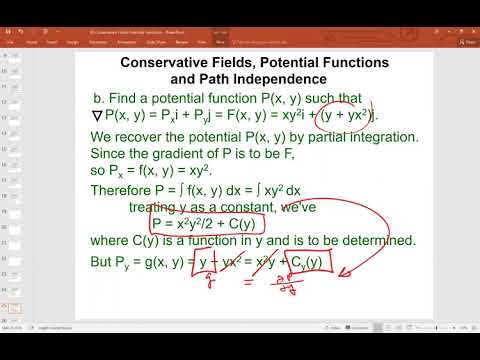
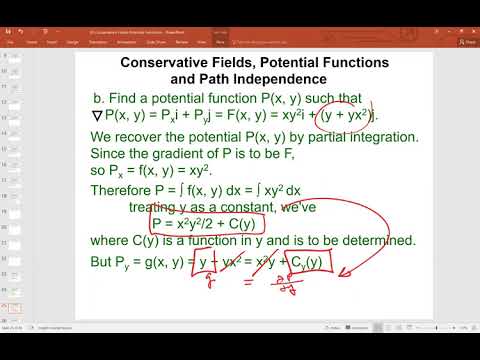
28 Conservative Fields, Potential Functions and The Fundamental Theorem of Line Integral

Path Independence, Conservative Fields, & Potential Functions

13.3 (8) The Fundamental Theorem of Line Integrals - Example #2: Finding the Potential (Part 1)

16.3: The FundamentalTheorem for Line Integrals

The Fundamental Theorem of Calculus

Real Analysis Lecture 61 Part 2: Existence of potential functions

potential function of a conservative vector field (KristaKingMath)

Fundamental Theorem for Line Integrals :: Conservative Vector Field Line Integral

30 Conservative Fields, Potential Functions and The Fundamental Theorem of Line Integral

Real Analysis Lecture 61 Part 1: The fundamental theorem of calculus for line integrals

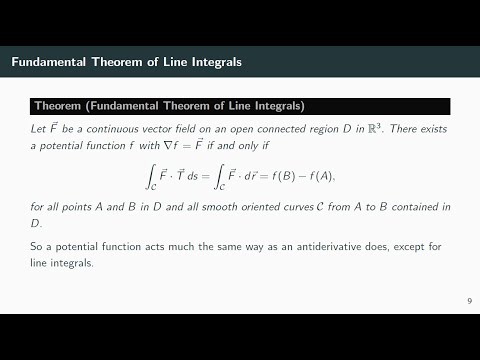
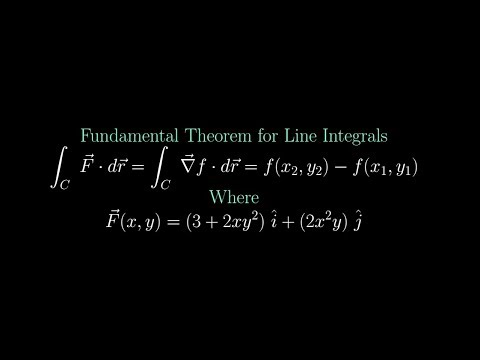
Fundamental Theorem of Line Integrals