filmov
tv
Problems in Real Analysis | Ep. 2
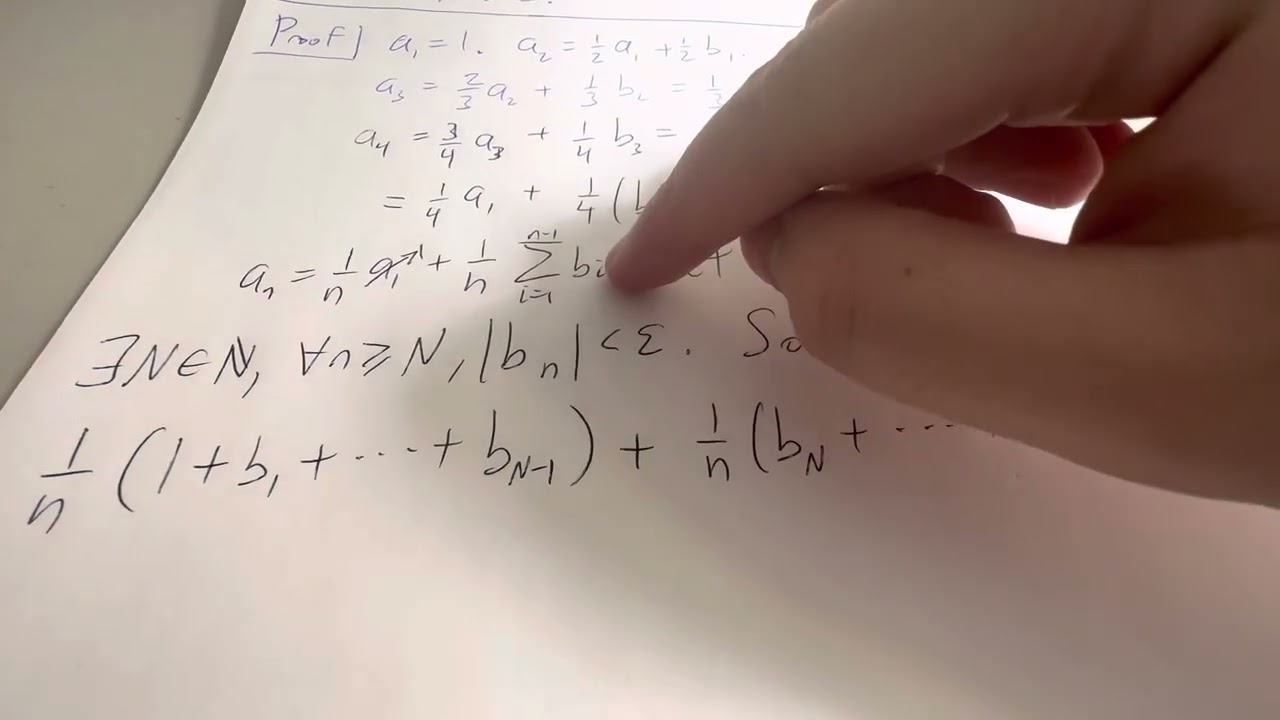
Показать описание
Real Analysis Exam 1 Review Problems and Solutions
Problems in Real Analysis | Ep. 1
Problems in Real Analysis | Ep. 2
10,000 Problems in Analysis
The Real Analysis Survival Guide
Why study real analysis?
Problems in Real Analysis | Ep. 3
Problems in Real Analysis | Ep. 6
NBHM 2024 | Question 6 Solution – Differentiability of a Function
Problems in Real Analysis | Ep. 4
Problems in Real Analysis | Ep. 7
Problems on Real Analysis(Chidume) || Real Number System || Part 1
How to Understand Analysis
Problems in Real Analysis | Ep. 10
Problems in Mathematics: Real Analysis-1
Problems in Real Analysis | Ep. 5
Real Analysis Exam 2 Review Problems and Solutions
Problems in Real Analysis-1
Real Analysis Live - Problem Solving - Continuous Functions (Problems here: https://tbsom.de/live)
Learn Real Analysis With This Excellent Book
Problems in Mathematics: Real Analysis-7
Real Analysis Exam 3 Review Problems and Solutions
Problems in Real Analysis | Ep. 8
Problems in Mathematics: Real Analysis-8
Комментарии
 1:05:33
1:05:33
 0:23:02
0:23:02
 0:26:14
0:26:14
 0:22:22
0:22:22
 0:09:12
0:09:12
 0:04:30
0:04:30
 0:23:53
0:23:53
 0:19:38
0:19:38
 0:11:23
0:11:23
 0:21:04
0:21:04
 0:19:18
0:19:18
 2:13:44
2:13:44
 0:00:53
0:00:53
 0:19:46
0:19:46
 0:02:27
0:02:27
 0:24:02
0:24:02
 1:19:35
1:19:35
 0:03:23
0:03:23
 2:13:31
2:13:31
 0:10:40
0:10:40
 0:06:11
0:06:11
 1:35:00
1:35:00
 0:18:17
0:18:17
 0:03:32
0:03:32