filmov
tv
Euler's log-trig integrals elegantly linked to a Berkeley Math Tournament integral
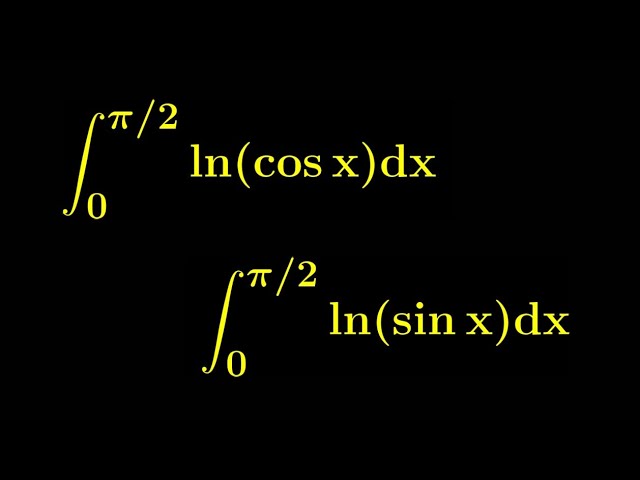
Показать описание
Exactly what the title says. You can solve these integrals using basic properties of the definite integral and high school integration techniques with some cool intuition.
Euler's log-trig integrals elegantly linked to a Berkeley Math Tournament integral
Euler's Integral
Every Student Should See This
MM02: Euler formula and integrals
The most beautiful equation in math, explained visually [Euler’s Formula]
Why greatest Mathematicians are not trying to prove Riemann Hypothesis? || #short #terencetao #maths
And You Thought Trigonometry Was Pointless…
Cosine and Sine Functions in terms of Complex Exponentials || Derivation from Euler's Formula
5 simple unsolvable equations
e^(iπ) in 3.14 minutes, using dynamics | DE5
The Beauty of Mathematics #inspiration #themanwhoknewinfinity
Albert Einstein doing physics | very rare video footage #shorts
Euler's Integrals l Beta Function l Gamma Function l First Eulerian Integral l Second Eulerian ...
Hyperbolic Functions - A Recreational Interlude: Euler’s Formula and the Unit Circle
ONE OF THE COOLEST INTEGRALS EVER!!! int ln(x)/(1+e^x) from 0 to infty
A ridiculously awesome integral with an epic result
A STUNNING integral from the JEE (main) exam
Another ridiculously awesome integral with a beautiful result
Feynman's Trick: MIT Integration Bee (23.5)
The Same Thing, but with Trig: MIT Integration Bee (21.5)
Is this hard integral from the 2020 Berkeley Math Tournament really that hard?
The essence of calculus
Physics Students Need to Know These 5 Methods for Differential Equations
Euler's Formula Beyond Complex Numbers
Комментарии
 0:13:14
0:13:14
 0:09:37
0:09:37
 0:00:58
0:00:58
 0:13:25
0:13:25
 0:26:57
0:26:57
 0:00:38
0:00:38
 0:01:00
0:01:00
 0:11:21
0:11:21
 0:00:50
0:00:50
 0:04:08
0:04:08
 0:00:59
0:00:59
 0:00:13
0:00:13
 0:08:29
0:08:29
 0:27:42
0:27:42
 0:19:24
0:19:24
 0:13:43
0:13:43
 0:09:47
0:09:47
 0:14:11
0:14:11
 0:15:33
0:15:33
 0:11:08
0:11:08
 0:11:42
0:11:42
 0:17:05
0:17:05
 0:30:36
0:30:36
 0:29:57
0:29:57