14 - What is Euler's Number 'e', Ln(x) - Natural Log & e^x Functions?

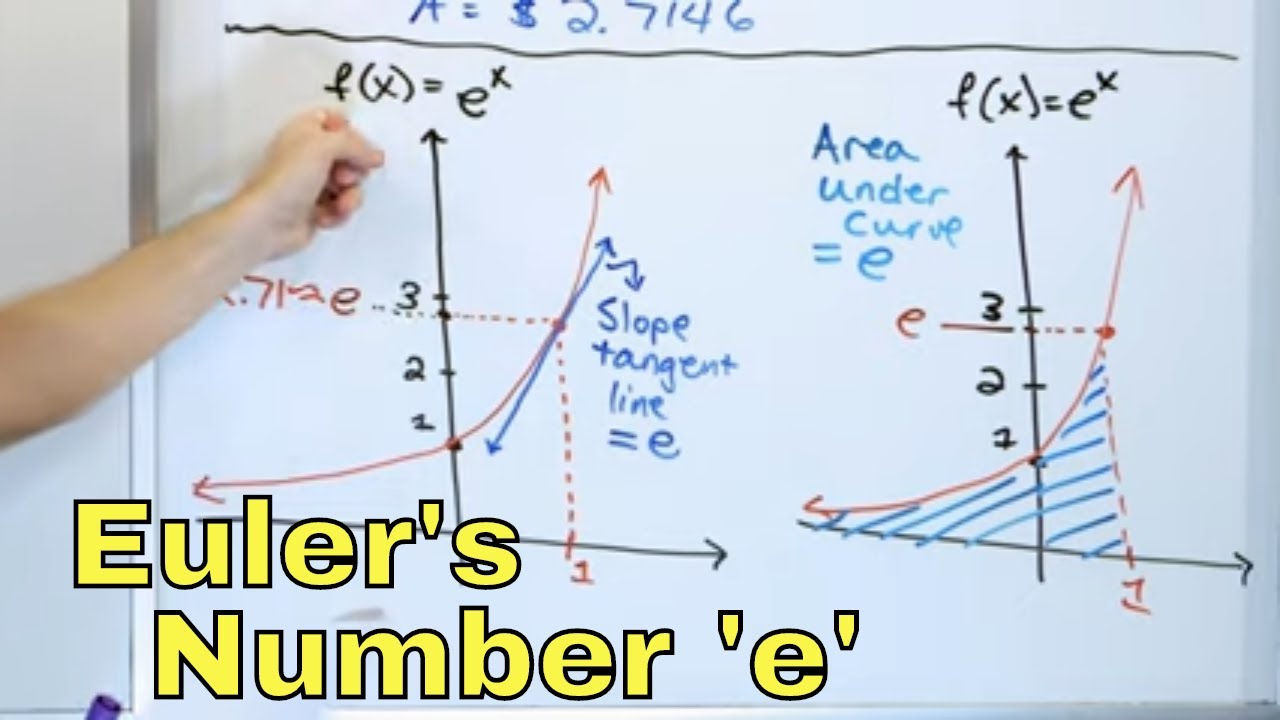
In this lesson, you will learn what Euler's number is and why it is important in math. We will learn that Euler's number comes from the exponential growth of money when the compounding period is allowed to get very, very small. Euler's number is then used to form the natural exponential function e^x and its inverse, the natural logarithm ln(x). The exponential function is special because it is the only function whose rate of change is equal to the value of the function itself and whose area under the curve is also equal to the value of the function itself.

[jimpringle]

I know how much harder is to teach using this method, and I really appreciate the effort you have put in these lessons. I am an engineer. I have used calculus in my career many times, but I must admit I never really understood it on a gut level. Unfortunately I never had an instructor who took his job as seriously as you. Thank you.

[onkeltschimi]

Love these lessons. Im an adult that never really did well in school. In todays world its really helping keep up at work by learning from youtube and for maths I highly recommend this guy!! Thanks for creating this content that is free for me👍👍 respect.

[dondonaldson]

I am an engineer and a keen lover of math. I was blessed to have a calculus teacher that explained this the same way that you did. I was also blessed to have a patient mother who drilled me in math so that I can do many complex calculations in my head by simplifying things back to first principles. My Dad was a mechanic who blessed me with the ingenuity to understand physics by asking questions that came from my curiosity. No wonder when I reached university the concepts of magnetism and electric fields helped me apply science and math to solve engineering problems. My favourite equation is the one you concluded with. e to the i*pi +1=0. It is so neat that the first derivative of the function (slope) is x and is the same as the integral (area under the curve) from - inf to x. Yes, Euler's number is indeed special.

[RonFitzgerald-wi]

This is the finest teaching of maths that I have ever come across. Absolutely compulsive. Dr Ron Fitzgerald

[defytyrantsofmississippi]

Jason your videos have really helped me understand more complex mathematics than I thought I could ever grasp. You truly are a master and 2nd to none at teaching complex mathematical concepts. Thank you so much for what you do.

[henrychen]

Please set this lesson as the standard class and textbook lesson to all the 1st year senior highs kids.
Now I have gotten it - why has the US been such a great nation? The instructor is the powerful answer!!!! Thank you so much

[TrinhNguyen-hcpz]

Nostalgia has brought a sixty something.here and it is quite amazing that something remaining in the dark for nearly half a century suddenly becomes clear, bright and simple. Thank you for your professional effort to simplify a complex subject.

[deirdreberger]

First off, your videos are excellent. Clear and to the point. Moving between the different whiteboards and using the different colored markers is brilliant. With schools closed, my daughter is watching your videos to finish off her senior year in high school. She will continue with her transition to college. Hopefully schools are open by then.

[nicknike]

I've been looking for this method of teaching math for like 3 years. I literally appreciate the effort.

[thescouselander]

One of the best things I've seen on YouTube. I've been using this stuff for years but nobody ever explained how it was derived or the meaning of the number. If only my maths lecturers were this good.

[mikemullenix]

This is one of the best examples and explanation of e and compound interest I've seen. So important for understanding the magic of compound interest.

[mohammedhafiz]

Watched this explanation of "e" number first time, Understood it first time.
Sir you are the Master we should have had in my school days, life would have been quite different.
Thank you so much. It makes it lot easier for our children to enjoy Maths. Thanks

[edvargas]

Brilliant explanation with simple language. I took math courses up to Differential equations and 'e' popped up everywhere, but I did not know why 'e' was so fundamental until now. Thanks a million!

[sebastiansalazar]

I received math lessons in my High school Education and in College. I am happy to tell you that your explanation of the Euler's number is the best and simple one I’ve seen. Many thanks for your time.

[felixyongco]

Thanks a lot and I am reminded of my days 58 YEARS ago.

You are such a very, very good teacher.

[cluelessinky]

Wish I had this instructor 60 years ago when I was studying calculus. It took me forever to understand the concept and he made it clear in ten minutes Well done and thank you

[bandreon]

What a stunning lesson, brilliant in its simplicity!

[DonaldWhiteIII]

What a great lesson. Some of the best teaching I have ever seen. The visualization and verbalization was incredible

[kamalhameedbhatti]

Didn't even noticed that 43 minutes of my life just passed by watching a lesson that had nothing to do with my life *BUT* it was the most interesting thing that I learnt today just for the sake of general you son.

[vythinathanduraiswamy]

The origin and importance of 'e' so well explained. You are an incredibly good and passionate teacher! Thank you very much.