filmov
tv
OMG, It Works! Solving a Mystery with Euler's Identity (MindSphere shorts)#maths
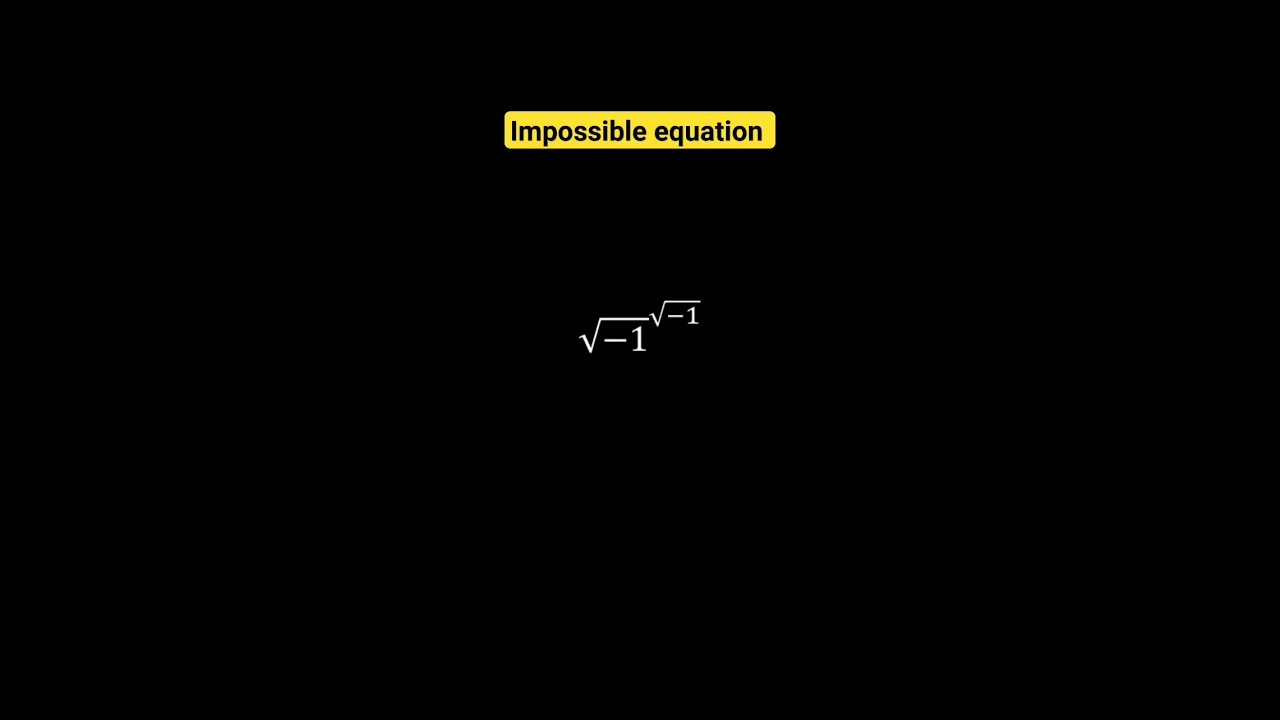
Показать описание
Explore the vast realm of mathematics with this extensive list of keywords, spanning topics such as addition, subtraction, multiplication, division, algebra, geometry, calculus, trigonometry, functions, equations, graphs, probability, statistics, prime numbers, fractions, decimals, percentages, exponents, logarithms, matrices, vectors, complex numbers, sequences, series, limits, derivatives, integrals, differential equations, linear algebra, quadratic equations, arithmetic, geometric shapes, circle, triangle, square, rectangle, parabola, hyperbola, ellipse, congruence, similarity, Pythagorean theorem, area, volume, perimeter, surface area, mean, median, mode, range, standard deviation, variance, probability distribution, normal distribution, binomial distribution, Poisson distribution, exponential distribution, Chi-square distribution, hypothesis testing, confidence intervals, regression analysis, correlation, interpolation, extrapolation, sets, union, intersection, complement, disjoint sets, universal set, subset, Venn diagrams, Cartesian coordinates, polar coordinates, parametric equations, complex plane, eigenvalues, eigenvectors, arithmetic progression, geometric progression, Fibonacci sequence, Pascal's triangle, Newton's method, Riemann sum, Fourier series, Taylor series, vector calculus, partial derivatives, gradient, divergence, curl, Laplace transform, Fourier transform, Z-transform, convolution, matrix multiplication, determinant, inverse matrix, linear regression, nonlinear regression, optimization, critical points, inflection points, sine function, cosine function, tangent function, cosecant function, secant function, cotangent function, arcsin function, arccos function, arctan function, hyperbolic functions, limits at infinity, indefinite integrals, definite integrals, fundamental theorem of calculus, chain rule, product rule, quotient rule, mean value theorem, integration by parts, integration by substitution, polar integration, surface integrals, line integrals, Green's theorem, divergence theorem, Stokes' theorem, Laplace's equation, boundary value ion, Fibonacci heap, golden ratio, Gaussian elimination, LU decomposition, Cholesky decomposition, QR decomposition, singular value decomposition, Gram-Schmidt process, least squares method, matrix factorization, eigen decomposition, power iteration, Jacobi method, Gauss-Seidel method, Newton-Raphson method, bisection method, secant method, regula falsi method, Brent's method, interpolation methods, Lagrange interpolation, Newton interpolation, Hermite interpolation, cubic spline interpolation, Chebyshev interpolation, Neville's algorithm, Neville's theta algorithm, Runge-Kutta methods, Euler method, Heun's method, midpoint method, fourth-order Runge-Kutta method, Adams-Bashforth method, Adams-Moulton method, multistep methods, boundary value methods.
#AlgebraIsAwesome #CalculusCrunch #GeometryGuru #ShapesOfMath #StatsSaturday #ProbabilityPuzzle #PrimePower #NumberNerd #ArithmeticAdventures #TrigTuesdays #MathMagic #MathematicsMatters #GeometricGenius #CalculusConcepts #AlgebraicExpressions #GeometryGoals #StatisticalInsights #ProbabilityPlays #PrimeNumbersOnly #NumberTheory #ArithmeticArt #TrigonometryTricks #MathematicalMind #MathMania #AlgebraAllDay #CalculusCraze #GeometryGenius #StatisticalStudies #ProbabilityPatterns #PrimeNumberPuzzles #NumberNinja #ArithmeticAddict #TrigonometryTales #MathematicalMaster #AlgebraicAdventures #CalculusChallenges #GeometryGreatness #StatisticalSolutions #ProbabilityProblems #PrimePowerhouse #NumberNerdiness #ArithmeticAficionado #TrigonometryTips #MathematicalMusings #AlgebraAcademy #CalculusConundrums #GeometryGalore #StatisticalAnalysis #ProbabilityPonderings #PrimeNumberPassion #NumberNovice #ArithmeticAmazement #TrigonometryTalk #MathematicalMarvels #AlgebraArtistry #CalculusCapers #GeometryGems #StatisticalStrategies #ProbabilityPuzzles # #MathematicalMysteries #AlgebraicAwesomeness #CalculusChampions #GeometryGoodness #StatisticalStudiousness #ProbabilityPuzzlers #PrimeNumberParadise #NumberNiche #ArithmeticAppreciation #TrigonometryTales #MathematicalMarvels
Keywords:
1. Funny
2. Comedy
3. Hilarious
4. Entertaining
5. Lighthearted
6. Amusing use
7. Quirky
8. Witty
9. Clever
10. Creative
11. Memorable
12. Unexpected
13. Surprising
14. Ingenious
15. Offbeat
16. Humorous
17. Quick
18. Snappy
19. Catchy
20. Trendy How to solve equations with Euler's identity
Euler's identity explained simply
Beginner's guide to Euler's formula
The magic of Euler's identity (if your video takes a more awe-inspiring approach)
Euler's identity MindSphere Shorts (combination of main and channel keyword)
#euleridentity
#eulersformula
#exponentialfunction
#trigonometry
#imaginaryunit
#mindsphere
#mindsphereshorts
#learnmath
Hashtags:
1. #FunnyShorts
2. #ComedyClips
3. #HilariousMoments
4. #EntertainmentShorts
5. #QuirkyVibes
6. #WittyHumor
7. #
13. #QuickLaughs
17. #s
20. #ViralVibes
#AlgebraIsAwesome #CalculusCrunch #GeometryGuru #ShapesOfMath #StatsSaturday #ProbabilityPuzzle #PrimePower #NumberNerd #ArithmeticAdventures #TrigTuesdays #MathMagic #MathematicsMatters #GeometricGenius #CalculusConcepts #AlgebraicExpressions #GeometryGoals #StatisticalInsights #ProbabilityPlays #PrimeNumbersOnly #NumberTheory #ArithmeticArt #TrigonometryTricks #MathematicalMind #MathMania #AlgebraAllDay #CalculusCraze #GeometryGenius #StatisticalStudies #ProbabilityPatterns #PrimeNumberPuzzles #NumberNinja #ArithmeticAddict #TrigonometryTales #MathematicalMaster #AlgebraicAdventures #CalculusChallenges #GeometryGreatness #StatisticalSolutions #ProbabilityProblems #PrimePowerhouse #NumberNerdiness #ArithmeticAficionado #TrigonometryTips #MathematicalMusings #AlgebraAcademy #CalculusConundrums #GeometryGalore #StatisticalAnalysis #ProbabilityPonderings #PrimeNumberPassion #NumberNovice #ArithmeticAmazement #TrigonometryTalk #MathematicalMarvels #AlgebraArtistry #CalculusCapers #GeometryGems #StatisticalStrategies #ProbabilityPuzzles # #MathematicalMysteries #AlgebraicAwesomeness #CalculusChampions #GeometryGoodness #StatisticalStudiousness #ProbabilityPuzzlers #PrimeNumberParadise #NumberNiche #ArithmeticAppreciation #TrigonometryTales #MathematicalMarvels
Keywords:
1. Funny
2. Comedy
3. Hilarious
4. Entertaining
5. Lighthearted
6. Amusing use
7. Quirky
8. Witty
9. Clever
10. Creative
11. Memorable
12. Unexpected
13. Surprising
14. Ingenious
15. Offbeat
16. Humorous
17. Quick
18. Snappy
19. Catchy
20. Trendy How to solve equations with Euler's identity
Euler's identity explained simply
Beginner's guide to Euler's formula
The magic of Euler's identity (if your video takes a more awe-inspiring approach)
Euler's identity MindSphere Shorts (combination of main and channel keyword)
#euleridentity
#eulersformula
#exponentialfunction
#trigonometry
#imaginaryunit
#mindsphere
#mindsphereshorts
#learnmath
Hashtags:
1. #FunnyShorts
2. #ComedyClips
3. #HilariousMoments
4. #EntertainmentShorts
5. #QuirkyVibes
6. #WittyHumor
7. #
13. #QuickLaughs
17. #s
20. #ViralVibes
Комментарии
 0:00:22
0:00:22
 0:00:18
0:00:18
 0:00:37
0:00:37
 0:00:25
0:00:25
 0:21:12
0:21:12
 0:00:16
0:00:16
 0:24:08
0:24:08
 0:00:56
0:00:56
 0:03:27
0:03:27
 0:00:34
0:00:34
 0:11:26
0:11:26
 0:00:23
0:00:23
 0:00:38
0:00:38
 0:00:41
0:00:41
 0:01:52
0:01:52
 0:00:57
0:00:57
 0:00:30
0:00:30
 0:35:14
0:35:14
 0:00:35
0:00:35
 0:00:36
0:00:36
 0:00:46
0:00:46
 0:00:15
0:00:15
 0:00:05
0:00:05
 0:02:03
0:02:03