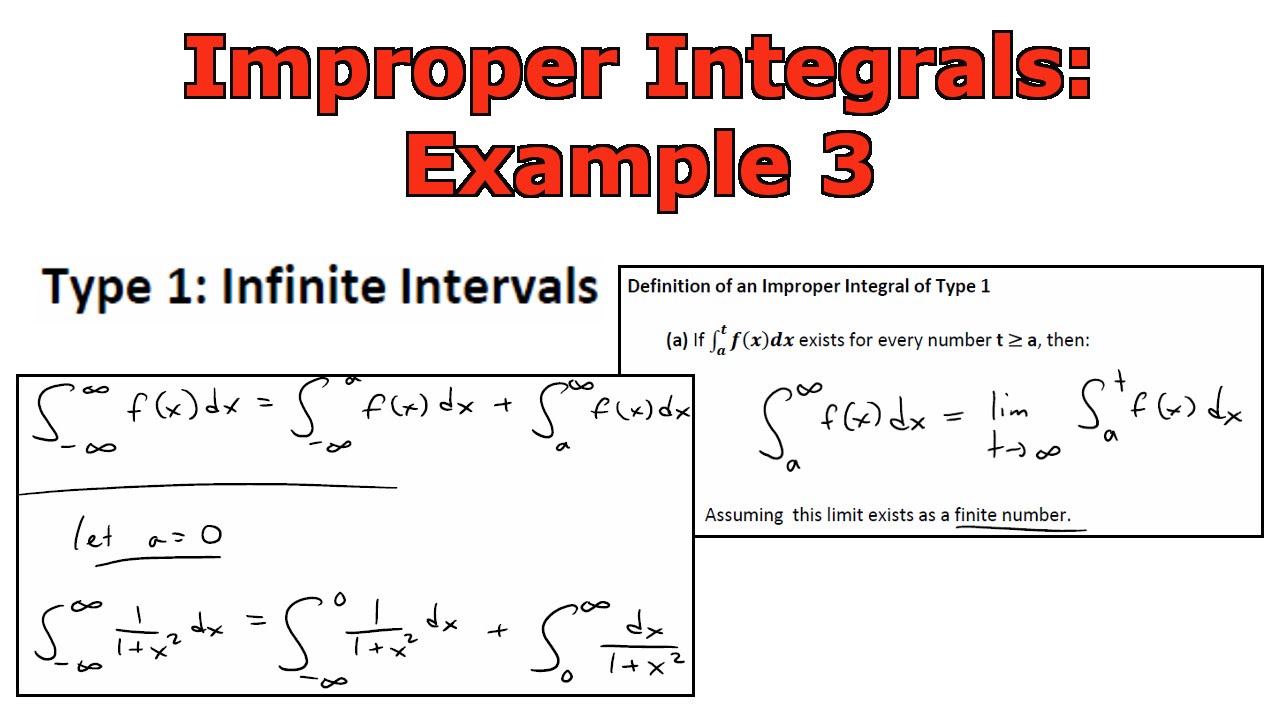
Improper Integrals: Example 3: 1/(1+x^2)

In this video I go over another example on improper integrals and this time solve the integral of the function 1/(1+x^2) from x approaches negative infinity to x approaches positive infinity. This integral can be simplified into two parts by integrating it from negative infinity until a number, chosen as zero in this example for convenience, and then from that number to positive infinity. This integral is very interesting as it converges at both positive and negative infinity resulting in the integral, and thus the area under the curve, to be equal to the number π.

Related Videos:

------------------------------------------------------

[mes]

I don't always integrate improper integrals from negative infinity to positive infinity but when I do I usually break the integral into 2 integrals and sum them up ;)