filmov
tv
Exponential Equation| Unique Exponential | Absolute Value | Solve x=–x^(x^2–3x+4/2x) for x
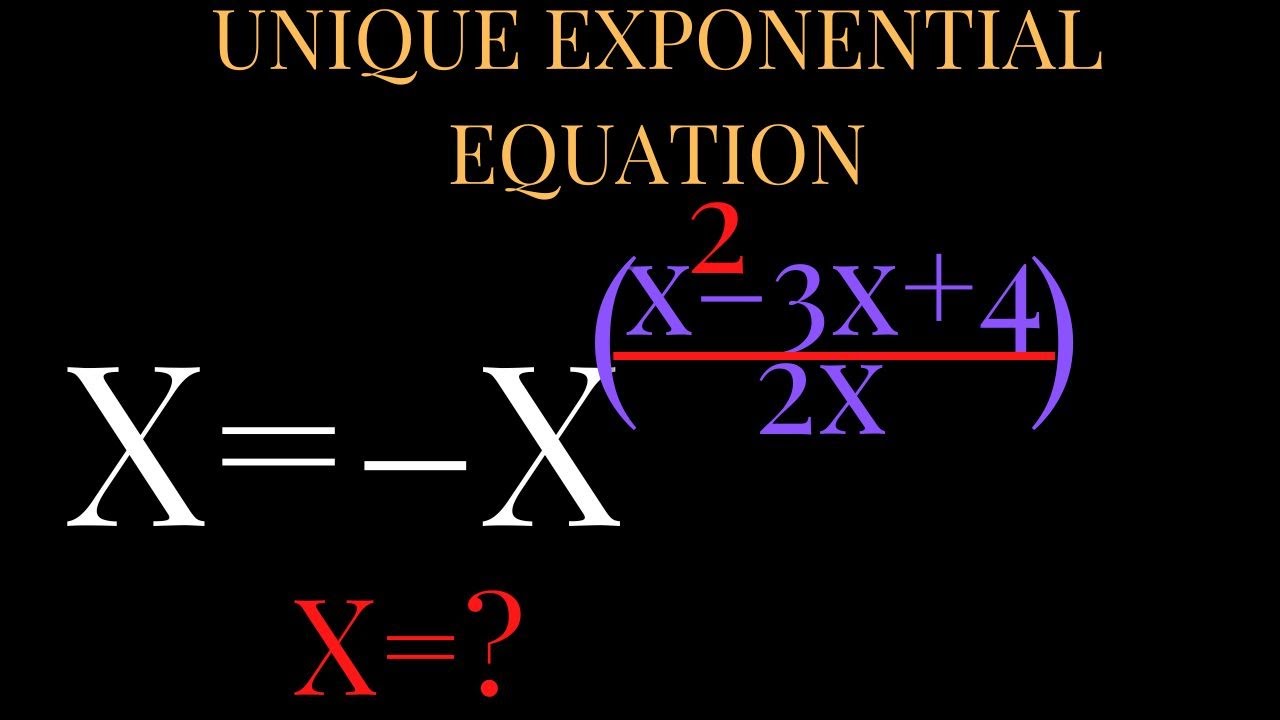
Показать описание
Exponential Equations occur in various formant.
some may appear complex why others may appear simple.
The tricks to solving exponential equations lies in the knowing of the major laws of indices and logarithms alongside their applications.
In this video you will learn how to solve exponential equations with entangled or quadratic powers.
One of the most important things you will learn from this video is how to use the absolute value to eliminate the negative or minus sign attached to the variable to enable the solving of the equation.
I will show you how to apply the power law of logarithms to resolve some steps.
Also in this vide, you will be exposed to the use or application of the division law of indices.
You will learn how to make use of the natural logarithm in order to solve for the variable x.
To get all the listed features in this video, watch from the beginning to the end of it without skipping any parts.
Besides, if you are new to this channel, kindly subscribe to get all our new videos.
Thanks.
some may appear complex why others may appear simple.
The tricks to solving exponential equations lies in the knowing of the major laws of indices and logarithms alongside their applications.
In this video you will learn how to solve exponential equations with entangled or quadratic powers.
One of the most important things you will learn from this video is how to use the absolute value to eliminate the negative or minus sign attached to the variable to enable the solving of the equation.
I will show you how to apply the power law of logarithms to resolve some steps.
Also in this vide, you will be exposed to the use or application of the division law of indices.
You will learn how to make use of the natural logarithm in order to solve for the variable x.
To get all the listed features in this video, watch from the beginning to the end of it without skipping any parts.
Besides, if you are new to this channel, kindly subscribe to get all our new videos.
Thanks.
A Cool Exponential Equation | Math Olympiads
A Cool Exponential Equation | Math Olympiads
A Cool Exponential Equation | Algebra
Solving Exponential Equations
A Cool Exponential Equation
How to Graph Exponential Functions
Solving Exponential Equation
Solving a Beautiful Exponential Equation!
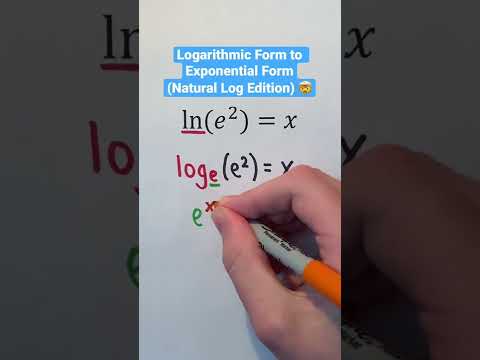
Logarithmic Form to Exponential Form (Natural Log Edition) 🤯 #Shorts #algebra #math #education
exponential function | infinite series #calculus #math #animation #learn #study
Solving A Quick and Easy Exponential Equation
Solving Exponential Equation
A Cool Exponential Equation | Algebra
A Cool Exponential Equation | Math Olympiads
Exponential Form to Logarithmic Form #Shorts #algebra #math #maths #mathematics #lesson #howto
Exponential Equation Grade 10
Solving A Cool Exponential Equation
A Cool Exponential Equation | i^x=e
A Nice Exponential Equation | A Special Function
Exponential Equations #maths #mathematics #shorts
Exponential Equations - Algebra and Precalculus
Solving Exponential Equations with Common Bases | Algebra
Can you solve this? | Exponential Equation | Algebra Problem.
Exponential derivative visual
Комментарии
 0:08:18
0:08:18
 0:10:06
0:10:06
 0:00:25
0:00:25
 0:16:36
0:16:36
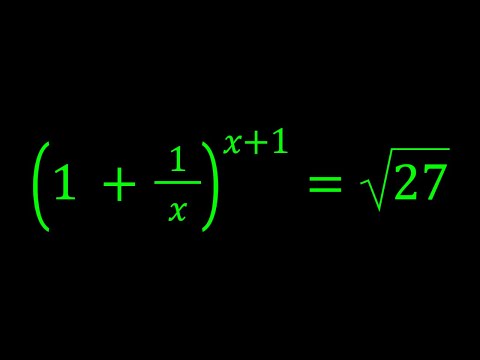 0:08:37
0:08:37
 0:00:40
0:00:40
 0:00:56
0:00:56
 0:00:31
0:00:31
 0:00:28
0:00:28
 0:00:12
0:00:12
 0:00:28
0:00:28
 0:00:54
0:00:54
 0:09:39
0:09:39
 0:09:36
0:09:36
 0:00:22
0:00:22
 0:01:39
0:01:39
 0:09:13
0:09:13
 0:09:21
0:09:21
 0:08:34
0:08:34
 0:00:35
0:00:35
 0:05:58
0:05:58
 0:00:55
0:00:55
 0:03:33
0:03:33
 0:00:57
0:00:57