filmov
tv
Complex Analysis 8 | Wirtinger Derivatives
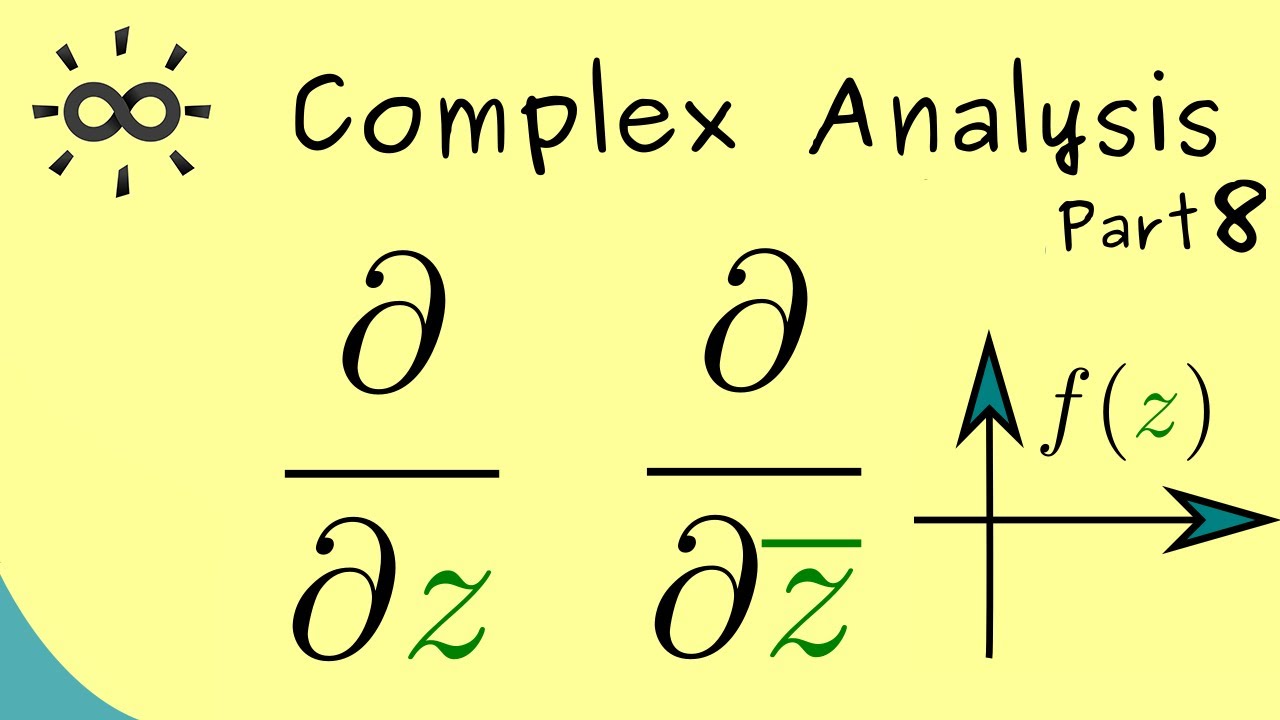
Показать описание
Please consider to support me if this video was helpful such that I can continue to produce them :)
🙏 Thanks to all supporters! They are mentioned in the credits of the video :)
Thanks to all supporters who made this video possible! They are mentioned in the credits of the video :)
This is my video series about Complex Analysis. I hope that it will help everyone who wants to learn about complex derivatives, curve integrals, and the residue theorem. Complex Analysis has a lof applications in other parts of mathematics and in physics.
x
00:00 Intro
00:19 Wirtinger derivatives — basic definition
01:35 Complex derivative through Cauchy-Riemann equations
05:12 Wirtinger derivatives — detailed definition
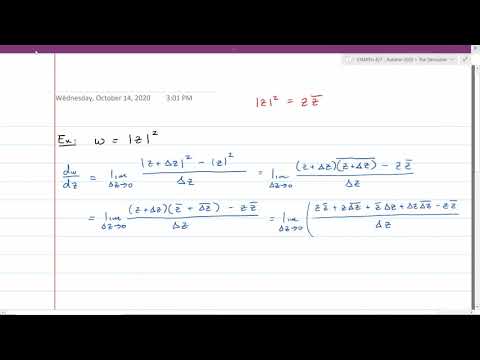
06:17 Example for z²
08:39 Summary: a criteria for holomorphic functions
#ComplexAnalysis
#Analysis
#Calculus
#Mathematics
#curveintegral
#integration
(This explanation fits to lectures for students in their first or second year of study: Mathematics, Mathematics for physicists, Mathematics for the natural science, Mathematics for engineers and so on)
Комментарии
 0:09:47
0:09:47
 0:12:28
0:12:28
 0:13:25
0:13:25
 0:51:20
0:51:20
 0:20:41
0:20:41
 0:59:51
0:59:51
 0:12:40
0:12:40
 0:40:37
0:40:37
 0:17:04
0:17:04
 0:15:03
0:15:03
 0:59:51
0:59:51
 0:22:21
0:22:21
 0:49:25
0:49:25
 0:10:45
0:10:45
 0:11:02
0:11:02
 0:13:18
0:13:18
 0:18:55
0:18:55
 0:29:24
0:29:24
 0:01:01
0:01:01
 0:14:04
0:14:04
 0:26:23
0:26:23
 0:08:54
0:08:54
 0:58:18
0:58:18
 0:07:11
0:07:11