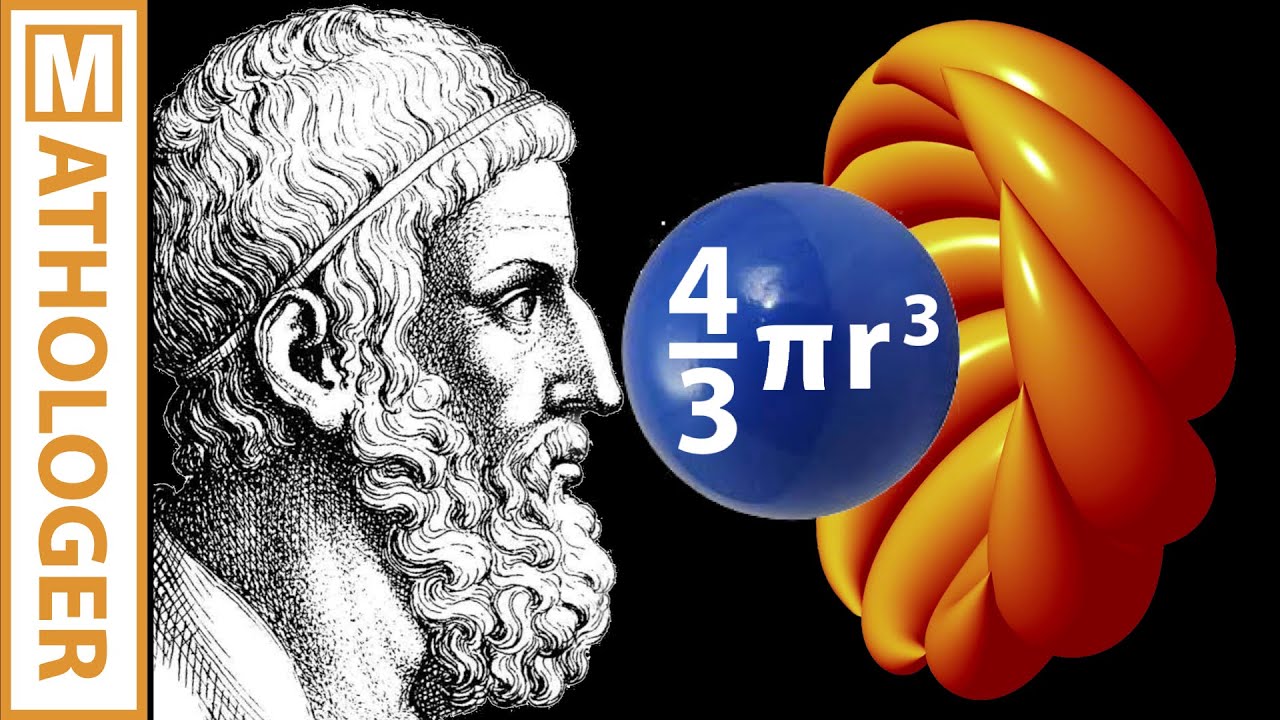
Why are the formulas for the sphere so weird? (major upgrade of Archimedes' greatest discoveries)

In today’s video we’ll make a little bit of mathematical history. I'll tell you about a major upgrade of one of Archimedes' greatest discoveries about the good old sphere that so far only a handful of mathematicians know about.

00:00 Intro to the baggage carousel
01:04 Archimedes baggage carousel
04:26 Inside-out animations
04:59 Inside-out discussion
10:38 Inside-out paraboloid
12:43 Ratio 3:2
13:28 Volume to area
18:40 Archimedes' claw
20:55 Unfolding the Earth
29:43 Lotus animation
30:38 Thanks!

Great wiki page on Archimedes

In "On the sphere and the cylinder"

Why is the formula for the surface area the derivative of the volume formula? Easy:
V'(r) = dV/dr = A(r) dr / dr = A(r).

Wiki page on Cavalieri's principle
Includes both hemisphere = cylinder - cone and paraboloid = cylinder - paraboloid
Video on the volume of the paraboloid using Cavalieri by Mathemaniac

Andrew's playlist of spectacular video clips complementing this Mathologer video:
All of Andrew's animations featured in this video plus a few more (actual footage of a fancy baggage carousel in action, alternative proof that we are really dealing with a cylinder minus a cone, paraboloid inside-out action, inside-out circle to prove the relationship between the area and circumference of the circle, etc.)

Funniest comment: Historians attempting to reconstruct the Claw of Archimedes have long debated how the weapon actually worked. The sources seem to have trouble describing exactly what it did, and now we know why. Turns out it was a giant disc that slid beneath the waters of a Roman ship, then raised countless eldritch crescents which inexplicably twisted into a sphere, entrapping the vessel before dragging it under the waves, all while NEVER LEAVING ANY GAPS in the entire process. No escape, no survivors, fucking terrifying. No wonder that Roman soldier killed Archimedes in the end, against the Consul's orders. Gods know what other WMDs this man would unleash on the battlefield if he were allowed to draw even one more circle in the sand. The Roman marines probably had enough PTSD from circles.

T-shirt: One of my own ones from a couple of years ago.
Music: Taiyo (Sun) by Ian Post

Enjoy!

Burkard

P.S.: Thanks you Sharyn, Cam, Tilly, and Tom for your last minute field-testing.

[theo]

Archimides was doing calculus without algebra. No wonder he's your favourite. That's pretty much the spirit of this channel if you think about it.

[NoNameAtAll]

1:36 "circle gets turned inside out"

**"smiles and frowns" flashbacks intensify**

[andrewkepert]

Thanks Burkard for putting together such a nice presentation and filling in so many connections. It has been a load of fun working with you and Henry on this.

[not_sure-no]

I remember when I first noticed the derivative relationship between volume, surface area, and circumference of the sphere. It’s been over a decade since my first calculus course but it’s still so satisfying. When you consider that the derivative is a rate of change, the relationship begins to make perfect sense. I think what really made it click was when we started doing integrals. If you integrate the circumference from r=0 to r=R, you wind up with a sweep of concentric rings that cover the whole area, meaning that the rate of change of the area is given as the circumference as r is varied. Similarly, as you integrate the surface shells across the range of r- values, you wind up with the whole sphere! It’s brilliant once it all clicks and you can see those animations in the mind 😊

[aafeer]

If you make a PDF I will buy it, and probably I will not be the only one. This really associates the formal math and the intuition in a striking way. Well designed, brilliant accomplishment.

[Wecoc]

Congrats on your 100th video! Very special number, since it's the fourth octadecagonal number, amongst other things 😆

[alokaggarwal]

Congratulations on 100 videos! Your channel is awesome ❤

[gonshi]

Awesome video (im a 33m into the past time traveler)

[caspermadlener]

Hey, I won gold because of this channel (long story lol), and have a suggestion for a small addition to the part two.
The correspondence between the map of Lambert and Kepert is done by taking circle segments between two points, and varying the "angle" of the circle segment. 0° is a line segment, and 90° is a halfcircle, like used in the video.

One of my own proofs, of the cylic quadrilateral angle theorem (that has undoubtedly been found by someone else as well) is that given a quadrilateral, you can look at the line segments like they are circle segments. The "circle segment quadrilaterals" have invariant α–β+γ–δ. Since you can merge two pairs of circle segments, you basically directly get the theorem.
This even works for hyperbolic geometry!

It is a nice proof, using unorthodox techniques, so it probably has to show up on this channel eventually, although it likely won't fit.

It also has a dual theorem, where the opposing sides of a quadrilateral sum to the same length if and only if the quadrilateral has an inscribed circle.

[skyscraperfan]

It makes sense that the area is the derivative of the volume, if you think that the volume is created by adding all the surfaces of the spheres with smaller radiuses. Basically like blowing up a perfectly spherical balloon. That trick should work for any shape, not only spheres. For example a cube defined by the 8 points (+/-r, +/-r, +/-r) has a volume of 8r^3. If you drive that by r, you get 24r^2 and that is exactly the surface of such a cube with side length 2r.

[vinzdini]

I remember learning that the volume of a sphere equals the volume of the respective cylinder minus that of the cone back in school, but it completely blew my mind to learn the equivalent is also true for a parabola. Also, it's amazing how the derivative of the volume of a shpere is its surface area. Made me realize how I've been taking things for granted withhout actually analyzing them. Thank you for providing me with all this insight! Keep making great videos like this!!

[ianfowler]

The real key here is that we now have a transformation in which all the CURVED longitudes in 3-space map to a single flat surface with the same length - no distortion. The curved longitude really is a flat 2-d curve if you just look at it from the right perspective but now ALL of them map to the same plane! Then the area stuff follows by INTEGRATING over closer and closer longitudes. For me, this elusive mapping is a real game changer. I mean it really is. Curved arcs on the sphere are now lying on the same flat plane with no distortion from which it follows (by integration) that curved area of sphere = flat area of circle (like adding up the arc lengths of an infinite number of undistorted semi circular longitudes to get the area). With radius of 2R to boot! No stretching or squeezing. Well done and thank you ALL so, so much!

This has resolved a long time major conflict in my own mind trying to understand what curved area really means and how we can transform a curved area into an equal flat area with no distortion - which what area really is defined as all along - flat.

[briancooke]

I now understand why the edges of 3d shapes of constant width look just like the animation at 27 minutes! It's a sphere being mapped between the vertices, so elated! Thank you, Mathologer, for another wonder full lesson!

[FloydMaxwell]

Congratulations on 100 videos. Your videos are impressive, to say the least.

[nanamacapagal]

Congrats on 100 videos, mate. You really made it as a maths educator and content creator on YouTube, and I'm looking forward to seeing you do even better in the future. Hope you blow our minds again with each video you make

That said...

CHALLENGES!

11:18
I have no army of middle school minions but I am still ready to attack
Same reasoning as before. This time we start with the second paraboloid, the one carved from the cylinder. Makes the maths a little easier.
The paraboloid has radius R and height H. Cutting it at height h will leave a ring with outer radius R and inner radius r.
The paraboloid is modeled after a parabola y=ax^2, and so we should have H = aR^2 and h = ar^2. So it's possible to solve for r and get r = Rsqrt(h/H).
The ring thus has area pi * R^2 - pi * R^2 * h/H, or piR^2(1-h/H).
The first paraboloid should also have that area. Thus its radius should be Rsqrt(1-h/H).
Now the inverted paraboloid can be modeled by another quadratic, but the important takeaways are H = bR^2 and H - h = br^2. Solving for r this time gives Rsqrt(1-h/H), exactly the same as what was predicted by the circles area argument.


Or you can use integrals. Whatever floats your boat

17:42
The layers of the onion look almost like surface areas stuck together. That can be written as: V(R) = the integral from 0 to R of SA(r)dr
By FTC1, we can also write this as V'(R) = SA(R)
And so the derivative of the volume is the surface area. Even in 420 dimensions.

18:04
The base of the cylinder has area piR^2. The height is 2R, and the circumference is 2piR. In total, the surface area of the cylinder is 6piR.
With the surface area of the sphere being 4piR, the ratio of the surface areas of the two shapes really is 3:2.

[tractorboy]

My favourite Mathologer video thus far. Props to Archimedes et al.

[obscurity]

Congratulations on hitting 100 videos! That’s quite a milestone! Love your content, Mathologer!

[jacobbaer]

8:00
Just filling in the details of this proof because i havent seen any other commenter do so yet: (unless i missed them, idk)

Set R = outside radius of sphere= outside radius of cylinder.
h = height of our cut plane
r = radius of the circle x-section
And a = inside radius of ring.

Area of the circle cross section: A1 = pi*r^2 = pi(R^2 - h^2) via the Pythagoran theorem.
Area of the ring: A2 = pi (R^2 - a^2)

A1 will equal A2 if we set a = h, so then the subtracted cone will have straight sides with rise equal to run, and therefore the cone is actually a cone.

[bfbyzqy]

Gratulation zum 100. Video!
Alles ist wirklich ein Hochgenuss, einfach perfekt, vielen Dank!
👍

[uuxnvsy]

Congratulations Mr. Polster (and Marty) for your 100th video! The more I watch, the more I ❤ it.