filmov
tv
Solving x^{ln(x)}=ex

Показать описание
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #ExponentialEquations
via @YouTube @Apple @Desmos @NotabilityApp
@googledocs @canva
PLAYLISTS 🎵 :
#ChallengingMathProblems #ExponentialEquations
via @YouTube @Apple @Desmos @NotabilityApp
@googledocs @canva
PLAYLISTS 🎵 :
Solving x^{ln(x)}=ex
Is e^x=ln(x) solvable?
The Exponential Function e and The Natural Log ln
e^x=ln(x)
Solving an natural logarithmic equation using properties of logs
Solving x^{ln(x)}=xln(x) | A Log Equation
Solving e^x=ln(x)/log(x)
Solving the Logarithmic Equation 5*ln(x) = 10
Week 4| NPTEL Quiz-3 solution| Practice Problems on SVM
Natural Logarithms
OCR MEI Core 3 1.14 Solve ln(x + 1) + ln(x) = ln(6)
Solve for x ln(ln x) = 1, e^(e^x) = 10. Natural exponential functions
Simplify each natural exponential expression. Ln(ln e^(e^10), e^(x + ln x), e^(-2 ln 5)
NATURAL LOGARITHM: SOLVING FOR X
Solve the Exponential Equation e^(x + ln(3)) = 4e^x
AQA Core 3 1.03 Solving equations with e^x and ln(x)
Solve for x ln x less than 0 and e^x is greater than 5. Natural exponential inequalities
Solve for x, e^(3x +1) = k, and ln x + ln(x-1) = 1. Natural exponential equations
What is e and ln(x)? (Euler's Number and The Natural Logarithm)
Why does e^(ln x) = x
How do you solve an exponential equation with e as the base
Logarithms... How? (NancyPi)
How to differentiate e^x * ln(x) using the product rule
Solve for x e^(2x) - 3e^x + = 0, and ln(x^2 -1) = 3. Natural exponential functions
Комментарии
 0:05:37
0:05:37
 0:06:32
0:06:32
 0:10:16
0:10:16
 0:04:55
0:04:55
 0:01:15
0:01:15
 0:09:33
0:09:33
 0:06:25
0:06:25
 0:00:36
0:00:36
 2:49:04
2:49:04
 0:02:49
0:02:49
 0:01:35
0:01:35
 0:03:44
0:03:44
 0:04:41
0:04:41
 0:04:41
0:04:41
 0:02:18
0:02:18
 0:10:30
0:10:30
 0:02:10
0:02:10
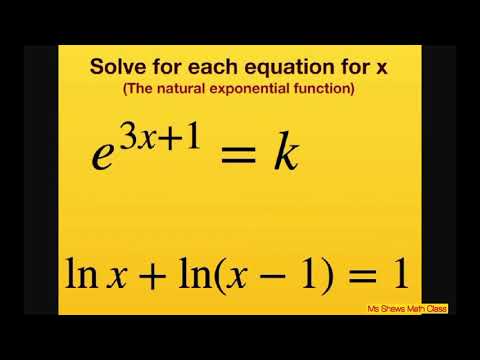 0:03:25
0:03:25
 0:12:02
0:12:02
 0:02:05
0:02:05
 0:02:47
0:02:47
 0:19:15
0:19:15
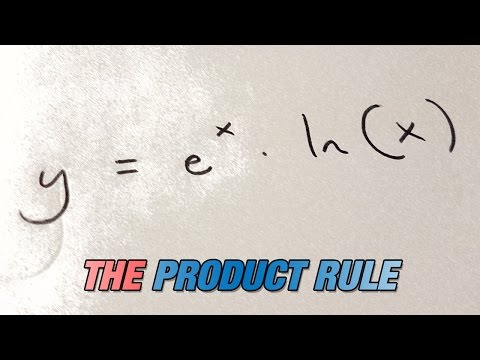 0:01:52
0:01:52
 0:03:40
0:03:40