Complex analysis: Summing series

preview_player
Показать описание
This lecture is part of an online undergraduate course on complex analysis.
This is a replacement for a previous video, correcting some minor typos.

We show how to use the residue calculus to sum series, such as Euler's series 1/1^2 + 1/2^2+ ...

Solution to exercise in rot 13: cv phorq bire guvegl gjb

  1. Richard E Borcherds
  2. Introduction
  3. Complex analysis
Рекомендации по теме
Complex analysis: Summing 0:16:43

Complex analysis: Summing series

Laurent Series Explained 0:13:56

Laurent Series Explained | How to Determine Laurent Series | Complex Analysis #9

[Complex Analysis] How 0:01:07

[Complex Analysis] How to find the infinite sum of (i/3)^n

Complex Analysis: Lecture 0:44:33

Complex Analysis: Lecture 35: series calculation via residues

Complex Analysis: One 0:15:37

Complex Analysis: One EXOTIC Infinite Series

Convergence and Divergence 0:16:18

Convergence and Divergence - Introduction to Series

Complex Analysis: Tricky 0:11:07

Complex Analysis: Tricky Sum Problem

But what is 0:22:11

But what is the Riemann zeta function? Visualizing analytic continuation

Solving AC Circuit 0:17:01

Solving AC Circuit Problems Impedance, Filters & Frequency Response

Every Student Should 0:00:58

Every Student Should See This

Taylor Series for 0:23:35

Taylor Series for Complex Valued Functions -- Complex Analysis 17

Laurent Series Harder 0:05:50

Laurent Series Harder Example

Complex Analysis: Basel 0:19:08

Complex Analysis: Basel Problem Variation

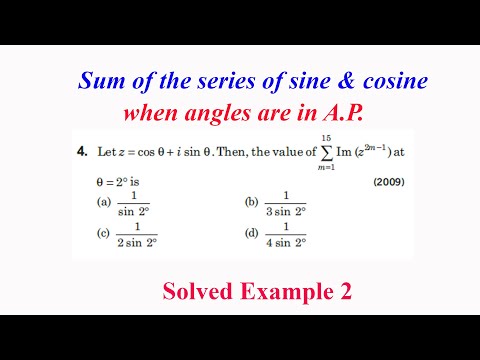
Solved example 2| 0:02:59

Solved example 2| Complex numbers | Sum of the series of sine & cosine when angles are in A.P.

A-Level Further Maths 0:14:45

A-Level Further Maths B9-05 Complex Numbers: Summing Series Example

Series | Lecture 0:04:31

Series | Lecture 15 | Finding the Sum of Series using Sequence of Partial Sum

Olympiad level counting 0:34:36

Olympiad level counting (Generating functions)

SUM of N 0:05:48

SUM of N COMPLEX exponentials

Example of Laurent's 0:14:04

Example of Laurent's Series | L16 | TYBSc Maths | Complex Power Series @ranjankhatu

Complex Analysis: Lecture 0:48:29

Complex Analysis: Lecture 25: sequences, convergence

Complex Integrals | 0:24:26

Complex Integrals | Chapter 3 Introduction to Complex Analysis

Complex Analysis | 0:02:15

Complex Analysis | Unit 1 | Lecture 24 | Power Series

But what is 0:24:47

But what is a Fourier series? From heat flow to drawing with circles | DE4

Complex Analysis | 0:09:05

Complex Analysis | Unit 2 | Lecture 13 | Example of Cauchy's Integral Formula

Комментарии
Автор

That was amazing! Really shows the beauty of this subject!
In the last exercise I got that the sum is π³/32, did anybody got the value too?

leeholzer
Автор

At 2:45 I don't get how the residues of 1/(z^2 tan z) are determined: Prof Borcherds says that at -2pi and 2pi the residues are 1/(2pi)^2, and at -pi or +pi they are 1/pi^2. On the other hand, later at 9:00, he shows from the expansion of 1/(z^2 tan z) = (1/z^2) cot(z) = (1/z^2) (1/z - z/3 ...) = 1/z^3 - 1/3z ..., that the residue is -1/3, which makes sense. But there seems to be a discrepancy.

reinerwilhelms-tricarico
Автор

Was this video re-uploaded? I'm sure I saw it about a week ago.

peterboneg
Автор

In the description, what's the solution to the exercise?

VaslavTchitcherine
Автор

This is an introductory course ... I will show a straightforward application of this difficult concept just introduced ... proceeds to a problem where subtle artifices are absolutely required ... same issue in video 12

a gift horse and all that ... but smh

dacianbonta